A 20 kg block is initially at rest. A 75 N force is required to set the block in motion. After the motion a force of 60 N is applied to keep the block moving with constant speed. The coefficient of static friction is
0.6
0.52
0.44
0.35
The correct Answer is:d
To find the coefficient of static friction (μ_s) for the block, we can follow these steps:
Step 1: Understand the forces acting on the block
When the block is at rest, the force of static friction (f_s) opposes the applied force. The block will start moving when the applied force exceeds the maximum static friction force.
Step 2: Write the equation for static friction
The maximum static friction force can be expressed as:
fs=μs⋅N
where:
- fs is the force of static friction,
- μs is the coefficient of static friction,
- N is the normal force.
Step 3: Determine the normal force (N)
For a block resting on a horizontal surface, the normal force (N) is equal to the weight of the block:
N=m⋅g
where:
- m=20kg (mass of the block),
- g=10m/s2 (acceleration due to gravity).
Calculating the normal force:
N=20kg⋅10m/s2=200N
Step 4: Set up the equation using the given force
According to the problem, a force of 75 N is required to set the block in motion. This means:
fs=75N
Step 5: Substitute into the static friction equation
Now we can substitute the values into the static friction equation:
75N=μs⋅200N
Step 6: Solve for the coefficient of static friction (μ_s)
Rearranging the equation to solve for μ_s gives:
μs=75N200N
μs=0.375
Final Answer
The coefficient of static friction μs is 0.375.
---
When the block is at rest, the force of static friction (f_s) opposes the applied force. The block will start moving when the applied force exceeds the maximum static friction force.
The maximum static friction force can be expressed as:
fs=μs⋅N
where:
- fs is the force of static friction,
- μs is the coefficient of static friction,
- N is the normal force.
For a block resting on a horizontal surface, the normal force (N) is equal to the weight of the block:
N=m⋅g
where:
- m=20kg (mass of the block),
- g=10m/s2 (acceleration due to gravity).
N=20kg⋅10m/s2=200N
According to the problem, a force of 75 N is required to set the block in motion. This means:
fs=75N
Now we can substitute the values into the static friction equation:
75N=μs⋅200N
Rearranging the equation to solve for μ_s gives:
μs=75N200N
μs=0.375
The coefficient of static friction μs is 0.375.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - MOTION IN FLUIDS
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - UNIFORM CIRCULAR MOTION
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics ( WORK POWER ENERGY )
35 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
A20 kg block is initally at rest. A 75 N force is required to set the block in motion. After the motion starts, a force of 60 N is required to keep the block moving with constant speed. The coefficient of static friction is
View SolutionA body of mass 25 kg is at rest on a horizontal surface. Minimum horizontal force required to just start motion is 73.5N and a force of 49N is needed to keep the body moving with a constant velocity. What is the coefficient of (i) static friction and (ii) kinetic friction ?
View SolutionA block of mass 5kg rests on a rough horizontal surface. It is found that a force of 10N is required to make the block just move However , once the motion begains, a force of only 8N is enough to maintain the motion find the coefficient of kinetic and static friction between the block and the horizontal surface

View SolutionA block of mass 5kg rests on a rough horizontal surface. It is found that a force of 10N is required to make the block just move However , once the motion begains, a force of only 8N is enough to maintain the motion find the coefficient of kinetic and static friction between the block and the horizontal surface

View SolutionA 5 kg block is placed on the top of a 15 kg block as shown in figure . A horizontal force of 60 N is applied to the 15 kg block and the 5kg block is tied to the wall . The coefficient of friction between moving surfaces is 0.2

(a) Draw the free body diagram for each block w.r.t ground and identify the action reaction forces between two blocks .
(b) Determine the tension in the string and magnitude of the acceleration of the 15 kg block .
View SolutionA force of 49 N just able to move a block of wood weighing 10 kg on a rough horizontal surface , then coefficient of friction is
View SolutionA horizontal force of 20 N is applied to a block of mass 4 kg resting on a rough horizontal table. If the block does not move on the table, how much frictional force the table is applying on the block. What can be said about the coefficient of static friction between the block and the table? Takeg=10ms2.
View Solution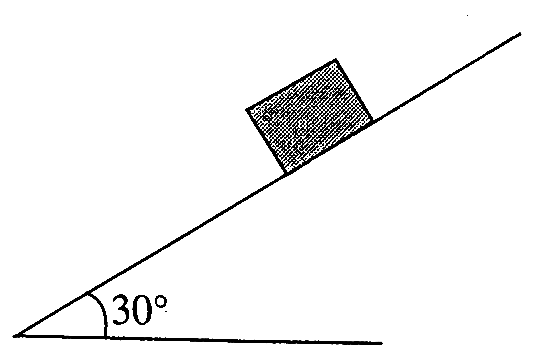
Figure shows a block kept on a rough inclined plane. The maximum external force down the incline for which the block remains at rest is 1 N while the maximum external force up the incline for which the block is at rest is 7 N. The coefficient of static friction μ is:
View SolutionA block weighing 20N rests on a horizontal surface . The coefficient of static friction between the block and surface is 0.4 and the coeffiecient of kinetic frictioni 0.20
a.How much is the friction force excerted on the block?
b. How much will the friction force be if a horizontal force of 5N is exerted on the block?
c. What is the minimum force that will start the block in motion ?
d. What is the minimum force that will keep the block in motion once is has been started ?
e. If the horizontal force is 10N what is the friction force?
View SolutionA block is lying static on the floor. The maximum value of static fictional force on the block is 10 N. If a horizontal force of 6 N is applied to the block,what will be the frictional force on the block?
View Solution
