Water is falling on the blades of turbine at a rate of 100 kg/s from a certain spring. IF the height of the spring be 100 m, the power transferred to the turbine will be
100 W
1 kW
10 kW
100 kW
The correct Answer is:d
To solve the problem of calculating the power transferred to the turbine by the falling water, we can follow these steps:
Step 1: Understand the formula for power
The power (P) transferred to the turbine can be calculated using the formula:
P=Wt
where W is the work done and t is the time.
Step 2: Express work done in terms of force and displacement
The work done (W) can also be expressed as:
W=F⋅d
where F is the force and d is the displacement. In this case, the displacement is the height (h) from which the water is falling.
Step 3: Determine the force acting on the water
The force (F) can be calculated using the formula:
F=m⋅g
where m is the mass of water and g is the acceleration due to gravity. Given that the mass flow rate is mt=100kg/s and taking g=10m/s2, we can substitute this into the force equation.
Step 4: Substitute values into the equations
Now we can express the power in terms of the mass flow rate:
P=m⋅g⋅ht
Since mt=100kg/s, we can rewrite the equation as:
P=(100kg/s)⋅g⋅h
Step 5: Plug in the values
Substituting the known values:
- g=10m/s2
- h=100m
We have:
P=100kg/s⋅10m/s2⋅100m
Step 6: Calculate the power
Now, performing the multiplication:
P=100⋅10⋅100=100000W
Step 7: Convert to kilowatts
Since 1kW=1000W:
P=100000W1000=100kW
Final Answer
The power transferred to the turbine is 100 kW.
---
The power (P) transferred to the turbine can be calculated using the formula:
P=Wt
where W is the work done and t is the time.
The work done (W) can also be expressed as:
W=F⋅d
where F is the force and d is the displacement. In this case, the displacement is the height (h) from which the water is falling.
The force (F) can be calculated using the formula:
F=m⋅g
where m is the mass of water and g is the acceleration due to gravity. Given that the mass flow rate is mt=100kg/s and taking g=10m/s2, we can substitute this into the force equation.
Now we can express the power in terms of the mass flow rate:
P=m⋅g⋅ht
Since mt=100kg/s, we can rewrite the equation as:
P=(100kg/s)⋅g⋅h
Substituting the known values:
- g=10m/s2
- h=100m
P=100kg/s⋅10m/s2⋅100m
Now, performing the multiplication:
P=100⋅10⋅100=100000W
Since 1kW=1000W:
P=100000W1000=100kW
The power transferred to the turbine is 100 kW.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - FRICTION
22 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - MOTION IN FLUIDS
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics ( Laws of motion )
36 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
From a waterfall, water is falling down at the rate of 100kg / s on the blades of turbine. If the height of the fall is 100 m , then the power delivered to the turbine is approximately equal to
View SolutionIn a dam,water falls at a rate of 1000 kg s−1 from a height of 100 m .
(b)Assuming that 60% of the energy of the falling water is converted to electrical energy,Calculate the power generated (Take g=9.8 m s−2)
View Solution
Knowledge Check
A crane lifts a mass of 100 kg to a height of 10 m in 20 s. The power of the crane is (Take g=10ms−2)
A100 WB200 WC250 WD500 W
Water flows through a tunnel from the reservior of a dam towards the turbine installed in its power plant . The power plant is situated h metre below the reservoir . If the ratio of the cross sectional areas of the tunnel at the resercoir and power station end is η , find the speed of the water entering into the turbine .
View SolutionWater falls from a height of 60m at the rate 15kg/s to operate a turbine. The losses due to frictional forces are 10% of energy . How much power is generated by the turbine? (g=10 m//s^(2)).
View SolutionWater falls from a height of 60m at the rate 15kg/s to operate a turbine. The losses due to frictional forces are 10% of energy . How much power is generated to by the turbine? (g=10 m//s^(2)).
View SolutionA block of 2 kg is suspended from the ceiling through a massless spring of spring constant k=100 N/m. What is the elongation of the spring? If another 1 kg is added to the block, what would be the further elongation?
View SolutionA 16kg block moving on a frictionless horizontal surface with a velocity of 4m/s compresses an ideal spring and comes to rest. If the force constant of the spring be 100N/m, then how much is the spring commpressed ?
View SolutionA block of mass √2 kg is released from the top of an inclined smooth surface as shown in fighre. If spring constant of spring is 100 N/m and block comes to rest after compressing the spring by 1 m, then the distance travelled by block befor it comes to rest is
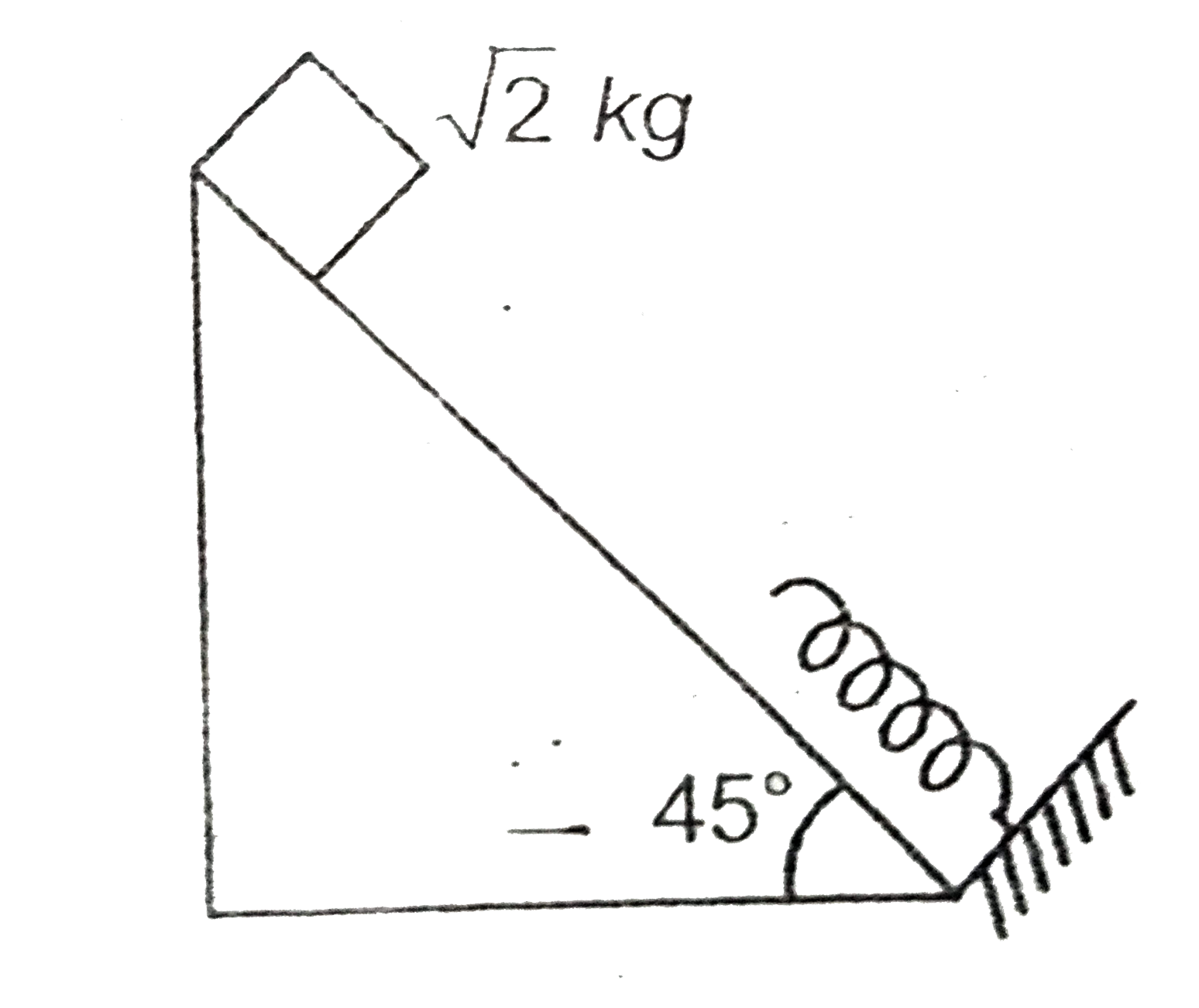
View SolutionA block of mass √2 kg is released from the top of an inclined smooth surface as shown in fighre. If spring constant of spring is 100 N/m and block comes to rest after compressing the spring by 1 m, then the distance travelled by block befor it comes to rest is

View Solution
