A particle of mass 0.1 kg is subjected to a force which is directly proportional to the distance x. the work done by the force is directly proportional to
x
The correct Answer is:d
To solve the problem, we need to analyze the relationship between the force acting on the particle and the work done by that force.
1. Understanding the Force Relationship:
The problem states that the force F is directly proportional to the distance x. This can be expressed mathematically as:
F=kx
where k is a constant of proportionality.
2. Work Done by the Force:
The work done W by a force when it moves an object through a distance S is given by the formula:
W=F⋅S
In this case, since the force is proportional to the distance x, we can substitute F into the work formula:
W=(kx)⋅S
3. Relating Work Done to Distance:
If we consider the displacement S to be the same as the distance x (i.e., the particle moves from 0 to x), we can rewrite the work done as:
W=kx⋅x=kx2
4. Conclusion on Proportionality:
From the equation W=kx2, we can see that the work done W is directly proportional to the square of the distance x. Therefore, we can conclude that:
W∝x2
Final Answer:
The work done by the force is directly proportional to x2.
---
The problem states that the force F is directly proportional to the distance x. This can be expressed mathematically as:
F=kx
where k is a constant of proportionality.
The work done W by a force when it moves an object through a distance S is given by the formula:
W=F⋅S
In this case, since the force is proportional to the distance x, we can substitute F into the work formula:
W=(kx)⋅S
If we consider the displacement S to be the same as the distance x (i.e., the particle moves from 0 to x), we can rewrite the work done as:
W=kx⋅x=kx2
From the equation W=kx2, we can see that the work done W is directly proportional to the square of the distance x. Therefore, we can conclude that:
W∝x2
The work done by the force is directly proportional to x2.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - FRICTION
22 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - MOTION IN FLUIDS
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics ( Laws of motion )
36 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
Knowledge Check
Stopping distance of a moving vehicle is directly proportional to
Asquare of the initial velocityBsquare of the initial accelerationCthe initial velocityDthe initial accelerationStopping distance of a moving vehicle is directly proportional to
ASquare of initial velocityBSquare of initial accelerationCThe initial velocityDThe initial acceleration
The radius of a nucleus of a mass number A is directly proportional to.
View Solution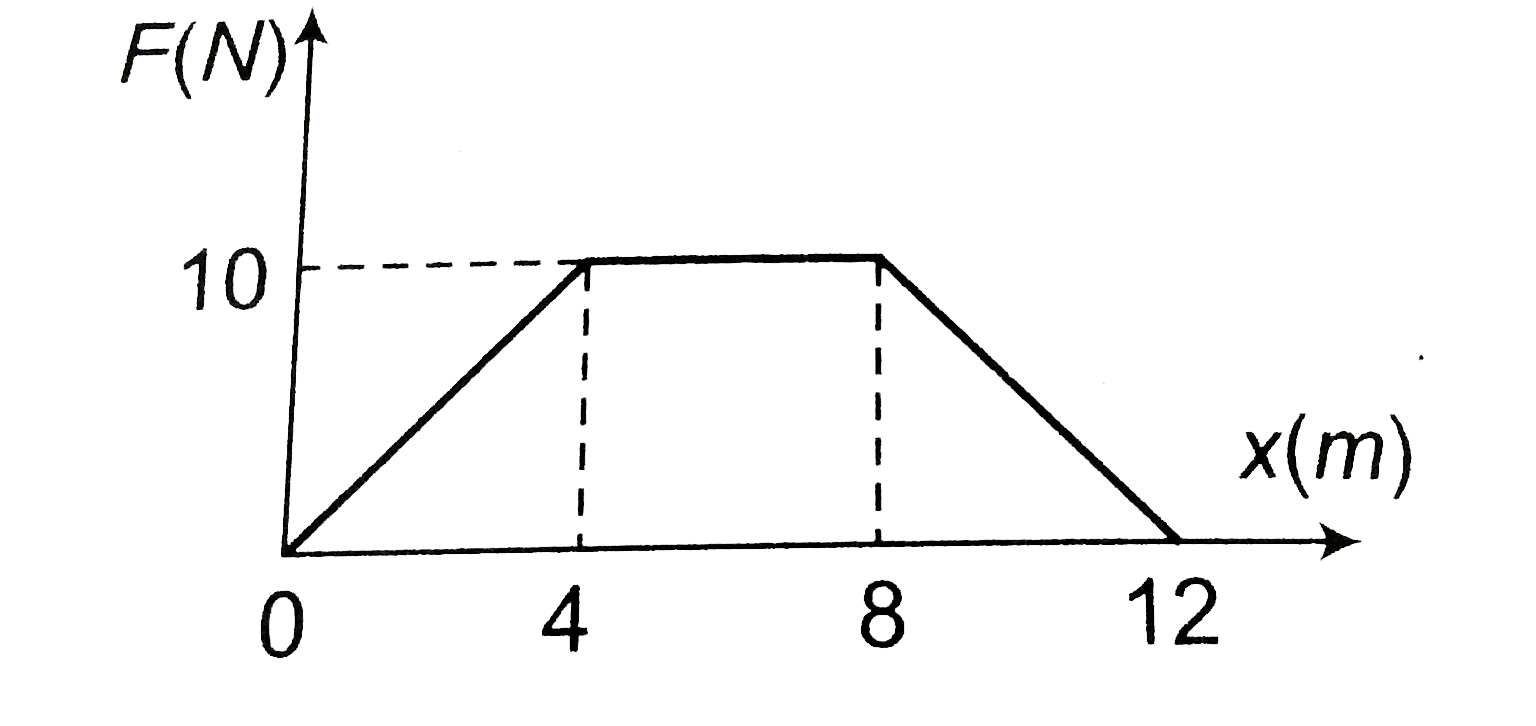
A particle of mass 0.1 kg is subjected to a force which varies with distance as shown in figure. If it starts its journey from rest at x=0, its velocity at x=12m is
View Solution
A particle of mass 0.1 kg is subjected to a force which varies with distance as shown in figure. If it starts its journey from rest at x=0, its velocity at x=12m is
View SolutionA particle of mass 0.1 kg is subjected to a force F which varies with distance x as shown. If it starts its journey from rest at x = 0, then its speed at X = 12 m is
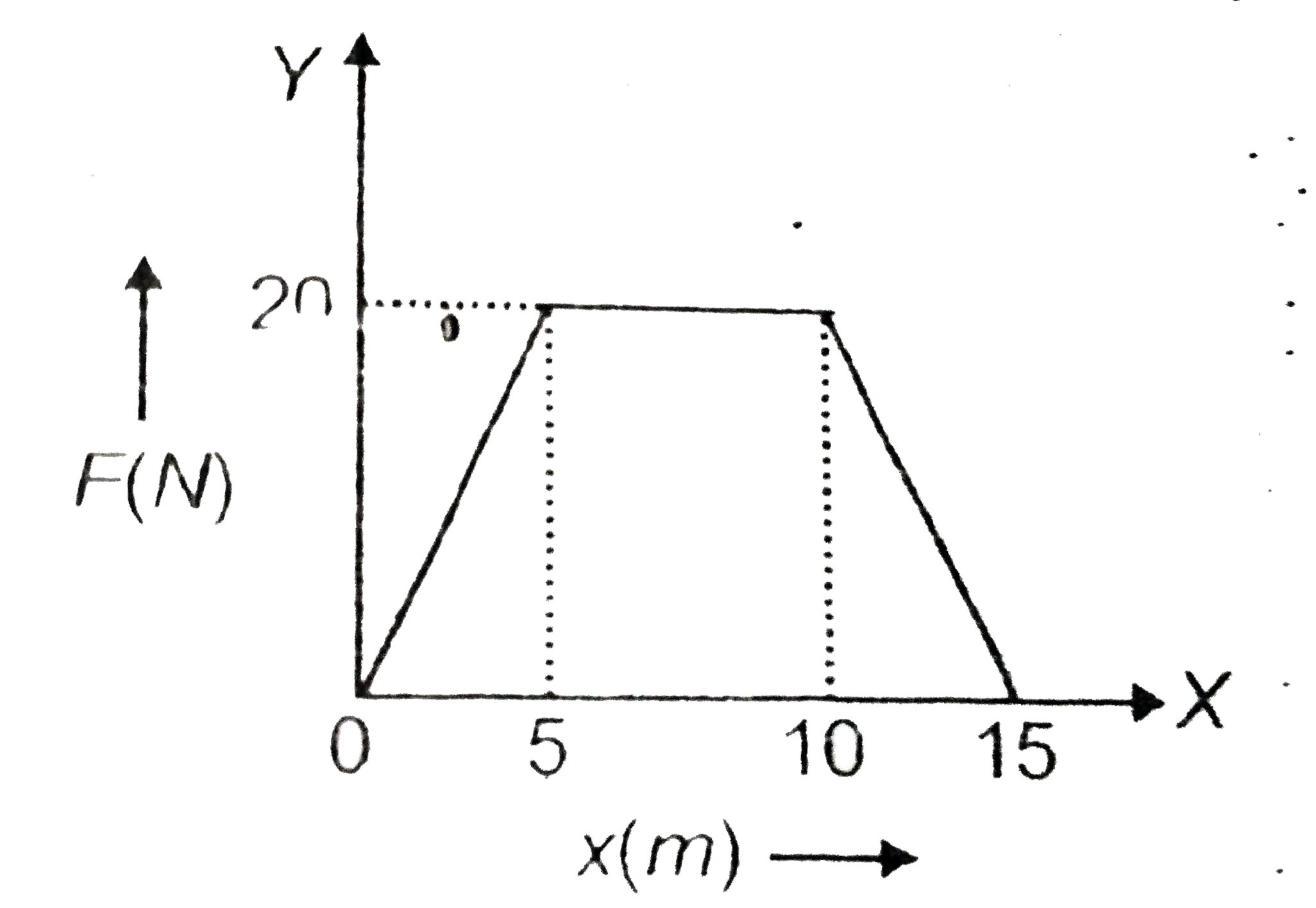
View SolutionA particle of mass 1 kg is subjected to a force which varies with distance as shown. If it starts it s journey from rest at x=0. then its velocity at x=15 m is
View SolutionThe damping force on an oscillator is directly proportional to the velocity. The units of the constant to proportionality are
View Solution
