The distance travelled by a body falling from reset in the frist, second and third seconds are in the ratio
1:2:3
1:3:5
1:4:9
none of the above
The correct Answer is:b
To find the distances traveled by a body falling from rest in the first, second, and third seconds, we can use the equations of motion under uniform acceleration due to gravity.
1. Understanding the Problem:
- A body falls from rest, meaning its initial velocity u=0.
- We need to calculate the distances covered in the first second (s1), the second second (s2), and the third second (s3).
2. Distance in the First Second (s1):
- The formula for distance traveled under constant acceleration is given by:
s=ut+12at2
- For the first second (t=1 s):
s1=0⋅1+12g(12)=12g
3. Distance in the First Two Seconds (s2):
- For the total distance traveled in the first two seconds (t=2):
stotal=0⋅2+12g(22)=12g⋅4=2g
- To find s2 (distance traveled during the second second), we subtract the distance traveled in the first second from the total distance in two seconds:
s2=stotal−s1=2g−12g=4g2−12g=3g2
4. Distance in the First Three Seconds (s3):
- For the total distance traveled in the first three seconds (t=3):
stotal=0⋅3+12g(32)=12g⋅9=9g2
- To find s3 (distance traveled during the third second), we subtract the distance traveled in the first two seconds from the total distance in three seconds:
s3=stotal−stotal(2s)=9g2−2g=9g2−4g2=5g2
5. Finding the Ratios:
- Now we have:
s1=12g,s2=32g,s3=52g
- The ratio s1:s2:s3 can be expressed as:
1/2g1/2g:3/2g1/2g:5/2g1/2g=1:3:5
Final Answer:
The distances traveled by the body in the first, second, and third seconds are in the ratio 1:3:5.
---
- A body falls from rest, meaning its initial velocity u=0.
- We need to calculate the distances covered in the first second (s1), the second second (s2), and the third second (s3).
- The formula for distance traveled under constant acceleration is given by:
s=ut+12at2
- For the first second (t=1 s):
s1=0⋅1+12g(12)=12g
- For the total distance traveled in the first two seconds (t=2):
stotal=0⋅2+12g(22)=12g⋅4=2g
- To find s2 (distance traveled during the second second), we subtract the distance traveled in the first second from the total distance in two seconds:
s2=stotal−s1=2g−12g=4g2−12g=3g2
- For the total distance traveled in the first three seconds (t=3):
stotal=0⋅3+12g(32)=12g⋅9=9g2
- To find s3 (distance traveled during the third second), we subtract the distance traveled in the first two seconds from the total distance in three seconds:
s3=stotal−stotal(2s)=9g2−2g=9g2−4g2=5g2
- Now we have:
s1=12g,s2=32g,s3=52g
- The ratio s1:s2:s3 can be expressed as:
1/2g1/2g:3/2g1/2g:5/2g1/2g=1:3:5
The distances traveled by the body in the first, second, and third seconds are in the ratio 1:3:5.
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:Dynamics (PROJECTILE MOTION)
Explore 31 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:Dynamics ( Laws of motion )
Explore 36 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:VECTORS AND SCALARS [Selected from Previoius Years Engg. & Med. & IIT Exam Qns]
Explore 35 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
Knowledge Check
The distances covered by a freely falling body in its first, second, third, ……, bth seconds of its motion a)forms an arithmetic progression b) forms a geometric progression c) do not form any well defined series d) form a series corresponding to the difference of square root of the successive natural numbers.
Aforms an arithematic progressionBforms a geometric progressionCdo not form any well defined seriesDform a series corresponding to the difference of square root of the successive natural numbers.
The distance travelled by a falling body in the last second of its motion, to that in the last but one second is 7: 5, the velocity with which body strikes the ground is
View SolutionIf the distance travelled by a freely falling body in the last second of its journey is equal to the distance travelled in the first 2s, the time of descent of the body is
View SolutionIf x=at+bt2 , where x is the distance travelled by the body in kilometer while t is the time in seconds , then find the units of b.
View SolutionFind the ratio of the distance moved by a free-falling body from rest in fourth and fifth seconds of its journey.
View SolutionThe distance moved by a freely falling body (startibg from rest) during st,2nd,3nd,……nth second of its motion are propotional to .
View SolutionThe distance travelled by a body during last second of its total flight is d when the body is projected vertically up with certain velocity. If the velocity of projection is doubled, the distance travelled by the body during last second of its total flight is
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
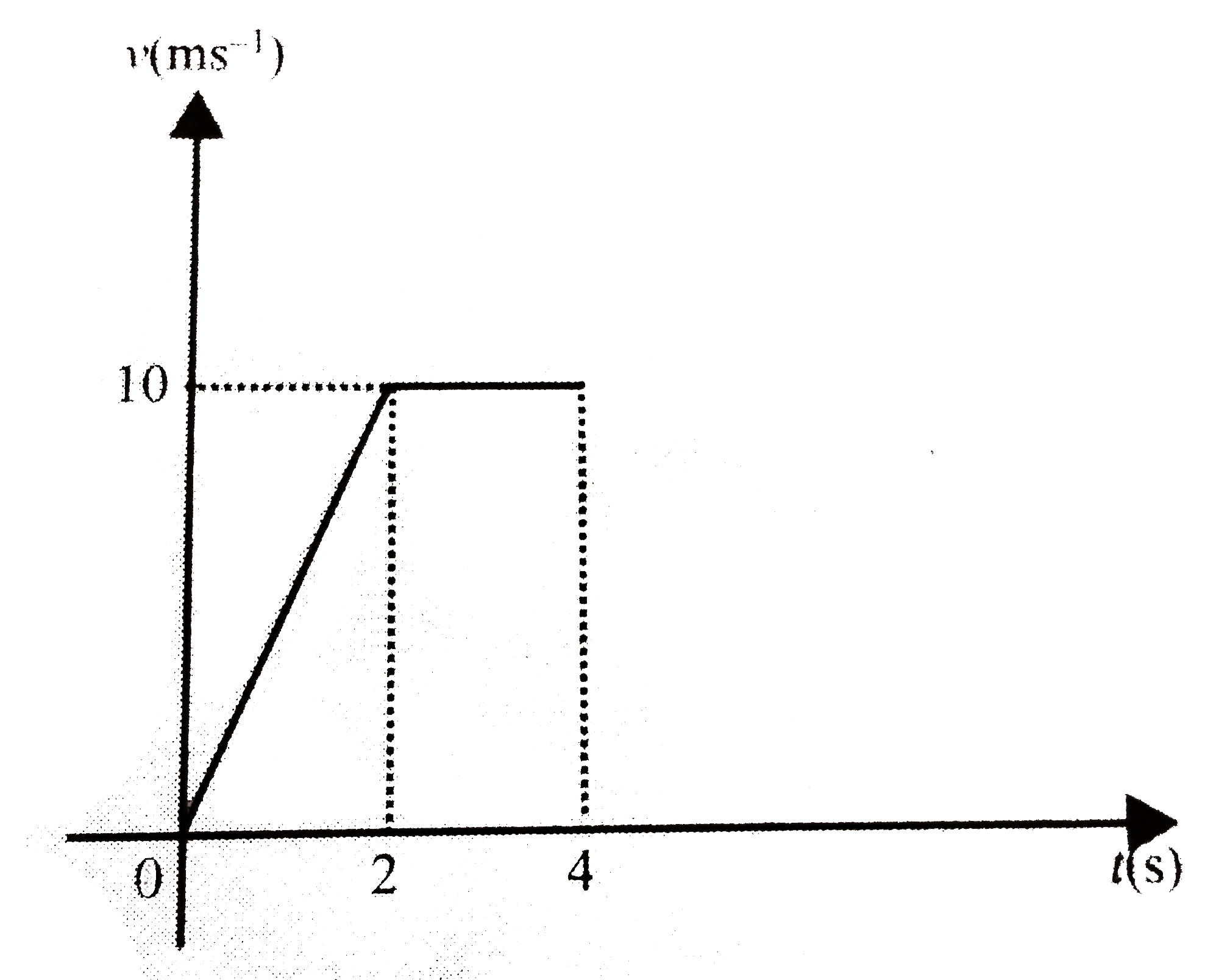
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View Solution