A man runs along the straight road for half the distance with velocity v1 and the remaining half distance with velocity v2. Then the average velocity is given by
v1v2
v22/v21
(v1+v2)/2
2v1v2/(v1+v2)
The correct Answer is:d
To find the average velocity of a man running half the distance with velocity v1 and the other half with velocity v2, we can follow these steps:
1. Define the Total Distance:
Let the total distance be 2x. This means the man runs half the distance x with velocity v1 and the other half x with velocity v2.
2. Calculate Time for Each Half:
- For the first half (distance x with velocity v1):
t1=xv1
- For the second half (distance x with velocity v2):
t2=xv2
3. Calculate Total Time:
The total time T taken to cover the entire distance 2x is the sum of the times for both halves:
T=t1+t2=xv1+xv2
4. Combine the Times:
To combine the two fractions, we can find a common denominator:
T=x(1v1+1v2)=x(v2+v1v1v2)
5. Calculate Average Velocity:
The average velocity Vavg is defined as the total distance divided by the total time:
Vavg=Total DistanceTotal Time=2xT
Substituting the expression for T:
Vavg=2xx(v1+v2v1v2)
6. Simplify the Expression:
The x in the numerator and denominator cancels out:
Vavg=2v1+v2v1v2=2v1v2v1+v2
Final Answer
Thus, the average velocity of the man is:
Vavg=2v1v2v1+v2
Let the total distance be 2x. This means the man runs half the distance x with velocity v1 and the other half x with velocity v2.
- For the first half (distance x with velocity v1):
t1=xv1
- For the second half (distance x with velocity v2):
t2=xv2
The total time T taken to cover the entire distance 2x is the sum of the times for both halves:
T=t1+t2=xv1+xv2
To combine the two fractions, we can find a common denominator:
T=x(1v1+1v2)=x(v2+v1v1v2)
The average velocity Vavg is defined as the total distance divided by the total time:
Vavg=Total DistanceTotal Time=2xT
Substituting the expression for T:
Vavg=2xx(v1+v2v1v2)
The x in the numerator and denominator cancels out:
Vavg=2v1+v2v1v2=2v1v2v1+v2
Thus, the average velocity of the man is:
Vavg=2v1v2v1+v2
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:Dynamics (PROJECTILE MOTION)
Explore 31 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:Dynamics ( Laws of motion )
Explore 36 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:VECTORS AND SCALARS [Selected from Previoius Years Engg. & Med. & IIT Exam Qns]
Explore 35 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
A person travels along a straight road for half the distance with velocity V1 and the remaining half distance with velocity V2 the average velocity is given by
View SolutionA person travels along a straight road for the half time with a velocity V1 and the next half time with a velocity V2. The mean velocity V of the man is
View Solution
Knowledge Check
A person travels along a straight road for the first half with a velocity v1 and the second half time with a velocity v2. Then the mean velocity →v is given by
A¯v=v1+v22B2v=1v1+1v2C¯v=√v1v2D¯v=√v2v1
A body covers one-third of the distance with a velocity v1 the second one-third of the distance with a velocity v2, and the last one-third of the distance with a velocity v3. The average velocity is:-
View SolutionA car completes the first half of its journey with a velocity V1 and the remaining half with a velocity V2. Then the average velocity of the car for the whole journey is
View SolutionA man traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity v1. For half the time and with velocity v2 for the other half of the time . Find the average speed of the man over the whole time of motion.
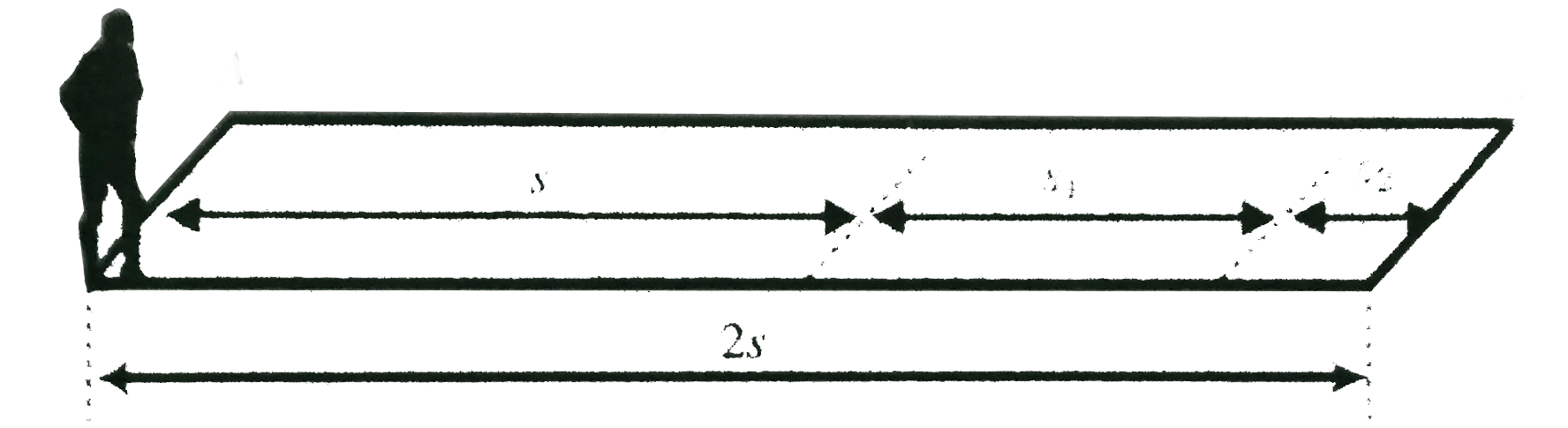 .
.
View SolutionA body travelling along a straight line , one thired of the total distance with a velocity 4ms−1. The remaining part of the distance was covered with a velocity 2ms−1 for half the time and with velocity 6ms−1 for the other half of time . What is the mean velocity averaged over te whle time of motin ?
View SolutionA person travelling on a straight line moves with a uniform velocity v1 for a distance x and with a uniform velocity v2 for the next equal distance. The average velocity v is given by
View SolutionA body travelling along a straight line traversed one third of the total distance with a velocity 4m/s. The remaining part of the distance was covered with a velocity 1 m/s for half the time and with velocity 3 m/s for the other half of time. The mean velocity averaged over the whole time of motion is :
View SolutionA particle traversed half of the distance with a velocity of V0.The remaining parts of the distance was covered with velocity V1, for half of the time and with V2 for other half of the time .Find the mean velocity of the particle averahed and the whole time of motion .
View Solution