If a capillary tube in dipped in a state of weightlessness how will the rise of water in it be different to that observed in nomal conditions?
To solve the question regarding the behavior of water in a capillary tube under weightlessness, we can follow these steps:
Step 1: Understand Capillary Action
Capillary action is the ability of a liquid to flow in narrow spaces without the assistance of external forces. It occurs due to the combination of cohesive forces (between liquid molecules) and adhesive forces (between liquid molecules and the surface of the tube).
Step 2: Identify the Role of Gravity
In normal conditions, the rise of water in a capillary tube is influenced by gravity (g). The height (h) to which the liquid rises can be calculated using the formula:
h=2Tcos(θ)Rρg
where:
- T is the surface tension of the liquid,
- θ is the contact angle,
- R is the radius of the capillary tube,
- ρ is the density of the liquid,
- g is the acceleration due to gravity.
Step 3: Consider the Effect of Weightlessness
In a state of weightlessness, the effective gravitational acceleration (g) becomes zero. This means that the downward force acting on the liquid column is absent.
Step 4: Analyze the Formula Under Weightlessness
Substituting g=0 into the formula for height:
h=2Tcos(θ)Rρ⋅0
This results in a division by zero, indicating that the height h approaches infinity.
Step 5: Conclusion
Since there is no downward force acting on the water due to the absence of gravity, the water will rise to the top of the capillary tube, effectively filling it completely.
Final Answer
In a state of weightlessness, the rise of water in a capillary tube will be different from normal conditions in that the water will rise to the top of the tube, potentially filling it completely, as there is no gravitational force acting downward.
---
Capillary action is the ability of a liquid to flow in narrow spaces without the assistance of external forces. It occurs due to the combination of cohesive forces (between liquid molecules) and adhesive forces (between liquid molecules and the surface of the tube).
In normal conditions, the rise of water in a capillary tube is influenced by gravity (g). The height (h) to which the liquid rises can be calculated using the formula:
h=2Tcos(θ)Rρg
where:
- T is the surface tension of the liquid,
- θ is the contact angle,
- R is the radius of the capillary tube,
- ρ is the density of the liquid,
- g is the acceleration due to gravity.
In a state of weightlessness, the effective gravitational acceleration (g) becomes zero. This means that the downward force acting on the liquid column is absent.
Substituting g=0 into the formula for height:
h=2Tcos(θ)Rρ⋅0
This results in a division by zero, indicating that the height h approaches infinity.
Since there is no downward force acting on the water due to the absence of gravity, the water will rise to the top of the capillary tube, effectively filling it completely.
In a state of weightlessness, the rise of water in a capillary tube will be different from normal conditions in that the water will rise to the top of the tube, potentially filling it completely, as there is no gravitational force acting downward.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 2(SURFACE TENSIONS) SELECTED PROBLEMS( FROM THE DEFINITION OF SURFACE TENSION)
27 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 2(SURFACE TENSIONS) FROM CAPILLARY
15 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 2(SURFACE TENSIONS)SHORT ANWER QUESTIONS
23 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
- SAMPLE QUESTION PAPER - 01 BOOK - ICSECHAPTER - SAMPLE QUESTION PAPER - 01 EXERCISE - SECTION - D
12 Videos
Similar Questions
Knowledge Check
When two capillary tubes of different diameters are dipped vertically, the rise of the liquids is
Asame in both the tubesBmore in tube of larger diameterCless in tube of smaller diameterDmore in the tube of smaller diameter
If the capillary tube is dipped in water is of insufficient length (l<h),will water overflow?
View SolutionIf a capillary tube is dipped and the liquid levels inside and outside the tube are same. Then the angle of contact is
View SolutionFigure shows a capillary tube of radius r dipped into The atmospheric pressure is P0 and the capillary rise of water is h. s is the surface tension for water-glass.
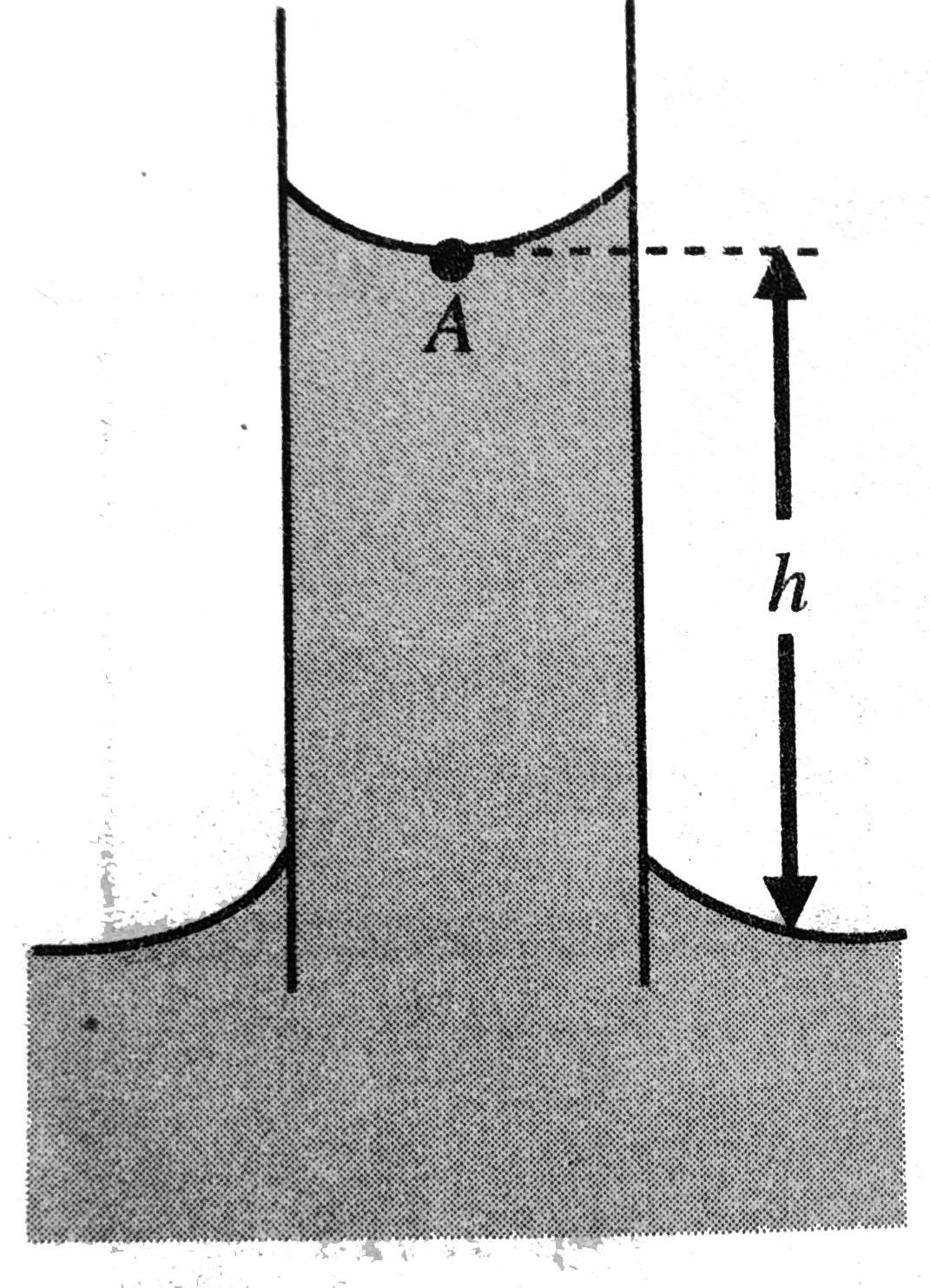
Initially, h=10cm. If the capillary tube is now incline at 45∘, the length of water rising in the tube will be
View SolutionA capillary tube is dipped in water to a depth and the water rises to a height h(<l) in the capillary tube. The lower end of the tube is closed in water by putting a lower over it. The tube is now taken out and the thumb is removed from the lower end and it kept open. The length of liquid column in the tube will be
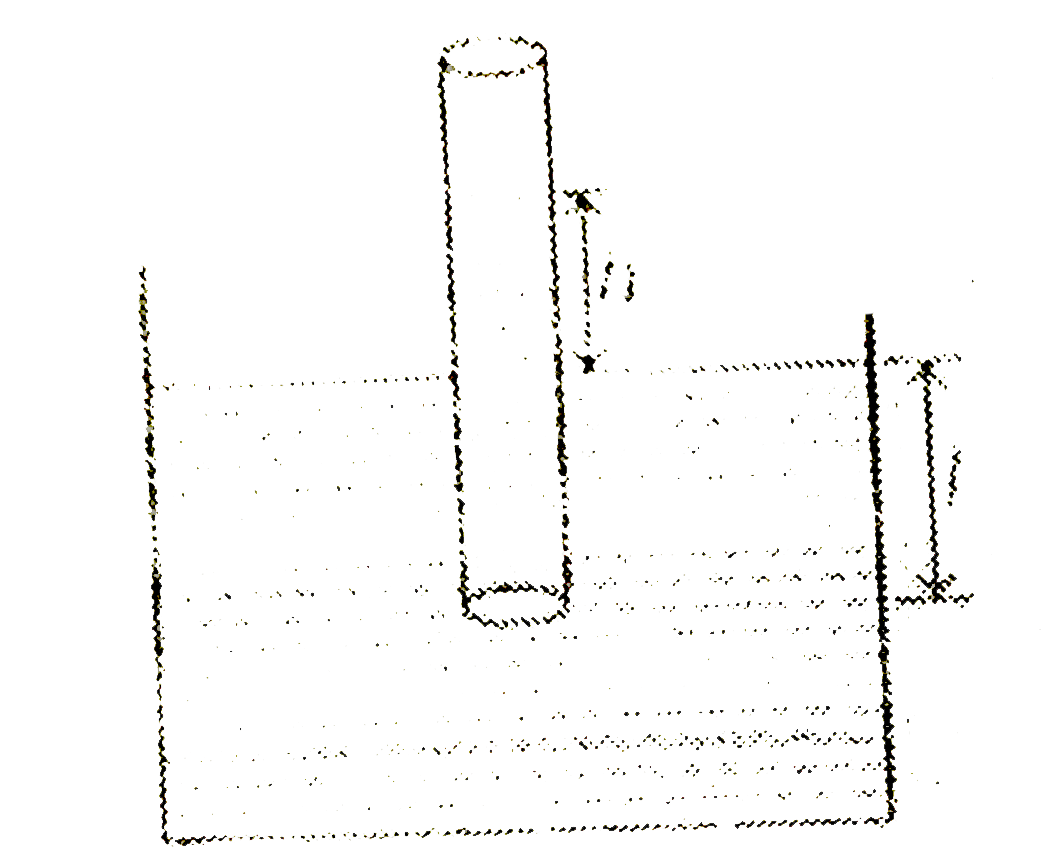
View SolutionWhen a long capillary tube is immersed in water, mass M of water rises in capillary. What mass of water will rise in another long capillary of half the radius and made of same material?
View SolutionFigure shows a capillary tube C dipped in a liquid that wets it. The liquid rises to a point A. If we blow air through the horizontal tube H, what will happen to the liquid column in the capillary tube?
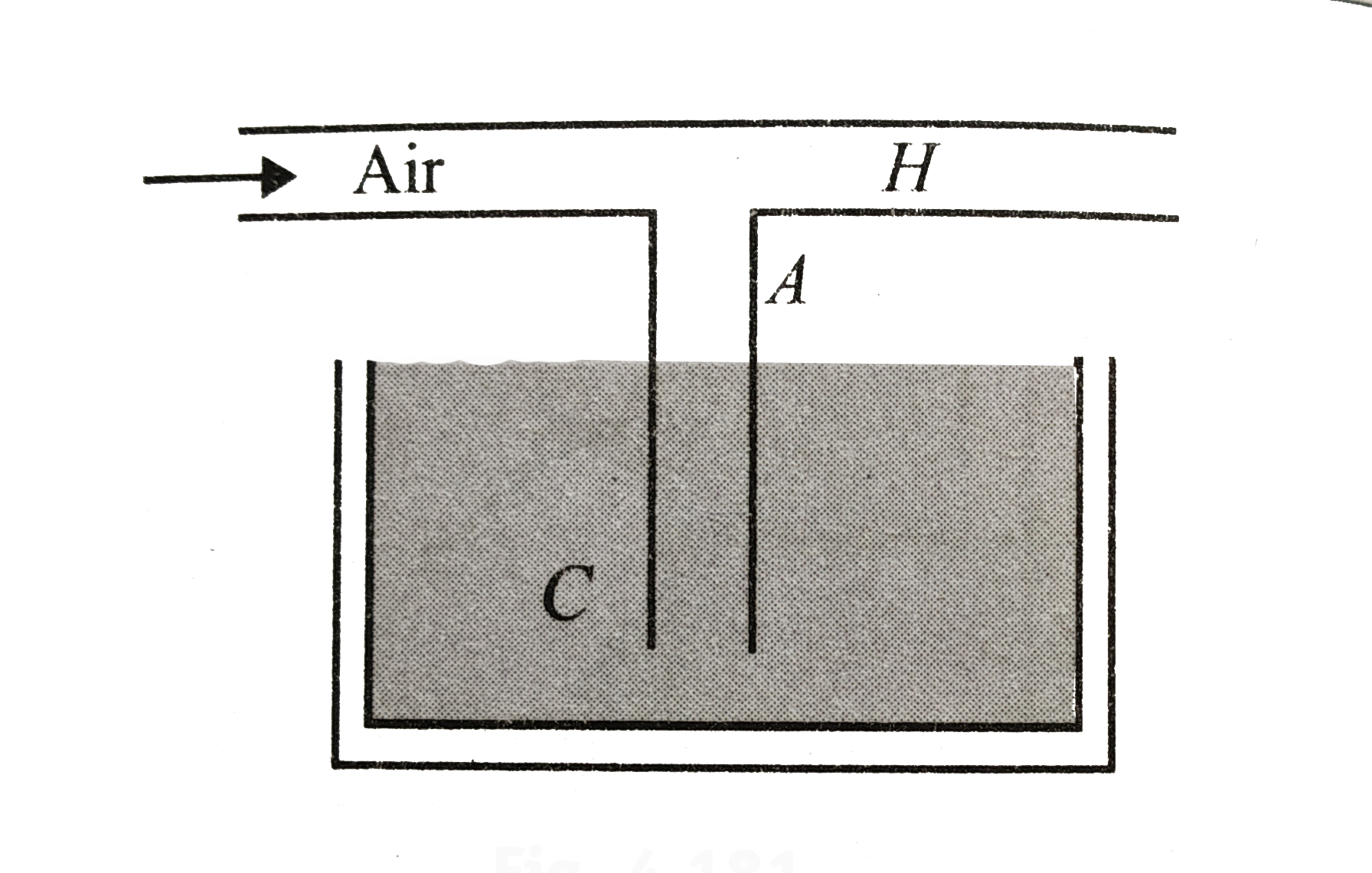
View SolutionA capillary tube is dipped in water vertically. It is long enough for the water to rise to the maximum height h in the tube. The length of portion immersed in water is I<h. The lower end of the tube is closed and then the tube is taken out and opened again. Will all the water flow out of tube? Explain.
View Solution
