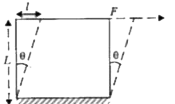
A square aluminium of side 50cm and thickness 5cm is subjected to a shearing force of magnitude 104N. The lower edge is revetted to the floor. How much is the upper edge displaced if shear modulus of aluminium is 2.5×1010Nm−2 ?
The correct Answer is:8×10−6m
To solve the problem step by step, we will follow the outlined procedure to find the displacement of the upper edge of the aluminum slab when subjected to a shearing force.
Step 1: Identify the given values
- Side of the square aluminum slab (L) = 50 cm = 0.5 m
- Thickness of the slab (t) = 5 cm = 0.05 m
- Shearing force (F) = 104 N
- Shear modulus of aluminum (G) = 2.5×1010 N/m²
Step 2: Calculate the area subjected to shearing stress
The area (A) on which the shearing force acts can be calculated using the formula:
A=L×t
Substituting the values:
A=0.5m×0.05m=0.025m2
Step 3: Calculate the shearing stress
Shearing stress (τ) is given by:
τ=FA
Substituting the values:
τ=104N0.025m2=4×106N/m2
Step 4: Relate shear modulus, shear stress, and shear strain
The shear modulus (G) is defined as:
G=τshear strain
Where shear strain is given by:
shear strain=ΔLL
Thus, we can write:
G=τΔLL
Rearranging gives:
ΔL=τ⋅LG
Step 5: Substitute the values to find the displacement (ΔL)
Now substituting the values we have:
ΔL=(4×106N/m2)⋅(0.5m)2.5×1010N/m2
Calculating this gives:
ΔL=2×1062.5×1010=8×10−6m
Final Answer
The upper edge of the aluminum slab is displaced by ΔL=8×10−6m or 8μm.
---
- Side of the square aluminum slab (L) = 50 cm = 0.5 m
- Thickness of the slab (t) = 5 cm = 0.05 m
- Shearing force (F) = 104 N
- Shear modulus of aluminum (G) = 2.5×1010 N/m²
The area (A) on which the shearing force acts can be calculated using the formula:
A=L×t
Substituting the values:
A=0.5m×0.05m=0.025m2
Shearing stress (τ) is given by:
τ=FA
Substituting the values:
τ=104N0.025m2=4×106N/m2
The shear modulus (G) is defined as:
G=τshear strain
Where shear strain is given by:
shear strain=ΔLL
Thus, we can write:
G=τΔLL
Rearranging gives:
ΔL=τ⋅LG
Now substituting the values we have:
ΔL=(4×106N/m2)⋅(0.5m)2.5×1010N/m2
Calculating this gives:
ΔL=2×1062.5×1010=8×10−6m
The upper edge of the aluminum slab is displaced by ΔL=8×10−6m or 8μm.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 1 (ELASTICITY) FROM WORK DONE IN STRETCHING A WIRE
4 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 2(SURFACE TENSIONS)SHORT ANSWER QUESTIONS WITH ANSWERS
14 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 1 (ELASTICITY) FROM BULK MODULUS
8 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
- SAMPLE QUESTION PAPER - 01 BOOK - ICSECHAPTER - SAMPLE QUESTION PAPER - 01 EXERCISE - SECTION - D
12 Videos
Similar Questions
A square lead slab of side 50cm and thickness 5.0cm is subjected to a shearing force (on its narrow face) of magnitude 9.0×104 N. The lower edge is riveted to the floor. How much is the upper edge displaced, if the shear modulus of lead is 5.6×1090 Pa?
View SolutionA square lead slab of sides 50cm and thickness 5.0cm is subjected to a shearing force ( on its narrow face ) of magnitude 9.0×104N . The lower edge is riveted to the floor. How much is the upper edge displaced if the shear modulus of lead is 5.6×109Pa ?
View Solution
Knowledge Check
A square lead slab of side 50 cm and thickness 10 cm is subjected to a shearing force (on its narrow face) of 9×104N. The lower edge is riveted to the floor. How much will the upper edge be displaced? (Shear modulus of lead=5.6×109Nm−2)
A0.16mmB1.6mmC0.16 cmD1.6 cm
A square lead slab of side 50 cm and thickness 10 cm is subjected to a shearing force (on its narrow face) of 9×104N. The lower edge is riveted to the floor. How much will the upper edge be displaced? (Shear modulus of lead=5.6×109Nm−2)
View SolutionA lead cube of side 20 cm is subjected under a shearing force of magnitude 5.6x106N. If the lower face of the cube is fixed, then the displacement in the upper face is (If shear modulus of lead is 5.6⋅106Nm2)
View SolutionA metalic cube whose side is 10cm is subjected to a shearing force of 100 N. The top face is displaced through 0.25cm with respect to the bottom . Calculate the shearing stress, strain and shear modulus.
View SolutionA metallic cube whose each side is 10 cm is subjected to a shearing force of 100 kgf. Calculate the shearing produced.
View SolutionA metal cube of side 20cm is subjected to a shearing force of 4000N. The top face is displaced through 0.50 cm with respect to the bottom. Find the rigidity modulus of the metal.
View SolutionOne of the square faces of a metal slab of side 50 cm and thickness 20 cm is rigidly fixed on a horizontal surface. If a tangential force of 30 N is applied to the top face and it is known that the shear modulus of the material is 4×1010N/m2, then the displacement (in m) of the top face is
View SolutionA metallic cube of side 10 cm is subjected to a shearing force of 300 kgf. The top force is displaced through 0.25 cm with respect ot the bottom ? Calculate the shearing strain produced .
View Solution
