Derive an equation for displacement of a projectile fired at an angle θ from the ground.
Displacement of a Projectile :
Suppose a body is projected up with an initial velocity u in a direction making an angle θ with the ground.
Resolving u in horizontal (ux) and vertical components (uy):
ux=ucosθ,uyusinθ

For horizontal motion, ux=ucosθ
By using equation
x=horizontal velocity×time
x=ucosθ×t
t=xucosθ
For vertical motion
uy=usinθ,accelerationay=g
Now, using equation
s=ut+12at2
y=usinθ(xucosθ)+12(−g)×(xucosθ)2
y=xtanθ−12gx2u2cos2θ
y=xtanθ−gx22u2cos2θ
This equation represents a parabola.
Topper's Solved these Questions
- SAMPLE QUESTION PAPER - 01 Book:ICSEChapter:SAMPLE QUESTION PAPER - 01 Exercise:SECTION - D
Explore 12 Videos
- SAMPLE QUESTION PAPER - 01 Book:ICSEChapter:SAMPLE QUESTION PAPER - 01 Exercise:SECTION - B
Explore 14 Videos
- PROPERTIES OF MATTERBook:ICSEChapter:PROPERTIES OF MATTERExercise:MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS
Explore 12 Videos
- SAMPLE QUESTION PAPER 4Book:ICSEChapter:SAMPLE QUESTION PAPER 4Exercise:SECTION - D (Answer all questions.)
Explore 7 Videos
Similar Questions
The position of a projectile launched from the origin at t = 0 is given by s=(40ˆi+50ˆj)mat t=2s. if the projectile was launched at an angle θ from the horizontal, then θis (take g = 10 ms–2
View SolutionA particle is executing vertical SHM about the highest point of a projectile. When the particle is at the mean position, the projectile is fired from the ground with velocity u at an angle θ with the horizontal.The projectile hits the oscillating particle .Then, the possible time period of the particle is
View Solution
Knowledge Check
Galileo writes that for angles of projection of a projectile at angles (45+θ) and (45−θ), the horizontal ranges described by the projectile are in the ratio of (if θ≤45)
A2:1B1:2C1:1D2:3
A point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
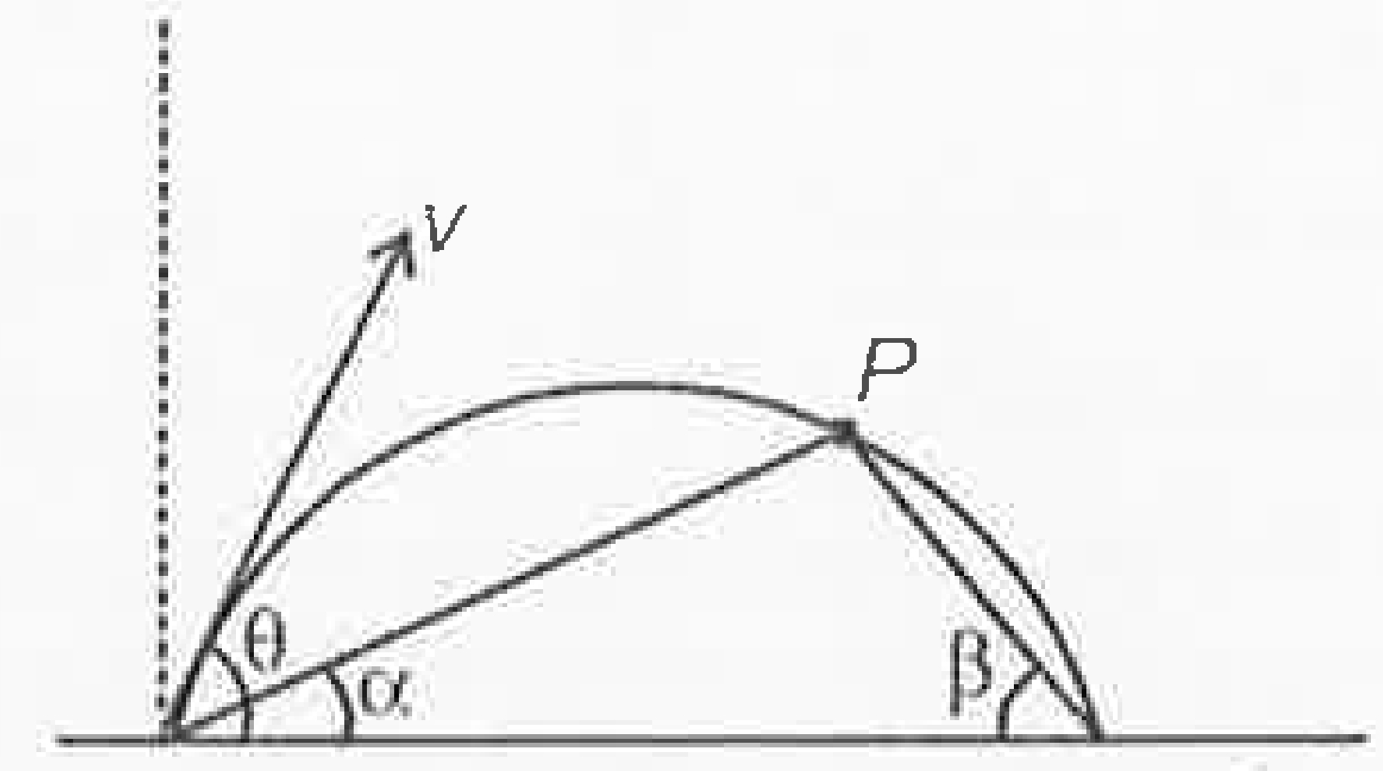
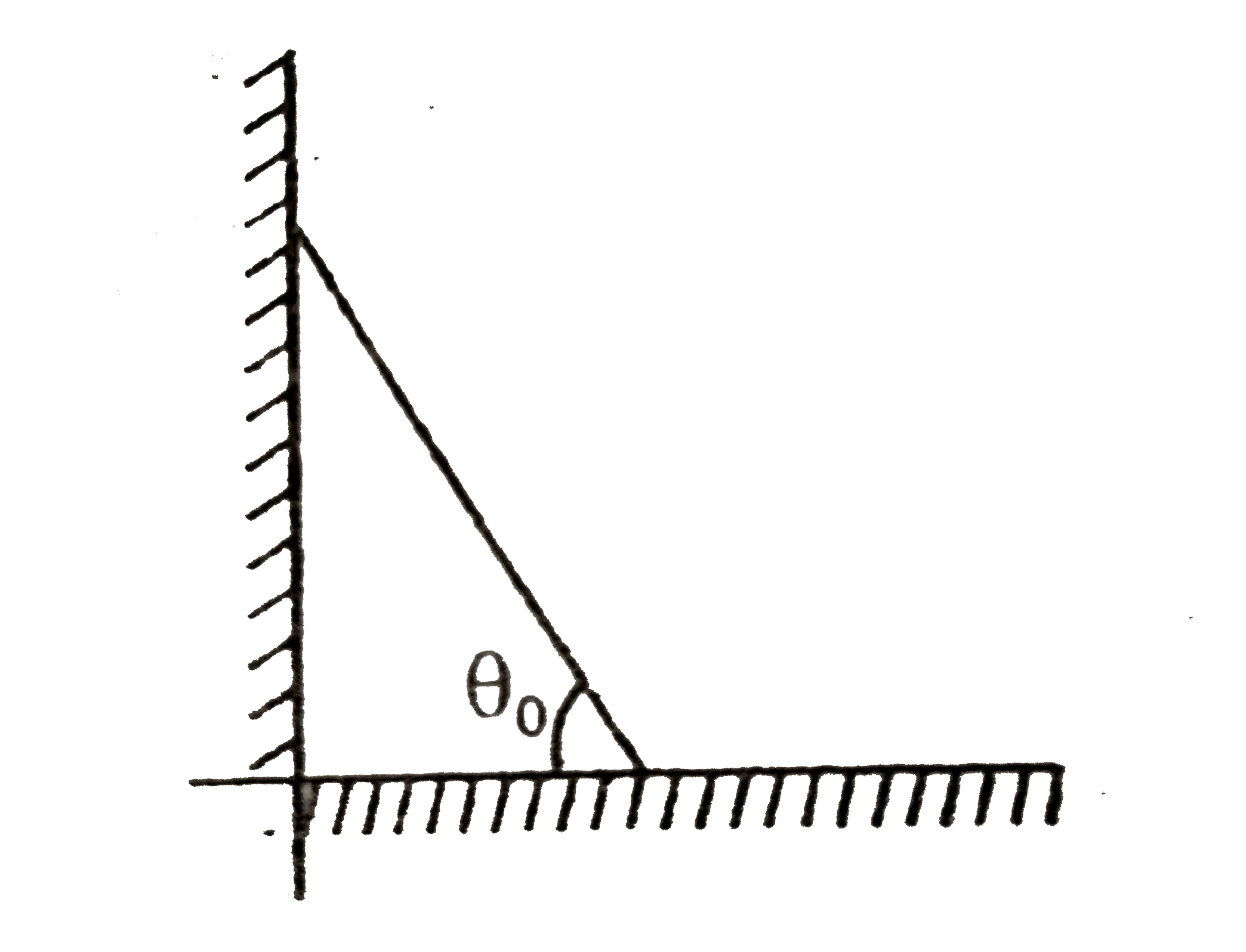
View SolutionA uniform rod is lying against a smooth wall and on a smooth floor. It is relased from rest when it makes an angle θ0 with the ground. Find the angle rod will make with the ground it just leaves the contact with the walls.
View SolutionA projectile is thrown from level ground at some angle
View SolutionIn case of a projectile fired at an angle equally inclined to the horizontal and vertical with velocity (u). The horizontal range is:-
View SolutionThe maximum height attained by a projectile when thrown at an angle θ with the horizontal is found to be half the horizontal range. Then θ is equal to
View SolutionFind (a) time of flight , (b) Max.height (c ) Horizontal range of projectile projected with speed (v) making an angle θ with the horizontal direction from ground.
View SolutionA projectile is fired with a velocity v at right angle to the slope inclined at an angle θ with the horizontal. The range of the projectile along the inclined plane is

View Solution