Write an expression for force per unit length between two long current carrying wires, kept parallel to each other, in vacuum and hence define an ampere, the SI unit of current
To solve the question, we will break it down into two parts:
1. Deriving the expression for the force per unit length between two long parallel current-carrying wires.
2. Defining an ampere, the SI unit of current.
Step 1: Expression for Force per Unit Length
The force per unit length f between two long parallel wires carrying currents I1 and I2 can be expressed using the following formula:
f=μ02π⋅I1I2r
Where:
- f is the force per unit length between the wires,
- μ0 is the permeability of free space (vacuum),
- I1 and I2 are the currents flowing through the wires,
- r is the distance between the two wires.
Step 2: Definition of an Ampere
An ampere (A) is defined as the amount of current that, when flowing through two parallel long wires placed one meter apart in a vacuum, produces a force of 2×10−7 Newtons per meter of length between them.
In summary:
- 1 ampere is the current that generates a force of 2×10−7 N/m between two parallel wires separated by a distance of 1 meter.
Final Expression
Thus, we have the expression for force per unit length:
f=μ02π⋅I1I2r
And the definition of an ampere:
1 ampere is defined as the current that produces a force of 2×10−7 N/m between two parallel wires separated by 1 meter.
---
2. Defining an ampere, the SI unit of current.
- f is the force per unit length between the wires,
- μ0 is the permeability of free space (vacuum),
- I1 and I2 are the currents flowing through the wires,
- r is the distance between the two wires.
- 1 ampere is the current that generates a force of 2×10−7 N/m between two parallel wires separated by a distance of 1 meter.
Similar Questions
Name and define the SI unit of current.
View SolutionDerive the expression for force per unit length between two long straight parallel current carrying conductors. Hence define one ampere.
View SolutionThe force between two parallel current carrying wires is independent of
View SolutionTwo long wires, carrying currents i1andi2, are placed perpendicular to each other in such a way that they just avoid a contact. Find the magnetic force on a small length dl of the second wire situated at a distance l from the first wire. (figure)

View SolutionDerive an expression for the force between two long parallel current carrying conductors. Use this expression to define SI unit of current.
View SolutionHow much force per unit length acts on a long current carrying conductor X due to a current flowing through another similar conductor Y, kept parallel to it in vacuum ? Use this equation to define an ampere, the fundamental unit of current.
View Solution(a) Derive an expression for the force between two long parallel current carrying conductors .
(b) Use this expression to define S.I. unit of current .
(c) A long straight wire AB carries a current I.A proton P travels with a speed v , parallel to the wire , at a distance d from it in a direction opposite to the current as shown in the figure . What is the force experienced by the proton and what is its direction ?
View SolutionFigure below shows two infinitely long and thin current carrying conductors X and Y kept in vacuum, parallel to each other, at a distance .a..
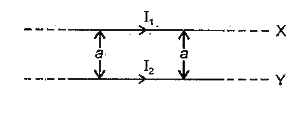
(i) How much force per unit length acts on the conductor y due to the current flowing through X ? Write your answer in terms of (μ04π),I1,I2 and a.
(Derivation of formula is not required.),
(ii) Define ampere, in terms of force between two current carrying conductors.
View SolutionThe force per unit length between two parallel current carrying wires =μ0i1i22πr . The force is attractive when the current is in same direction and repulsive, when the they are in opposite directions. The force between the wires of two parallel wires is shown. We can determine the equilibrium position. Then we displace upper wire by a small distance, keeping lower wire fixed. If the wire returns to or tries to return to its equilibrium position, its equilibrium is stable. We can thus show that upper wire can execute linear simple harmonic motion or not. The length of wire AB is large as compared to separation between the wires.

If wire CD is displaced upward to increase the separation by dh, the magnitude of net force per unit length acting on the wire CD becomes
View SolutionThe force per unit length between two parallel current carrying wires =μ0i1i22πr . The force is attractive when the current is in same direction and repulsive, when the they are in opposite directions. The force between the wires of two parallel wires is shown. We can determine the equilibrium position. Then we displace upper wire by a small distance, keeping lower wire fixed. If the wire returns to or tries to return to its equilibrium position, its equilibrium is stable. We can thus show that upper wire can execute linear simple harmonic motion or not. The length of wire AB is large as compared to separation between the wires.
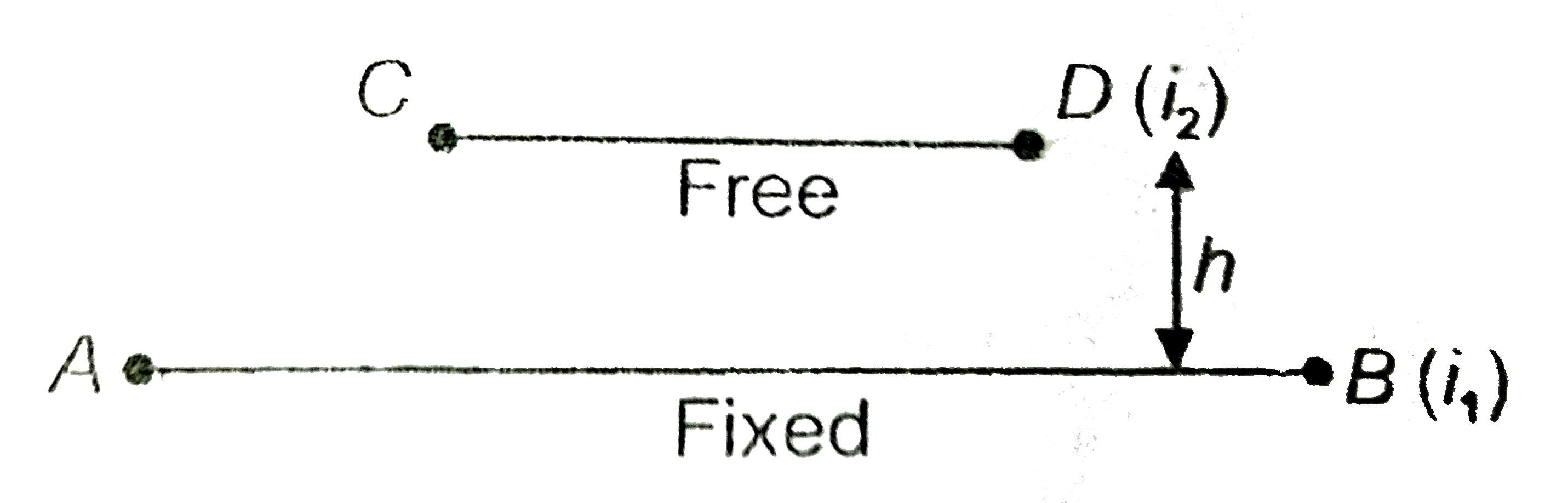
If λ is mass per unit length of wire CD, then the equilibrium separation h is given by
View Solution
