Find the equation of the regression line of y on x, if the observation (x,y) are as follows :
Also, find the estimated value of y when x = 14.
The correct Answer is:y = 22
To find the equation of the regression line of y on x based on the given observations, we will follow these steps:
Step 1: List the Observations
The observations provided are:
(1,4),(2,8),(3,2),(4,12),(5,10),(6,14),(7,16),(8,6),(9,18)
Step 2: Calculate the Necessary Summations
We need to calculate the following:
- Σx
- Σy
- Σxy
- Σx2
- Σy2
Calculations:
1. Sum of x:
Σx=1+2+3+4+5+6+7+8+9=45
2. Sum of y:
Σy=4+8+2+12+10+14+16+6+18=90
3. Sum of xy:
Σxy=(1⋅4)+(2⋅8)+(3⋅2)+(4⋅12)+(5⋅10)+(6⋅14)+(7⋅16)+(8⋅6)+(9⋅18)
=4+16+6+48+50+84+112+48+162=490
4. Sum of x2:
Σx2=12+22+32+42+52+62+72+82+92=1+4+9+16+25+36+49+64+81=285
5. Sum of y2:
Σy2=42+82+22+122+102+142+162+62+182
=16+64+4+144+100+196+256+36+324=1140
Step 3: Calculate the Regression Coefficient byx
The formula for the regression coefficient byx is:
byx=Σxy−Σx⋅ΣynΣx2−(Σx)2n
Where n is the number of observations (which is 9).
Substituting the values:
byx=490−45⋅909285−4529
=490−450285−225=4060=23
Step 4: Calculate ¯x and ¯y
¯x=Σxn=459=5
¯y=Σyn=909=10
Step 5: Write the Equation of the Regression Line
The equation of the regression line is given by:
y−¯y=byx(x−¯x)
Substituting the values:
y−10=23(x−5)
Rearranging gives:
y=23x+(10−23⋅5)
y=23x+(10−103)=23x+303−103=23x+203
Step 6: Find the Estimated Value of y when x=14
Substituting x=14 into the regression equation:
y=23(14)+203=283+203=483=16
Final Result
The equation of the regression line of y on x is:
y=23x+203
And the estimated value of y when x=14 is:
y=16
The observations provided are:
(1,4),(2,8),(3,2),(4,12),(5,10),(6,14),(7,16),(8,6),(9,18)
We need to calculate the following:
- Σx
- Σy
- Σxy
- Σx2
- Σy2
1. Sum of x:
Σx=1+2+3+4+5+6+7+8+9=45
Σy=4+8+2+12+10+14+16+6+18=90
Σxy=(1⋅4)+(2⋅8)+(3⋅2)+(4⋅12)+(5⋅10)+(6⋅14)+(7⋅16)+(8⋅6)+(9⋅18)
=4+16+6+48+50+84+112+48+162=490
Σx2=12+22+32+42+52+62+72+82+92=1+4+9+16+25+36+49+64+81=285
Σy2=42+82+22+122+102+142+162+62+182
=16+64+4+144+100+196+256+36+324=1140
The formula for the regression coefficient byx is:
byx=Σxy−Σx⋅ΣynΣx2−(Σx)2n
Where n is the number of observations (which is 9).
byx=490−45⋅909285−4529
=490−450285−225=4060=23
¯x=Σxn=459=5
¯y=Σyn=909=10
The equation of the regression line is given by:
y−¯y=byx(x−¯x)
Substituting the values:
y−10=23(x−5)
Rearranging gives:
y=23x+(10−23⋅5)
y=23x+(10−103)=23x+303−103=23x+203
Substituting x=14 into the regression equation:
y=23(14)+203=283+203=483=16
The equation of the regression line of y on x is:
y=23x+203
And the estimated value of y when x=14 is:
y=16
Topper's Solved these Questions
Similar Questions
The following observations are given : (1,4), (2, 8), (3, 2), (4,12), (5,10), (6,14), (7,16), (8,6), (9,18).
Estimate the value ofy when the value of x is 10 and also estimate the value ofx when the value of y = 5
View SolutionGiven that the observations are:
(9, -4), (10, -3), (11, -1), (12, 0), (13, 1), (14, 3), (15, 5), (16, 8).
Find the two lines of regression and estimate the value of y when x = 13.5.
View SolutionGiven that the observations are :
(9,−4),(10,−3),(11,−1),(12,0),(13,1),(14,3),(15,5),(16,8). Find the two lines of regression and estimate the value of y when x = 13.5.
View SolutionFind the equations of the two lines of regression for the following observations : (3, 6), (4, 5), (5, 4), (6,3), (7,2). Find the estimation of y for x = 2.5.
View SolutionFind the line of regression of y on x from the following table :
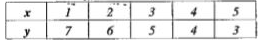
Hence estimate the value of y when x=6.
View SolutionIf 2(130x)+(y012)=(5618), then find the value of x+y.
View SolutionFind the equation of the asymptotes of the hyperbola 3x2+10xy+9y2+14x+22y+7=0
View SolutionFind the equations of the normal to the curve y=x3+2x+6 which are parallel to the line x+14y+4=0.
View SolutionFind the value of x3−8y3−36xy−216, when x=2y+6.
View SolutionFind the value of x3−8y3−36xy−216, when x=2y+6.
View Solution
