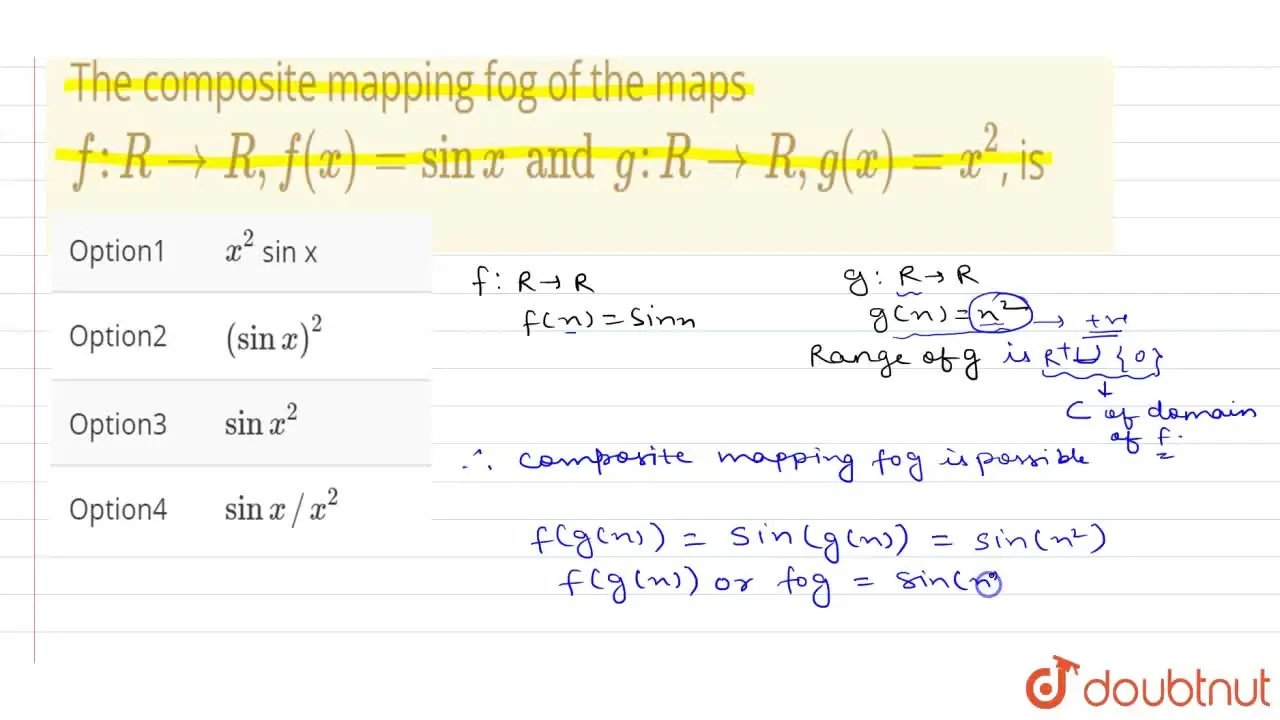
The composite mapping fog of the maps f:R→R,f(x)=sinxandg:R→R,g(x)=x2, is
x2 sin x
(sinx)2
sinx2
sinx/x2
The correct Answer is:C
f:R→R
implies f(x) = sin x and g:R→R
⇒g(x)=x2
Range of g is R+∪{0}, which is the subset of domain of f.
∴ Composition of fog is possible.
fog=f(g(x))=f(x2)
=sinx2
Related Playlists
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (More Than One Correct Option Type Questions)3videos
SETS, RELATIONS AND FUNCTIONS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Passage Based Questions)6videos
SETS, RELATIONS AND FUNCTIONS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise For Session 310videos
SETS, RELATIONS AND FUNCTIONS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)38videos
SEQUENCES AND SERIES
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)17videos
THE STRAIGHT LINES
Similar Questions
If f:R→R, f(x)=x2 and g(x)=2x+1, then
View SolutionLet :R→R ; f(x)=sinx and g:R→R ; g(x)=x2 find fog and gof .
View SolutionIf f:R→R,f(x)=x3andg:R→R,g(x)=2x2+1, and R is the set of real numbers then find fog(x) and gof (x).
View SolutionFind fog(2) and gof(1) when: f:R→R;f(x)=x2+8 and g:R→R;g(x)=3x3+1.
View SolutionFind fog(2) and gof(1) when: f:R→R;f(x)=x2+8 and g:R→R;g(x)=3x3+1.
View SolutionIf f:R→Randg:R→R be two mapping such that f(x) = sin x and g(x) = x2, then
find the values of (fog) √π2and (gof)(π3).View SolutionFind fog (2) and gof (1) when: f:R→R ; f(x)=x2+8 and g:R→R ; g(x)=3x3+1 .
View SolutionThe values of a and b for which the map f:R→R, given by f(x)=ax+b(a,b∈R) is a bijection with fof as indentity function, are
View SolutionFunction f:R→Randg:R→R are defined as f(x)=sinxandg(x)=ex .
Find (gof)(x) and (fog)(x).View SolutionIf f:R→R be defined by f(x)=3x2−5 and g:R→R by g(x)=xx2+1. Then, gof is
View Solution