Question

On a frictionless surface, a block of mass M moving at speed v collides elastically with another block of same mass M which is intially at rest. After collision the first block moves at an angle $\theta$ to its initial direction and has a speed $\frac{v}{3}$. The second block's speed after the collision is

Options

A. `(sqrt(3))/(@)v`
B. `(2sqrt(2))/(3)v`
C. `(3)/(4)v`
D. `(3)/(sqrt(2))v`

Answer

B

Explanation

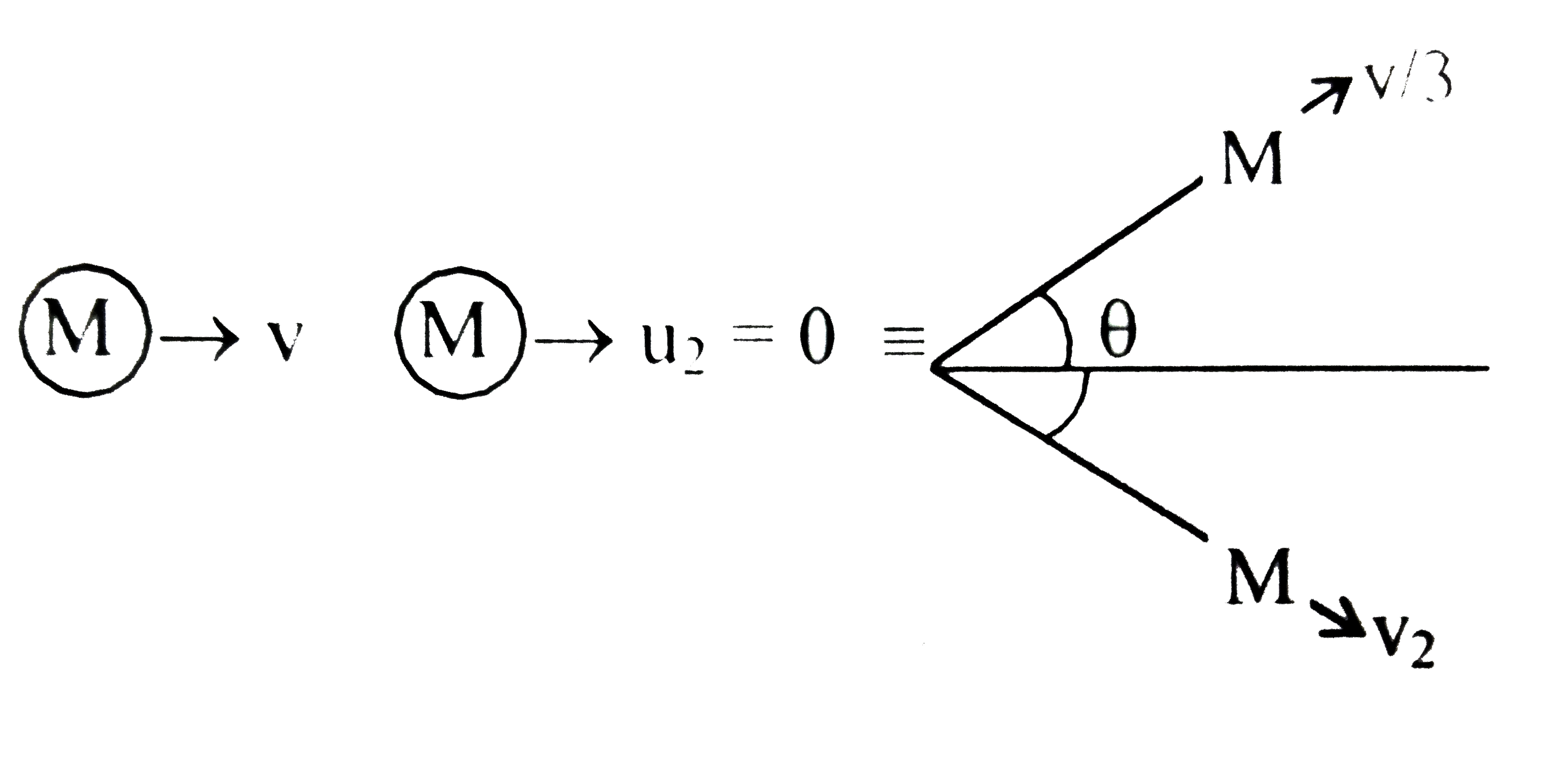
Using conservation of K.E.
`(1)/(2)Mv^(2)+0=(1)/(2)M((v)/(3))^92)+(1)/(2)Mv_(2)^(2)`
`v^(2)=(v^(2))/(9)=v_(2)^(2)`
`v_(2)^(2)=(8v^(2))/(9)`
`v_(2)=(2sqrt(2)v)/(3)`