A chain of length l is placed on a smooth spherical surface of radius R with one of its ends fixed at the top of the sphere. What will be the acceleration of the each element of the chain when its upper end is released? It is assumed that the length of the chain l<(πR2).
gRl
gRl[1−cos(lR)]
glR[1−cos(Rl)]
2lR
To solve the problem of finding the acceleration of each element of a chain placed on a smooth spherical surface when its upper end is released, we can follow these steps:
1. Understanding the Setup:
- We have a chain of length l placed on a smooth spherical surface of radius R.
- One end of the chain is fixed at the top of the sphere, and the other end is free to move.
2. Define Mass per Unit Length:
- Let the total mass of the chain be m.
- The mass per unit length of the chain is given by:
mass per unit length=ml
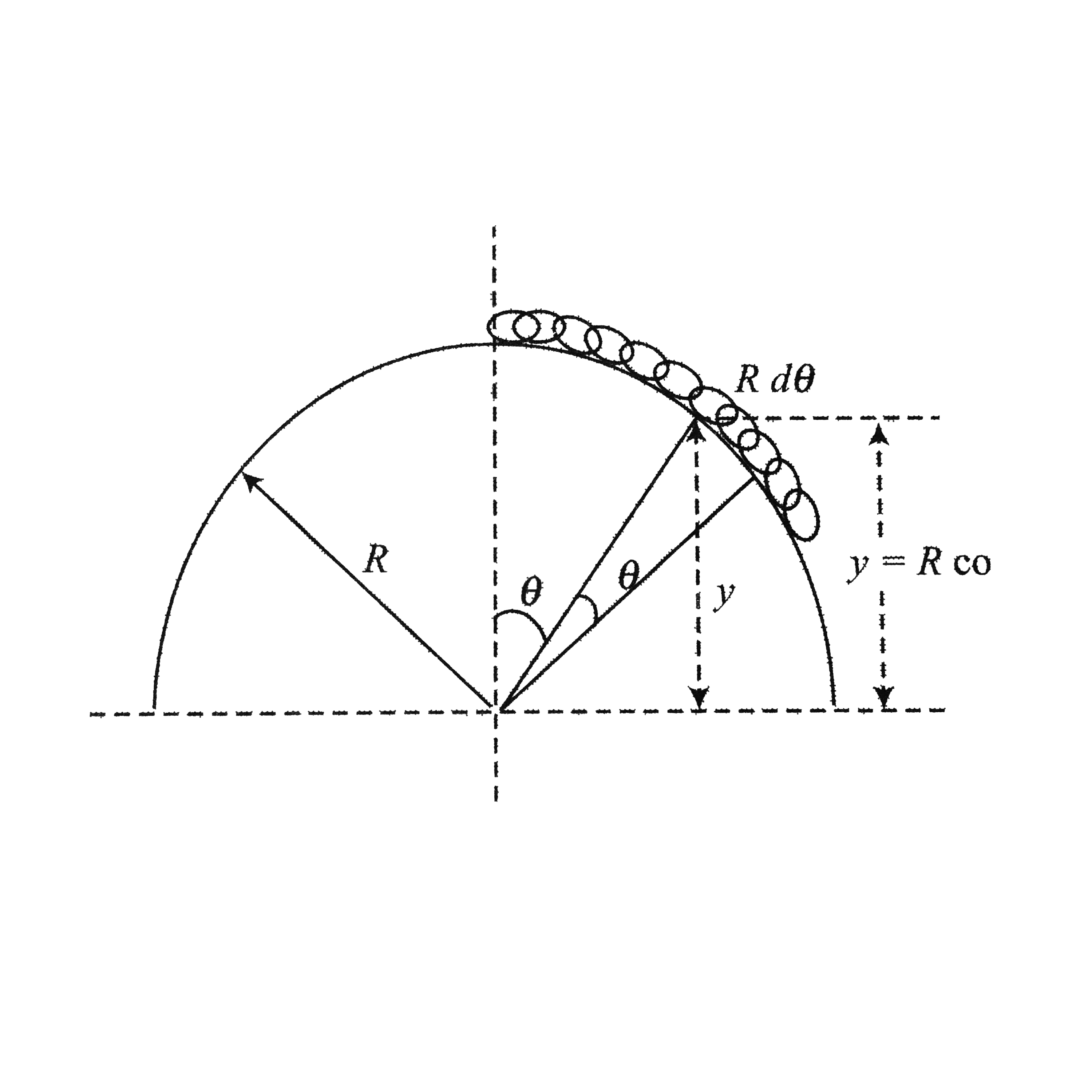
3. Consider an Element of the Chain:
- Take an infinitesimal element of the chain of length dl that subtends an angle dθ at the center of the sphere.
- The mass of this element dm can be expressed as:
dm=ml⋅dl=ml⋅R⋅dθ
4. Calculate the Force Acting on the Element:
- The force acting on the element due to gravity can be expressed as:
dF=dm⋅g⋅sin(θ)
- Substituting for dm:
dF=(ml⋅R⋅dθ)⋅g⋅sin(θ)=m⋅R⋅g⋅sin(θ)l⋅dθ
5. Integrate to Find Total Force:
- The total force F acting on the chain can be found by integrating dF from 0 to α (the angle subtended by the length of the chain):
F=∫α0dF=m⋅R⋅gl∫α0sin(θ)dθ
- The integral of sin(θ) is:
∫α0sin(θ)dθ=−cos(θ)∣∣∣α0=1−cos(α)
- Thus, we have:
F=m⋅R⋅gl(1−cos(α))
6. Calculate the Acceleration:
- The acceleration α of the chain can be found using Newton's second law F=m⋅a:
a=Fm=R⋅gl(1−cos(α))
7. Final Expression for Acceleration:
- Therefore, the acceleration of each element of the chain when the upper end is released is:
a=R⋅gl(1−cos(lR))
- We have a chain of length l placed on a smooth spherical surface of radius R.
- One end of the chain is fixed at the top of the sphere, and the other end is free to move.
- Let the total mass of the chain be m.
- The mass per unit length of the chain is given by:
mass per unit length=ml
- Take an infinitesimal element of the chain of length dl that subtends an angle dθ at the center of the sphere.
- The mass of this element dm can be expressed as:
dm=ml⋅dl=ml⋅R⋅dθ
- The force acting on the element due to gravity can be expressed as:
dF=dm⋅g⋅sin(θ)
- Substituting for dm:
dF=(ml⋅R⋅dθ)⋅g⋅sin(θ)=m⋅R⋅g⋅sin(θ)l⋅dθ
- The total force F acting on the chain can be found by integrating dF from 0 to α (the angle subtended by the length of the chain):
F=∫α0dF=m⋅R⋅gl∫α0sin(θ)dθ
- The integral of sin(θ) is:
∫α0sin(θ)dθ=−cos(θ)∣∣∣α0=1−cos(α)
- Thus, we have:
F=m⋅R⋅gl(1−cos(α))
- The acceleration α of the chain can be found using Newton's second law F=m⋅a:
a=Fm=R⋅gl(1−cos(α))
- Therefore, the acceleration of each element of the chain when the upper end is released is:
a=R⋅gl(1−cos(lR))
Topper's Solved these Questions
Similar Questions
A chain of length l is placed on a smooth spherical surface of radius R with one of its ends fixed at the top of the sphere. What will be the acceleration w of each element of the chain when its upper end is released? It is assumed that the length of the chain l<12πR.
View SolutionA uniform chain of mass m and length l<πR2 is placed on a smooth hemisphere of radius R with on of its ends fixed at the top of the sphere.
(a) Find the gravitational potential energy of chain assuming base of hemisphere as reference.
(b) what will be the tangential acceleraion of the chain when it starts sliding down.
(c ) If the chain slides down the sphere, find the kinetic energy of the chain when it has slipped through an angle β.
View Solution
Knowledge Check
A chain of length l=πR/4 is placed. On a smooth hemispherical surface of radius R with one of its ends fixed at the top of the sphere. Mass of chain is √πkg and R=1m.(g=10m/s2).
If the chain sliped down the sphere, kinetic energy of the chain when it has sliped through an angle θ=π4.A23.4JB63.44JC80 JD97.4JA chain of length l=πR/4 is placed. On a smooth hemispherical surface of radius R with one of its ends fixed at the top of the sphere. Mass of chain is √πkg and R=1m.(g=10m/s2).
The tangential acceleration of the chain when its starts sliding down.A40π(1−1√2)B20π(1−1√2)C10(1−1√2)DzeroA chain of length l=πR/4 is placed. On a smooth hemispherical surface of radius R with one of its ends fixed at the top of the sphere. Mass of chain is √πkg and R=1m.(g=10m/s2).
The gravitational potential energy of the chain considering reference level at the base of hemisphere isA20 JB20√2JC40 JD40√2J
AS chain of length l and mass m lies o the surface of a smooth sphere of radius R>l with one end tied to the top of the sphere. a.Find the gravitational potential energy of the chain with reference level at the centre of the sphere. B. suppose the chin is released and slides down the sphere. Find the kinetic eneergy of the chain, when it has slid through an angle θ c. find the tangential acceleration dvdt of the chain when the chain starts sliding down.
View SolutionA chain of length l and mass m lies of the surface of a smooth hemisphere of radius R>l with one end tied to the top of the hemisphere. Taking base of the hemisphere as reference line, find the gravitational potential energy of the chain.
View SolutionA chain AB of length l is located in a smooth horizontal tube so that its fraction of length h hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?

View SolutionA chain (AB) of length l loaded in a smooth horizontal table so that its fraction of length h hangs freely and toucher the surface of the table with its end B. At a certain moment ,the end A of the chain is set free. With what velocity will this end the chain slip out of the table ?
 .
.
View SolutionA chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B
View Solution
