Draw a neat labelled diagram of Young.s Double Slit experiment. Show that β=λDd, where the terms have their usual meaning (either for bright or dark fringe).
Step-by-Step Solution
1. Draw the Diagram:
- Start by drawing a horizontal line to represent the screen.
- Mark the central maximum (C) at the center of the screen.
- Draw two narrow slits (S1 and S2) parallel to each other and above the screen. Label the distance between the slits as 'd'.
- Draw lines from S1 and S2 to a point P on the screen, which is at a distance 'x' from the central maximum (C).
2. Label the Distances:
- Label the distance from the slits to the screen as 'D'.
- Mark the distances from the central maximum (C) to the point P as 'x'.
- The distance from the center of the slits to the point P can be divided into two parts: CP=x and the distances from C to S1 and S2 as d/2.
3. Path Difference Calculation:
- The path difference (Δ) between the two waves reaching point P is given by:
Δ=S2P−S1P
- Using the right triangle properties, we can express S2P and S1P in terms of D and x:
- For S2P:
S2P2=D2+(x+d/2)2
- For S1P:
S1P2=D2+(x−d/2)2
4. Subtract the Two Equations:
- Subtract the equation for S1P from S2P:
S2P2−S1P2=(x+d/2)2−(x−d/2)2
- This simplifies to:
Δ=S2P−S1P=d⋅xD
5. Relate Path Difference to Fringe Width:
- For constructive interference (bright fringes), the path difference must equal nλ:
Δ=nλ
- Setting the two expressions for Δ equal gives:
d⋅xD=nλ
6. Express Fringe Width (β):
- The fringe width (β) is defined as the distance between two consecutive bright fringes:
β=xn+1−xn
- From the previous equations, we can express:
xn=nλDdandxn+1=(n+1)λDd
- Therefore, the fringe width is:
β=(n+1)λDd−nλDd=λDd
Final Result
Thus, we conclude that the fringe width β is given by:
β=λDd
- Start by drawing a horizontal line to represent the screen.
- Mark the central maximum (C) at the center of the screen.
- Draw two narrow slits (S1 and S2) parallel to each other and above the screen. Label the distance between the slits as 'd'.
- Draw lines from S1 and S2 to a point P on the screen, which is at a distance 'x' from the central maximum (C).
- Label the distance from the slits to the screen as 'D'.
- Mark the distances from the central maximum (C) to the point P as 'x'.
- The distance from the center of the slits to the point P can be divided into two parts: CP=x and the distances from C to S1 and S2 as d/2.
- The path difference (Δ) between the two waves reaching point P is given by:
Δ=S2P−S1P
- Using the right triangle properties, we can express S2P and S1P in terms of D and x:
- For S2P:
S2P2=D2+(x+d/2)2
- For S1P:
S1P2=D2+(x−d/2)2
- Subtract the equation for S1P from S2P:
S2P2−S1P2=(x+d/2)2−(x−d/2)2
- This simplifies to:
Δ=S2P−S1P=d⋅xD
- For constructive interference (bright fringes), the path difference must equal nλ:
Δ=nλ
- Setting the two expressions for Δ equal gives:
d⋅xD=nλ
- The fringe width (β) is defined as the distance between two consecutive bright fringes:
β=xn+1−xn
- From the previous equations, we can express:
xn=nλDdandxn+1=(n+1)λDd
- Therefore, the fringe width is:
β=(n+1)λDd−nλDd=λDd
Thus, we conclude that the fringe width β is given by:
β=λDd
Similar Questions
Draw a neat labelled diagram of Young.s Double Slit experiment. Show that β=λDd, where the terms have their usual meaning (either for bright or dark fringe).
View SolutionDraw neat labeled diagram of a d.c. motor.
View SolutionDraw a neat labelled diagram of Young.s Double Slit experiment. Show that β=λDd, where the terms have their usual meaning (either for bright or dark fringe).
View SolutionDraw a neat and labelled diagram of an experimental setup of Young.s double slit experiment to study the interference of light and show that:
β=λDd
Where the terms have their usual meaning. Show intensity variation in the interference pattern graphically.
View SolutionIn Young.s double slit experiment sodium light is replaced by blue lamp, then the fringe width
View SolutionWhat is the difference between 2.0m and 2.00m.
View SolutionIn Young.s double slit experiment, what is the path difference between the two light waves forming 5th bright band (fringe) on the screen ?
View SolutionIn Young's double slit experiment, the phase difference between the two waves reaching at the location of the third dark fringe is
View SolutionThe figure shows a schematic diagram showing the arrangement of Young.s Double Slit Experiment
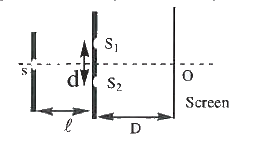
Choose the correct statement (s) related to the wavelength of light used
View SolutionAssertion:- When a width of one of the slits of Young's double slit experiment is double that of the other the brighter frings are nine times brighter then the dark fringes.
Reason:- The amplitudes of the wave is proportional to the width of the slit.
View Solution
