In ΔABC,AD and CE are perpendiculars from A and C on the opposite sides. If AD=6cm.BC=14cm,AB=10cm,find CE.
The correct Answer is:3:4:8
To solve the problem, we need to find the length of CE in triangle ABC, given the following information:
- AD = 6 cm (perpendicular from A)
- BC = 14 cm (base)
- AB = 10 cm (another side)
1. Understand the Triangle and Given Information:
- We have triangle ABC with points A, B, and C.
- AD and CE are the perpendicular heights from points A and C to the opposite sides (BC and AB respectively).
2. Use the Area Formula for Triangle:
- The area of triangle ABC can be calculated in two different ways using the heights AD and CE.
- The area using height AD is:
Area=12×base×height=12×BC×AD
- The area using height CE is:
Area=12×AB×CE
3. Set the Two Area Expressions Equal:
- Since both expressions represent the area of the same triangle, we can set them equal to each other:
12×BC×AD=12×AB×CE
4. Substitute the Known Values:
- Substitute BC = 14 cm, AD = 6 cm, and AB = 10 cm into the equation:
12×14×6=12×10×CE
5. Simplify the Equation:
- The 12 cancels out from both sides:
14×6=10×CE
6. Calculate the Left Side:
- Calculate 14×6:
14×6=84
- So, we have:
84=10×CE
7. Solve for CE:
- Divide both sides by 10 to find CE:
CE=8410=8.4 cm
Conclusion:
Thus, the length of CE is 8.4 cm.
- BC = 14 cm (base)
- AB = 10 cm (another side)
- We have triangle ABC with points A, B, and C.
- AD and CE are the perpendicular heights from points A and C to the opposite sides (BC and AB respectively).
- The area of triangle ABC can be calculated in two different ways using the heights AD and CE.
- The area using height AD is:
Area=12×base×height=12×BC×AD
- The area using height CE is:
Area=12×AB×CE
- Since both expressions represent the area of the same triangle, we can set them equal to each other:
12×BC×AD=12×AB×CE
- Substitute BC = 14 cm, AD = 6 cm, and AB = 10 cm into the equation:
12×14×6=12×10×CE
- The 12 cancels out from both sides:
14×6=10×CE
- Calculate 14×6:
14×6=84
- So, we have:
84=10×CE
- Divide both sides by 10 to find CE:
CE=8410=8.4 cm
Thus, the length of CE is 8.4 cm.
Topper's Solved these Questions
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - EXERCISE 17.3
16 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - REVISION EXERCISE
24 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - EXERCISE 17.1
15 Videos
- PERCENTAGE, PROFIT AND LOSSBOOK - ICSECHAPTER - PERCENTAGE, PROFIT AND LOSSEXERCISE - REVISION EXERCISE
26 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - TRY THIS
14 Videos
Similar Questions
In Δ ABC, BP and CQ are perpendiculars from B and C to the opposite sides. If AB = 16 cm. AC= 24 cm, and CQ=20.4cm, find BP.
View SolutionIn ΔABC, perpendiculars AP and CQ are droped from vertices A and C on opposite sides BC and AB, respectively. If AB=10cm,BC=16cm,AP=8.8cm, find PQ .
View SolutionConstruct Δ ABC with sides AB = 7 cm, BC = 5 cm, CA = 6 cm.
View SolutionIn figure, ABCD is a parallelogram, AE perpendicular to DC and CF perpendicular to AD. If AB = 16cm, AE = 8cm and CF = 10cm, Find AD.
View SolutionIn ΔABC,D and E are the points on sides AB and AC respectively.
Find whether DE || BC, if :
AB = 9 cm, AD = 4 cm, AE = 6 cm and EC = 7.5 cm.
View SolutionIn ΔABC,D and E are the points on sides AB and AC respectively.
Find whether DE || BC, if :
AB = 6-3 cm, EC = 11:0 cm, AD = 0.8 cm and AE = 1.6 cm.
View SolutionIn ΔABC,D and E are points on the sides AB and AC respectively. Find whether DE||BC if
(i) AD= 3cm , BD = 4.5cm, AE = 4cm, AC= 10cm
(ii) AB=7 cm , BD = 4.5cm, AE = 3.5 cm, CE= 5.6cm.
View SolutionIn ΔABC, D and E are points on the sides AB and AC respectively. Find whether DE//BC, if :
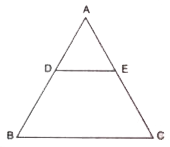
AD=7cm, BD=45cm,AE=35cm and CE=56cm
View SolutionABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:

If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
View SolutionConstruct an Δ ABC with sides AB = 5 cm, BC = 5.5 cm, and CA = 6 cm.
View Solution
