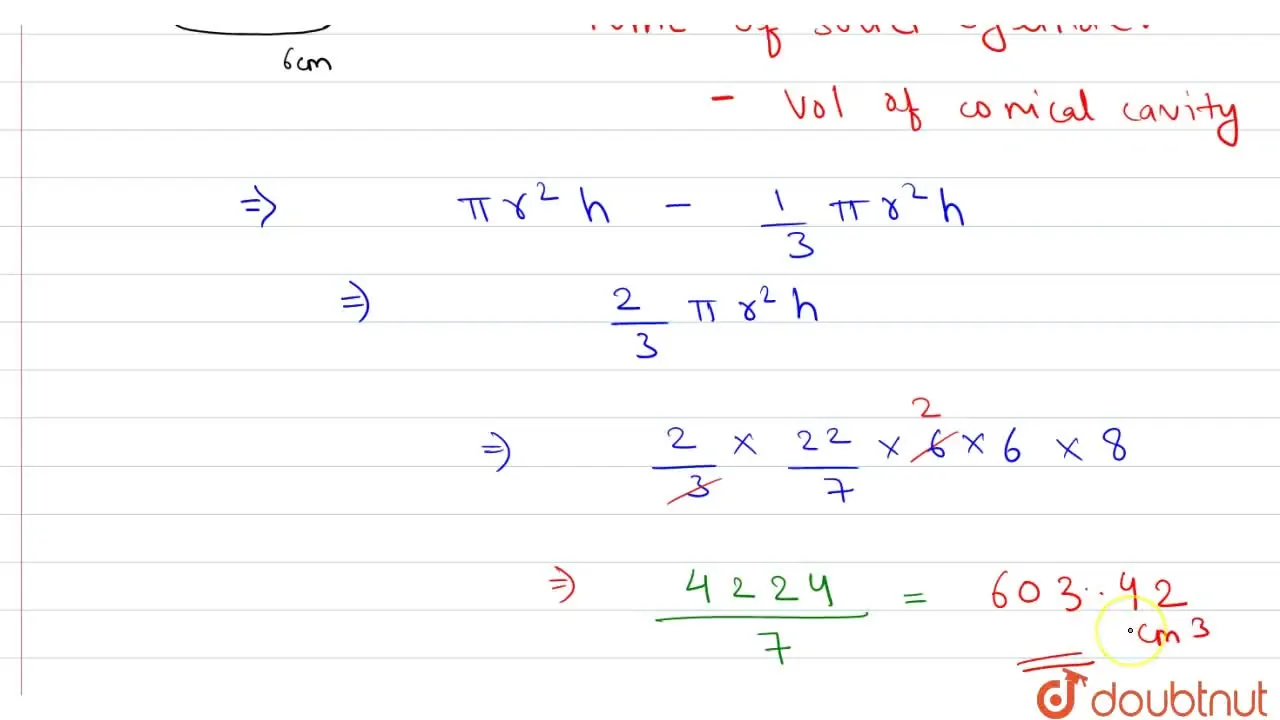
From a solid cylinder, whose height is 8 cm and radius is 6 cm, a conical cavity of height 8 cm and of base radius 6 cm is hollowed out. Find the volume of the remaining solid.
The correct Answer is:60337cm2
Step by step video, text & image solution for From a solid cylinder, whose height is 8 cm and radius is 6 cm, a conical cavity of height 8 cm and of base radius 6 cm is hollowed out. Find the volume of the remaining solid. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - ICSECHAPTER - CYLINDER, CONE AND SPHERE EXERCISE - EXERCISE 20 (A)36videos
CYLINDER, CONE AND SPHERE
- BOOK - ICSECHAPTER - CYLINDER, CONE AND SPHERE EXERCISE - EXERCISE 20 (B)17videos
CYLINDER, CONE AND SPHERE
- BOOK - ICSECHAPTER - CONSTRUCTIONS (CIRCLES)EXERCISE - EXERCISE39videos
CONSTRUCTIONS (CIRCLES)
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(E)68videos
EQUATION OF A LINE
Similar Questions
From a solid cylinder whose height is 16 cm and radius is 12 cm, a conical cavity of height 8 cm and of base radius 6 cm is hollowed out. Find the volume and total surface area of the remaining solid.
View SolutionFrom a solid cube of side 7 cm, a conical cavity of height 7 cm and radius 3 is hollwed out. Find the volume of the remaining solid.
View SolutionFrom a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π=22/7 )
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2 .
View SolutionFrom a solid cylinder of height 36 cm and radius 14 cm, a conical cavity of radius 7 cm and height 24 cm is drilled out. Find the volume and the total surface area of the remaining solid.
View SolutionFrom a solid circular cylinder with height 10 cm and radius of the base 6 cm, a circular cone of the same height and same base is removed. Find the volume of remaining solid and also find the whole surface area.
View SolutionA cone of same height and same base radius is cut from a cylinder of height 8 cm and base radius 6 cm .Find the total surface area and volume of the remaining solid.
View SolutionFrom a right circular cylinder with height 10cm and radius of base 6cm, a right circular cone of the same height and base is removed. Find the volume of the remaining solid.
View SolutionFrom a right circular cylinder with height 10cm and radius of base 6cm, a right circular cone of the same height and base is removed. Find the volume of the remaining solid.
View Solution