Question

From a solid cube of side 7 cm, a conical cavity of height 7 cm and radius 3 is hollwed out. Find the volume of the remaining solid.

Explanation

Given that , side of a solid cube (a) = 7 cm
Height of conical cavity i.e., cone h = 7 cm

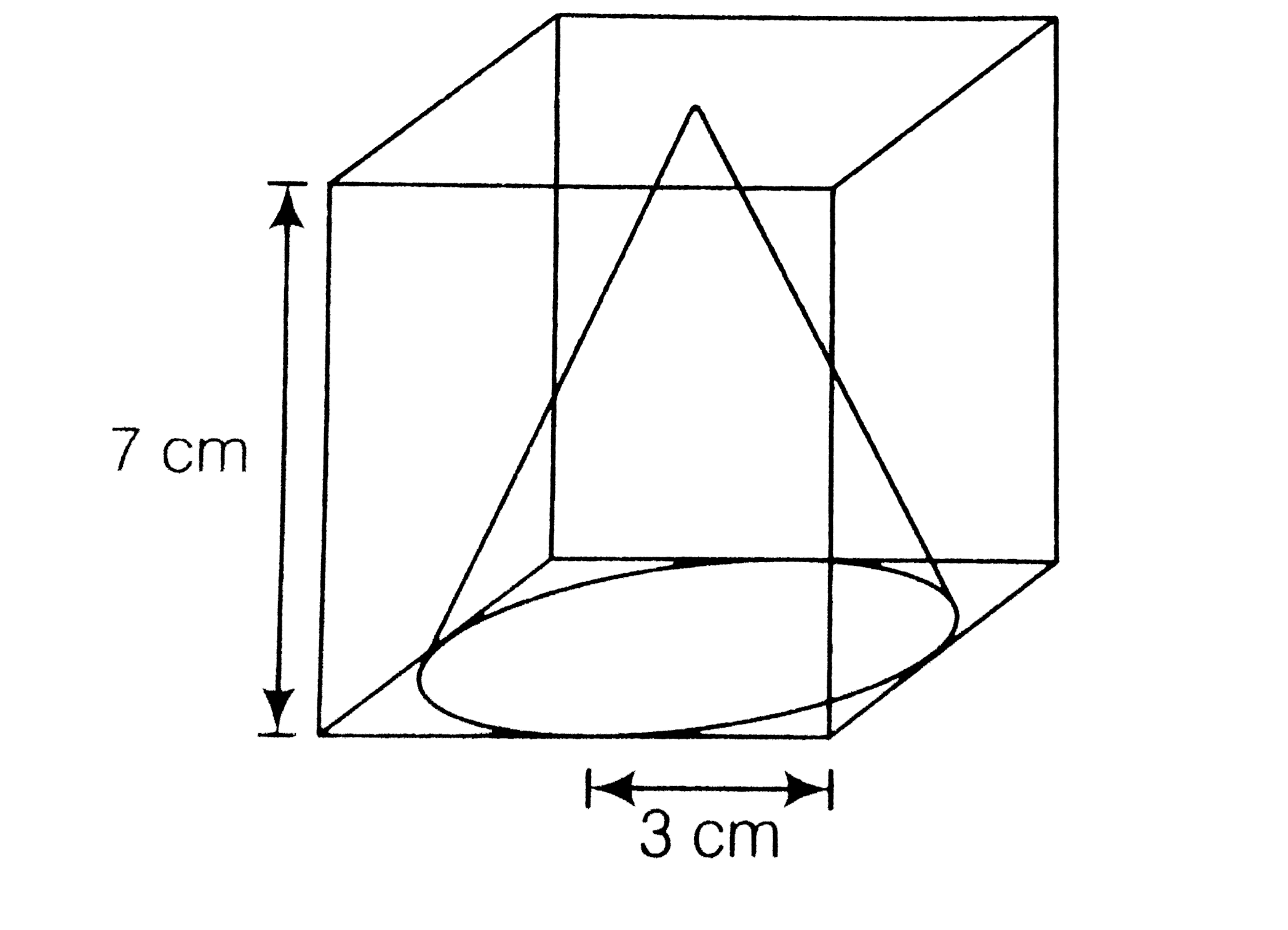
Since, the height of conical cavity and the sode of cube is equal that means the conical cavity fit vertically in the cube.
Radius of conical cavity i.e., cone ,r =3 cm
`rArr` " "Diameter `= 2 xx r = 2xx 3 = 6 cm`
Since, the diameter is less than the side of a cube that means the base of a conical cavity is
not fit inhorizatal of cube .
Now, volume of cube `= (side)^(3) = a^(3) = (7) = 343 cm^(3)`
and volume of conical cavity i.e., cone `= (1)/(3) pi xx r^(2) xx h`
`= (1)/(3) xx (22)/(7) xx3xx 3 xx7`
` = 66 cm^(3)`
`therefore` Volume of remainig soild = Volume of cube - Volume of conical cavity
` = 343 - 66 = 277 cm^(3)`
Hence, the required volume of solid is `277 cm^(3)`.