In a cyclic -quadrilateral PQRS angle PQR =135∘ , Sides SP and RQ produced meet at point A whereas sides PQ and SR produced meet at point B. If ∠A:∠B=2:1. find angles A and B
The correct Answer is:∠A=60∘and∠B=30∘
To solve the problem, we need to find angles A and B in the cyclic quadrilateral PQRS, given that angle PQR = 135° and the ratio of angles A to B is 2:1.
1. Identify the Given Information:
- Angle PQR = 135°
- The ratio of angles A to B = 2:1
2. Use the Property of Cyclic Quadrilaterals:
- In a cyclic quadrilateral, the sum of opposite angles is 180°. Therefore, we can find angle S:
Angle S+Angle Q=180°
Since angle Q is angle PQR, we have:
Angle S+135°=180°
Angle S=180°−135°=45°
3. Determine Angle PQA:
- Since SP and RQ are produced to meet at point A, angle PQA is supplementary to angle PQR:
Angle PQA+Angle PQR=180°
Angle PQA+135°=180°
Angle PQA=180°−135°=45°
4. Identify Angles at Point B:
- Angle A and angle B are formed by the intersection of the extended lines at points A and B. Since angle PQA = 45°, angle A can be expressed in terms of angle B:
Angle SRQ=Angle S+Angle B=45°+Angle B
5. Set Up the Equation Using the Ratio:
- Let angle B = x. Then angle A = 2x. The sum of angles around point B gives:
45°+45°+x+2x=180°
Simplifying this, we have:
90°+3x=180°
3x=180°−90°=90°
x=30°
6. Calculate Angles A and B:
- Angle B = x = 30°
- Angle A = 2x = 2(30°) = 60°
Final Answer:
- Angle A = 60°
- Angle B = 30°
- Angle PQR = 135°
- The ratio of angles A to B = 2:1
- In a cyclic quadrilateral, the sum of opposite angles is 180°. Therefore, we can find angle S:
Angle S+Angle Q=180°
Since angle Q is angle PQR, we have:
Angle S+135°=180°
Angle S=180°−135°=45°
- Since SP and RQ are produced to meet at point A, angle PQA is supplementary to angle PQR:
Angle PQA+Angle PQR=180°
Angle PQA+135°=180°
Angle PQA=180°−135°=45°
- Angle A and angle B are formed by the intersection of the extended lines at points A and B. Since angle PQA = 45°, angle A can be expressed in terms of angle B:
Angle SRQ=Angle S+Angle B=45°+Angle B
- Let angle B = x. Then angle A = 2x. The sum of angles around point B gives:
45°+45°+x+2x=180°
Simplifying this, we have:
90°+3x=180°
3x=180°−90°=90°
x=30°
- Angle B = x = 30°
- Angle A = 2x = 2(30°) = 60°
- Angle A = 60°
- Angle B = 30°
Topper's Solved these Questions
- CIRCLESBOOK - ICSECHAPTER - CIRCLESEXERCISE - EXERCISE 17(B)
10 Videos
- CHAPTERWISE REVISION EXERCISEBOOK - ICSECHAPTER - CHAPTERWISE REVISION EXERCISEEXERCISE - CHAPTERWISE REVISION EXERCISE (PROBABILITY)
16 Videos
- CONSTRUCTIONS (CIRCLES)BOOK - ICSECHAPTER - CONSTRUCTIONS (CIRCLES)EXERCISE - EXERCISE
39 Videos
Similar Questions
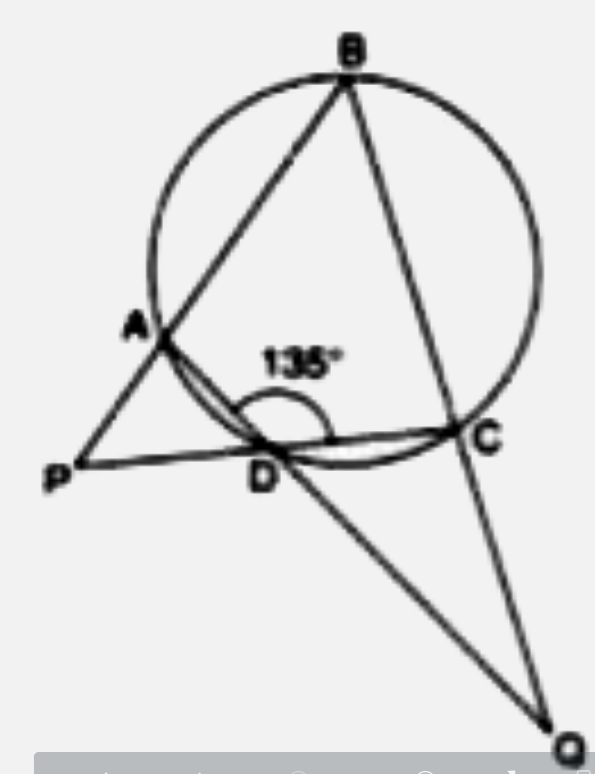
ABCD is a syclic quadrilateral with ∠ADC=135∘. Sides BA and CD produced meet at point P, sides AD and BC produced meet at point Q.If∠P:∠Q=2:1, find angles P and Q.
View SolutionABCD is a cyclic quadrilateral , Sides AB and DC produced meet at point E , whereas sides BC and AD produced meet at point F.
If ∠DCF:∠F:∠E=3:5:4 find the angles of the cyclic quadrilateral ABCD.
View SolutionThe sides AB and DC of a cyclic quadrilateral ABCD when produced meet at E and the sides DA and CB when produced meet at F. Given ∠BEC=38∘,∠BAD=102∘. Find the values of ∠AFB and ∠ADC.
View SolutionIn the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T.
(i) Prove ΔTPS~ΔTRQ.
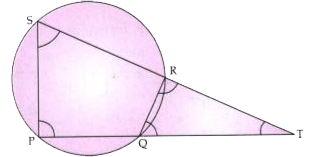
View SolutionABCD is a cyclic quadrilateral. ABandDC are produced to meet in E. Prove that EBC~EDA.
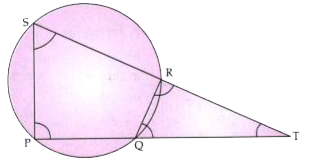
View SolutionIn the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T.
(ii) Find SP if TP = 18 cm, RQ = 4 cm and TR = 6 cm.
View SolutionIn the pentagon ABCDE, angle A=110∘, angle B= 140∘ and angle D= angle E. The sides AB and DC, when produced, meet at right angle. Calculate angles BCD and E.
View SolutionIn quadrilateral PQRS , PS = QR and ∠SPQ=∠RQP . Prove that :
∠QPR=∠PQS
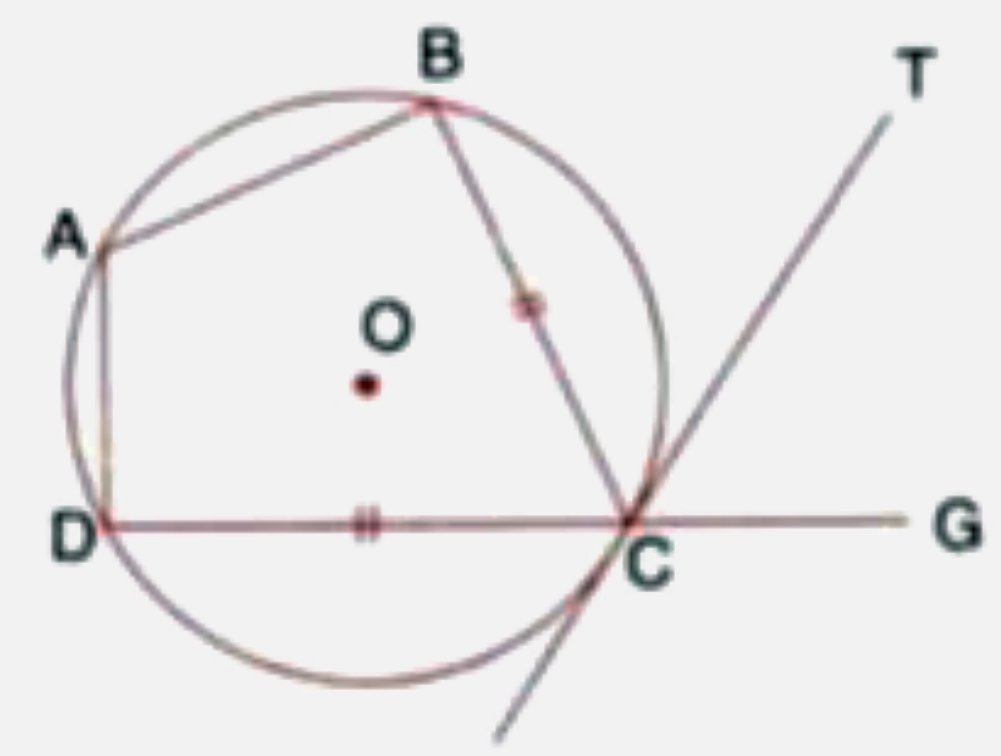
View SolutionIn the figure ABCD is a cyclic quadrilateral with BC=CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG=108∘ and O is the centre of the circle find
(i) angle BCT
(ii) angle DOC
View SolutionIn a cyclic quadrilateral ABCD ∠A:∠C=3:1and angle B : angle D = 1 : 5 find each angle of the quadrilateral
View Solution
