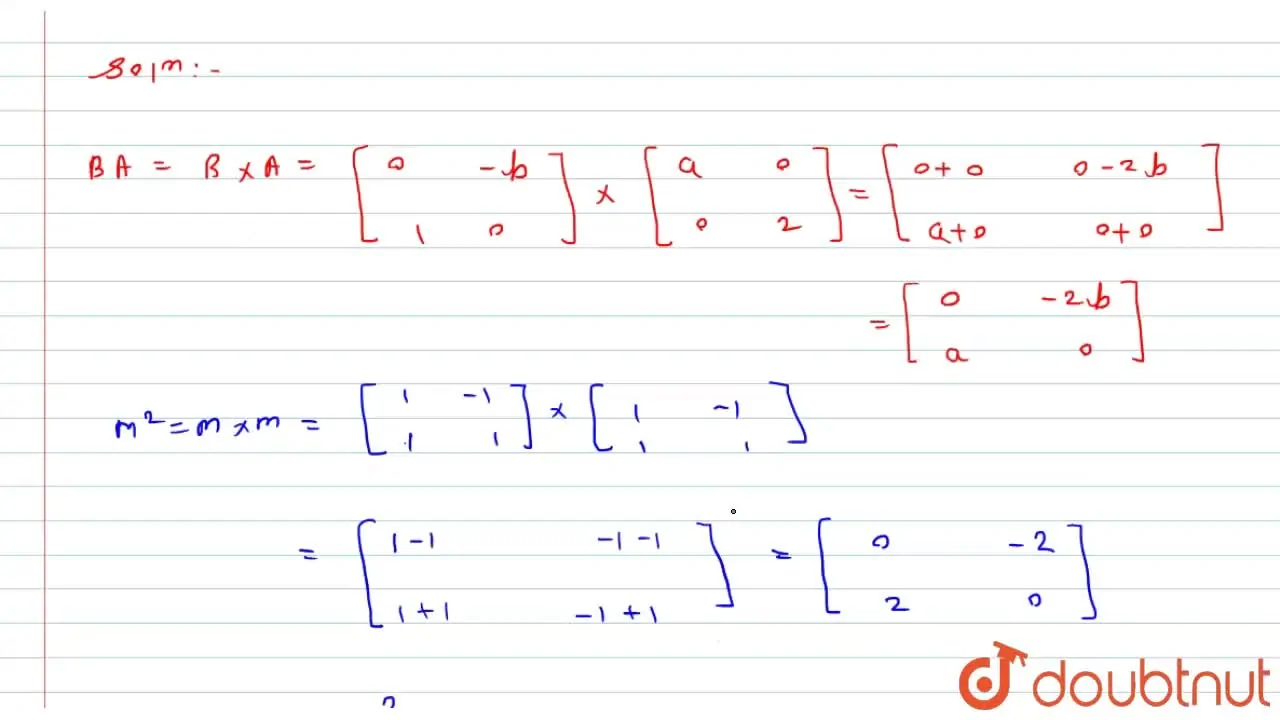
If and , find the values of a and b.
The correct Answer is:a=2 and b=1
Step by step video, text & image solution for If A=[{:(,a,0),(,0,2):}], B=[{:(,0,-b),(,1,0):}], M=[{:(,1,-1),(,1,1):}] and BA=M^2, find the values of a and b. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - ICSECHAPTER - MATRICESEXERCISE - Exercise 9D25videos
MATRICES
- BOOK - ICSECHAPTER - MATRICESEXERCISE - Exercise 9B11videos
MATRICES
- BOOK - ICSECHAPTER - MATHEMATICS-2020 EXERCISE - SECTION-B17videos
MATHEMATICS-2020
- BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (E)23videos
MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)
Similar Questions
Given A=[p002],B=[0−q10],C=[2−222]andBA=C2. Find the values of p and q.
View SolutionIf A=[0−14−3],B=[−56]and3A×M=2B, find matrix M.
View SolutionIf A=[2x01],B=[43601]andA2=B then the value of x is :
View SolutionIf A=[2x01]andB=[43601], find the value of x, given that A2=B
View SolutionLet A=[1021],B=[23−10]. Find A2+AB+B2
View SolutionIf A=[21201],B=[4x01]andA2=B, then the value of x is .........
View SolutionifA=[40−3120]andB=⎡⎢⎣211−234⎤⎥⎦'then find AB and BA.
View SolutionA=⎡⎢⎣a101bd1bc⎤⎥⎦ then find the value of |A|
View SolutionLet A+B+C=[4−101],4A+2B+C=[0−1−32]and9A+3B+C=[0221]then find A
View Solutionif A=[123456]andB=⎡⎢⎣−3−201−4−5⎤⎥⎦, then find AB and BA ,
View Solution