The moment of a force about a given axis depends:
(a) only on the magnitude of force
(b) only on the perpendicular distance of force from the axis
(c) neither on the force nor or the perpendicular distance of force from the axis
(d) both, on the force and its perpendicular distance from the axis.
To solve the question regarding the moment of a force about a given axis, we can follow these steps:
1. Understanding Moment of Force:
The moment of a force (also known as torque) about a given axis is a measure of the tendency of the force to cause rotation about that axis.
2. Formula for Moment of Force:
The moment (M) can be calculated using the formula:
M=F×d
where:
- M = moment of the force,
- F = magnitude of the force applied,
- d = perpendicular distance from the line of action of the force to the axis of rotation.
3. Analyzing the Dependence:
From the formula M=F×d, we can see that:
- The moment depends on the magnitude of the force (F).
- The moment also depends on the perpendicular distance (d) from the axis of rotation to the line of action of the force.
4. Conclusion:
Since the moment of a force is directly proportional to both the magnitude of the force and the perpendicular distance, we conclude that the moment of a force about a given axis depends on both factors.
5. Final Answer:
Therefore, the correct option is:
D: both on the force and perpendicular distance from the axis of rotation.
The moment of a force (also known as torque) about a given axis is a measure of the tendency of the force to cause rotation about that axis.
The moment (M) can be calculated using the formula:
M=F×d
where:
- M = moment of the force,
- F = magnitude of the force applied,
- d = perpendicular distance from the line of action of the force to the axis of rotation.
From the formula M=F×d, we can see that:
- The moment depends on the magnitude of the force (F).
- The moment also depends on the perpendicular distance (d) from the axis of rotation to the line of action of the force.
Since the moment of a force is directly proportional to both the magnitude of the force and the perpendicular distance, we conclude that the moment of a force about a given axis depends on both factors.
Therefore, the correct option is:
D: both on the force and perpendicular distance from the axis of rotation.
Topper's Solved these Questions
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(A) Numericals
18 Videos
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1B
11 Videos
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1A
27 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Video
- HOUSEHOLD CIRCUITS BOOK - ICSECHAPTER - HOUSEHOLD CIRCUITS EXERCISE - EXERCISE -9(B) (MULTIPLE CHOICE TYPE)
2 Videos
Similar Questions
Write the expression for the moment of force about a given axis.
View SolutionState one way to reduce the moment of a given force about a given axis of rotation.
View SolutionState one way to obtain a greater moment of a force about a given axis of rotation.
View SolutionThe moment of inertia of a body about a given axis of rotation depends upon :-
View SolutionThe moment of a force of 50 N about a point is 5 Nm. Find the perpendicular distance of force from that point.
View SolutionStatement-I : Magnitude of torque of a force about a given point does not depend upon the location of the origin of the co-ordinate system.
Statement II: Moment of couple is different for different points in its plane
View SolutionThe moment of inertia of ring about an axis passing through its diameter is I. Then moment of inertia of that ring about an axis passing through its centre and perpendicular to its plane is
View SolutionThe moment of intertia of a disc about an axis passing through its centre and normal to its plane is I. The disc is now folded along a diameter such that the two halves are mutually perpendicular. Its moment of inertia about this diameter will now be
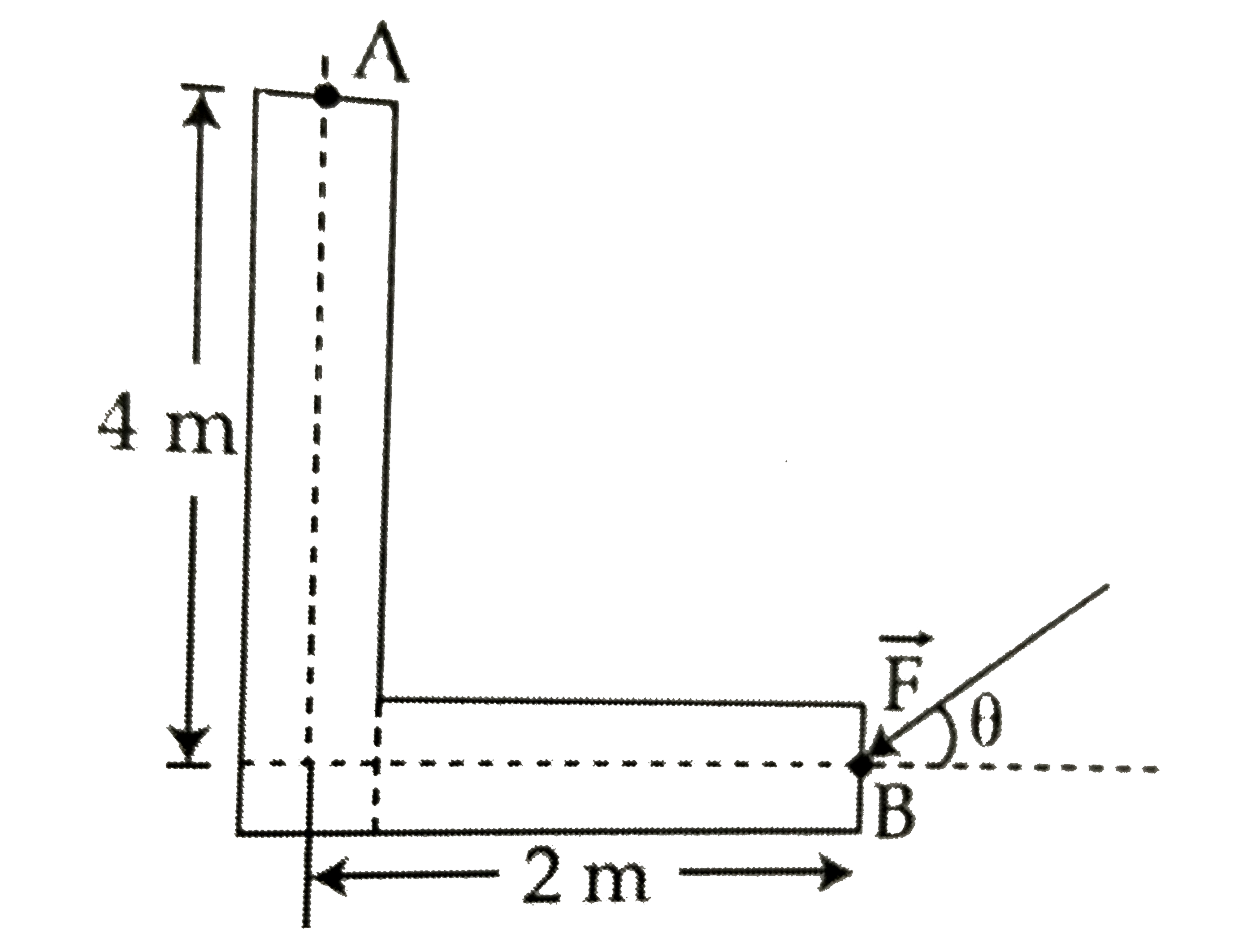
View SolutionA force of 40 N acts on a point B at the end of the L-shaped object, as shown in the figure. The angle θ that will produce maximum moment of the force about point A is given by :
View SolutionThe moment of inertia of a rod about its perpendicular bisector is l, when temperature of rod is increased by ΔT, the increase in the moment of inertia of the rod about same axis (γ = temperature coefficient of volume expansion)
View Solution
