A block of iron floats on mercury. Find the fractionof volume which remains immersed in mercury. (Densities of iron and mercury are 7.8gcm−3 and 13.6gcm−3 respectively).
To solve the problem of finding the fraction of the volume of an iron block that remains immersed in mercury, we can follow these steps:
1. Identify Given Data:
- Density of iron, ρiron=7.8g/cm3
- Density of mercury, ρmercury=13.6g/cm3
2. Let the Volume of the Iron Block be V:
- Let V be the total volume of the iron block.
3. Let the Volume of Mercury Displaced be Vd:
- Let Vd be the volume of the iron block that is submerged in mercury.
4. Apply Archimedes' Principle:
- According to Archimedes' principle, the weight of the iron block is equal to the weight of the mercury displaced by the submerged part of the block.
- Mathematically, this can be expressed as:
Weight of iron block=Weight of mercury displaced
- This can be written as:
V⋅ρiron⋅g=Vd⋅ρmercury⋅g
- Here, g (acceleration due to gravity) cancels out from both sides.
5. Rearranging the Equation:
- We can simplify the equation to:
V⋅ρiron=Vd⋅ρmercury
- Rearranging gives:
VdV=ρironρmercury
6. Substituting the Densities:
- Substitute the known densities into the equation:
VdV=7.813.6
7. Calculating the Fraction:
- Now calculate the fraction:
VdV=7.813.6≈0.574
8. Conclusion:
- Therefore, the fraction of the volume of the iron block that remains immersed in mercury is approximately 0.574.
Final Answer:
The fraction of the volume of the iron block that remains immersed in mercury is 0.574.
---
- Density of iron, ρiron=7.8g/cm3
- Density of mercury, ρmercury=13.6g/cm3
- Let V be the total volume of the iron block.
- Let Vd be the volume of the iron block that is submerged in mercury.
- According to Archimedes' principle, the weight of the iron block is equal to the weight of the mercury displaced by the submerged part of the block.
- Mathematically, this can be expressed as:
Weight of iron block=Weight of mercury displaced
- This can be written as:
V⋅ρiron⋅g=Vd⋅ρmercury⋅g
- Here, g (acceleration due to gravity) cancels out from both sides.
- We can simplify the equation to:
V⋅ρiron=Vd⋅ρmercury
- Rearranging gives:
VdV=ρironρmercury
- Substitute the known densities into the equation:
VdV=7.813.6
- Now calculate the fraction:
VdV=7.813.6≈0.574
- Therefore, the fraction of the volume of the iron block that remains immersed in mercury is approximately 0.574.
The fraction of the volume of the iron block that remains immersed in mercury is 0.574.
Topper's Solved these Questions
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A)
28 Videos
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A) (Multiple Choice Question)
3 Videos
- SOUNDBOOK - ICSECHAPTER - SOUNDEXERCISE - TOPIC 2 Infrasonic and Ultrasonic Waves ( 3 Marks Questions)
3 Videos
Similar Questions
A tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively
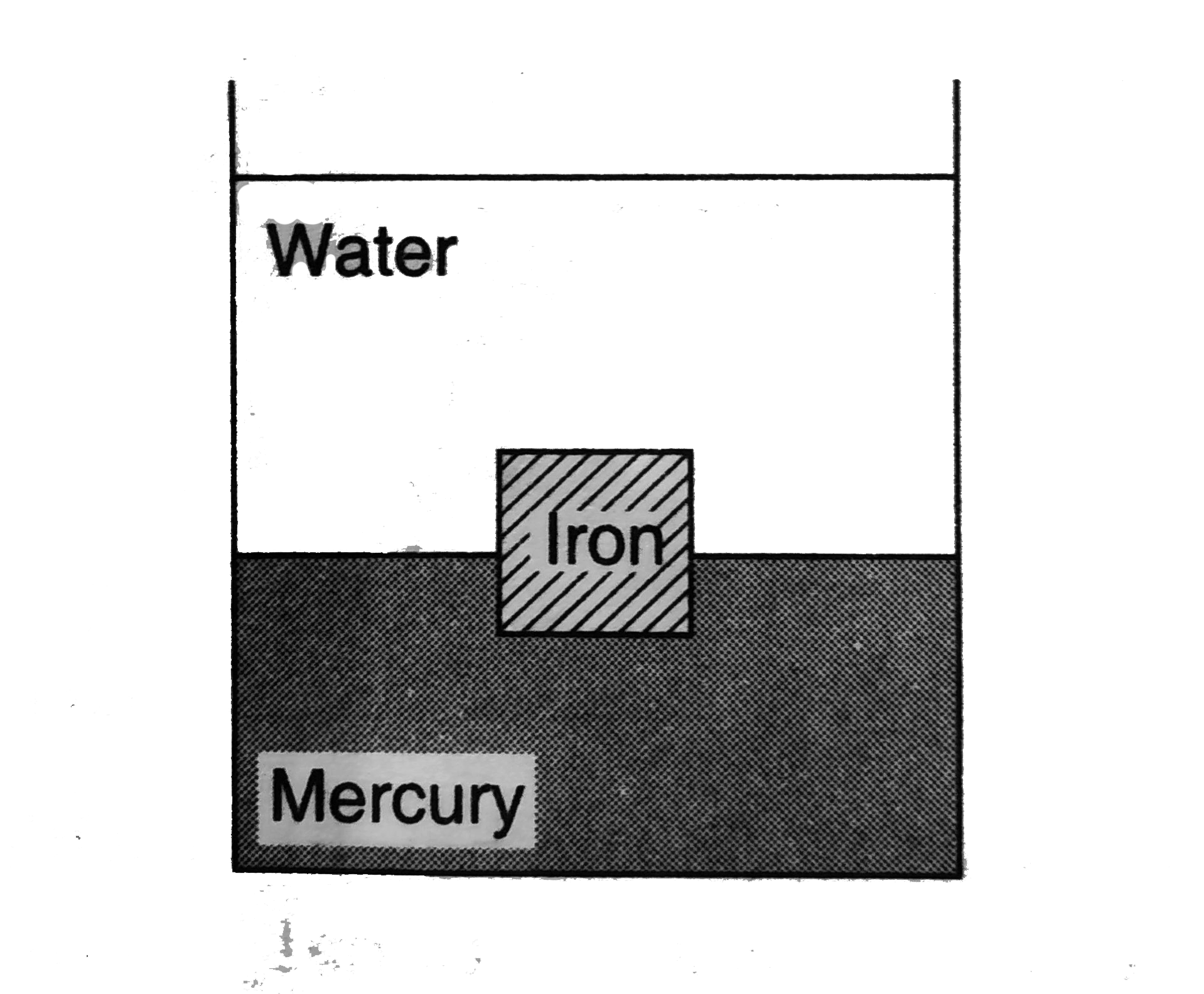
View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively

View SolutionA cube of iron of edge 5cm floats on the surface of mercury, contained in a tank. Water is then poured on top, so that the cube just gets immersed. Find the depth of water layer. (Specific gravities of iron-and mercury are 7.8 and 13.6, respectively.)
View SolutionA cube is floating on mercury with (1/5)th of its volume submerged. If suficient water Is added to cover the cube, what fraction of its volume will remain immersed in mercury ? Density of mercury = 13600kg/m3.
View SolutionA platinum sphere floats in mercury. Find the percentage change in the fraction of volume of sphere immersed in mercury whenthe temperature is raised by 80∘C (volume expansivity of mercury is 182××10−6/∘C and linear expansivity of platinum 9×10−6/∘C)
View SolutionA piece of iron (density 7.6 g cm−3 ) sinks in mercury (density 13.6g cm−3 ).
View SolutionA piece of metal floats on mercury. The coefficient of volume expansion of metal and mercury are γ1andγ2, respectively. if the temperature of both mercury and metal are increased by an amount ΔT, by what factor does the frection of the volume of the metal submerged in mercury changes ?
View SolutionThe interanal radius of one limb of a capillary U−tube is r1=1mm and the internal radius of the second limb is r2=2mm, the tube is filled with same mercury, and one of the limbs is connected to a vecuum pump. The surface tension & density of mercury are 480dyn/cm & 13.6gm/cm3 respectively (assume contact angle to be θ=180∘)(g=9.8m/s2)
What will be the difference in air pressure when the mercury levels in both limbs are at the same height ?
View SolutionExplain why an iron nail floats on mercury, but it sinks in water.
View SolutionA glass vessel is filled up to 3/5th of its volume by mercury. If the volume expansivities of glass and mercury be 9×10−6/∘C and 18×10−5/∘C respectively, then the coefficient of apparent expansion of mercury is
View Solution
