Question

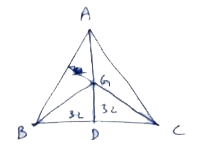
In a $\Delta ABC$ all three medians AD, BE and CF intersects at o. If area of ABC IS 10 z sq units, then find area of quadrilateral BDOF .

Answer

34 . 5 q . Units

Explanation

To find the area of quadrilateral BDOF in triangle ABC, we can follow these steps:

Step 1: Understand the Triangle and Medians
In triangle ABC, we have three medians AD, BE, and CF that intersect at point O. The area of triangle ABC is given as 10z square units.

Step 2: Divide the Triangle into Smaller Triangles
The medians divide triangle ABC into six smaller triangles (AOB, BOC, COA). Since the medians intersect at point O, these six triangles will have equal areas.

Step 3: Calculate the Area of Each Smaller Triangle
Since the total area of triangle ABC is 10z square units and it is divided into six equal parts, the area of each smaller triangle (e.g., AOB, BOC, COA) can be calculated as follows:
\( \text{Area of each triangle} = \frac{\text{Total Area}}{6} = \frac{10z}{6} = \frac{5z}{3} \text{ square units} \)

Step 4: Identify the Area of Quadrilateral BDOF
Quadrilateral BDOF is made up of triangles BOD and EOF. Each of these triangles is one of the six smaller triangles formed by the medians. Therefore, the area of quadrilateral BDOF can be calculated as:
\( \text{Area of BDOF} = \text{Area of triangle BOD} + \text{Area of triangle EOF} = \frac{5z}{3} + \frac{5z}{3} = \frac{10z}{3} \text{ square units} \)

Step 5: Final Calculation
Thus, the area of quadrilateral BDOF is:
\( \text{Area of BDOF} = \frac{10z}{3} \text{ square units} \)

Final Answer
The area of quadrilateral BDOF is \(\frac{10z}{3}\) square units.

---