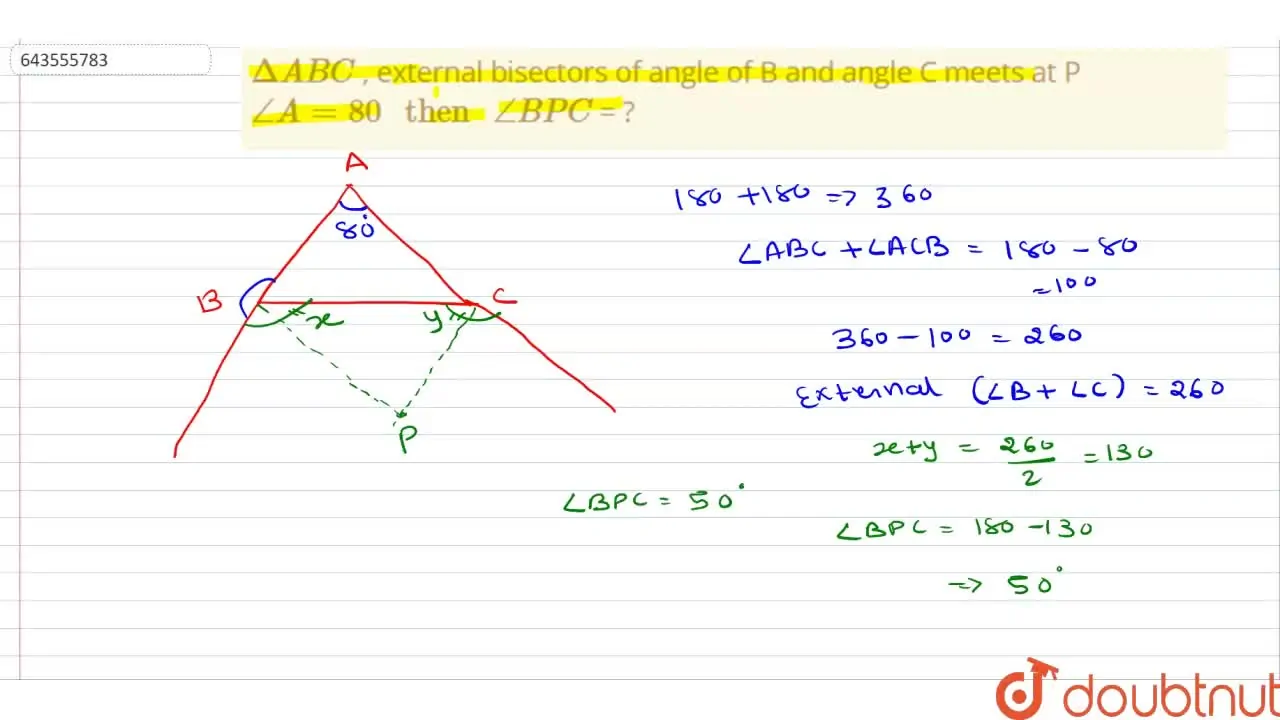
ΔABC ,angle B external angle C meets at P ∠A=80 then ∠BPC = ?
The correct Answer is:40∘
Step by step video, text & image solution for Delta ABC ,angle B external angle C meets at P angle A = 80 " then " angle BPC = ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
ΔABC ,angle B external angle C meets at P ∠A=80 then ∠BPC = ?
View SolutionIn a ΔABC, angle bisector of exterior ∠B&∠C meets at point P, ∠BPC=65∘ then find ∠A .
View SolutionIn ΔABC, ∠A+∠B=65∘,∠B+∠C=140∘ then find ∠B.
View SolutionIf in a ΔABC angle bisectors of exterior angle ∠Band∠C intersect at P ∠BPC=40∘ find ∠A
View SolutionIn a ΔABC, angle bisector of interior angle B and exterior angle C intersect at P . If ∠A=70∘ . Find ∠BPC.
View SolutionThe sides AB and AC of △ABC are produced to points D and E, respectiely. The bisectors of ∠CBD and ∠BCE meet at P. If ∠A=82∘, then the measure of ∠P is :
View SolutionIn a ΔABC,∠C=3∠B=2(∠A+∠B), then ∠B = ?
View SolutionIn a triangle ABC, AB=BC and ∠A=60∘. Find ∠B.
View SolutionIn △ABC,∠C is the greatest angle , then
View SolutionThe sides AB and AC of △ABC are produced to points D and E, respectively. The bisectors of ∠CBDand∠BCE meet at P. If ∠A=72∘, then the measure of ∠P is:
View Solution