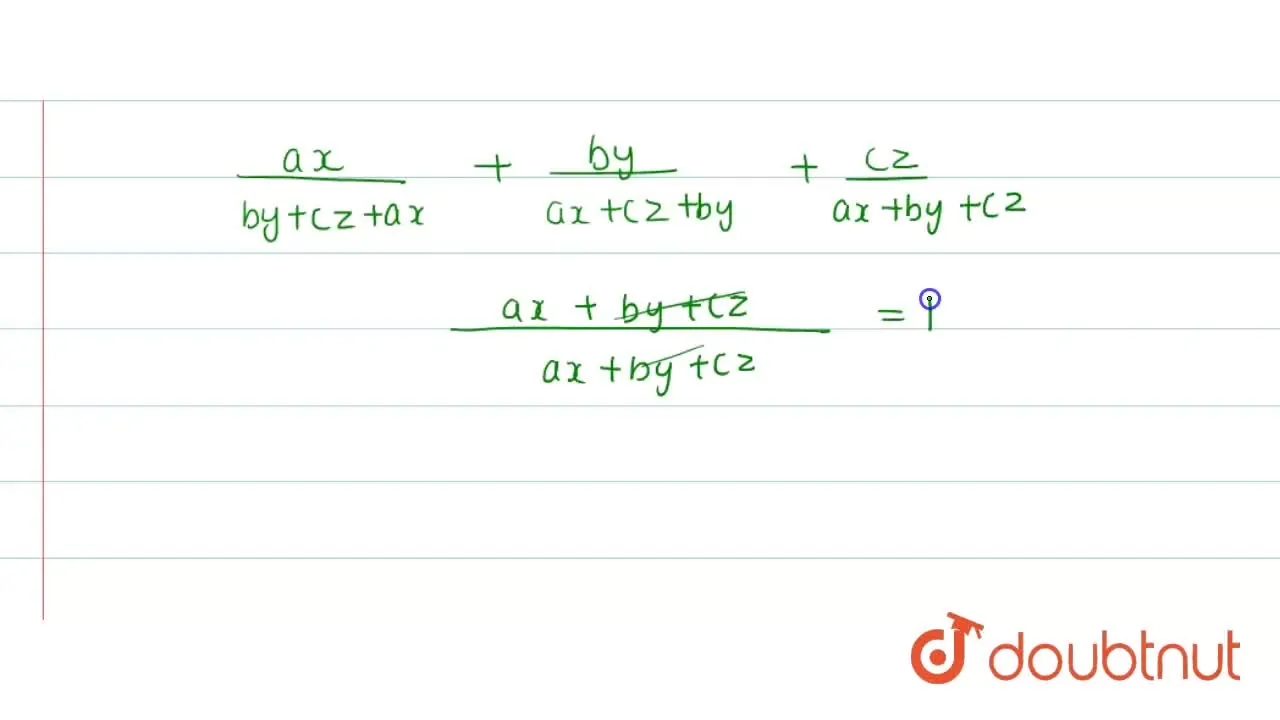
x2by+cz=y2ax+cz=z2ax+by=1 find
ax+a+by+b+cz+c= ?
The correct Answer is:1
Step by step video, text & image solution for (x^(2))/(by + cz) = (y^(2))/(ax + cz)= (z^(2))/(ax + by)=1 find (a)/(x+a) + (b)/(y+b)+ (c )/(z+c )= ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
x2by+cz=y2ax+cz=z2ax+by=1 find
xx+a+yy+a+zz+c= ?View SolutionIf x2by+cz=y2ax+cz=z2ax+by=2
ax+2a+by+2b+cz+2c= ?View SolutionIf x2by+cz=y2ax+cz=z2ax+by=2
xx+2a+yy+2b+zz+2c= ?View SolutionIf x2by+cz=y2cz+ax=z2ax+by=1, then value of aa+x+bb+y+cc+z is
View SolutionIf a2=by+cz,b2=cz+ax,c2=ax+by, then the value of xa+x+yb+y+zc+z is
View SolutionIf ax−1+4by−2b+9cz−3c=6−a find axx−1+2yy−2b+3zz−3c= ?
View SolutionIf x2−yza=y2−zxb=z2−xyc , then (x+y+z)(a+b+c)=ax+by+cz
View SolutionIf a2=by+cz,b2=cz+ax,c2=ax+by, then the value of xa+x+yb+y+zc+z is
View SolutionIf xb−c=yc−a=za−b, prove that ax+by+cz=0
View SolutionShow that 3√ax2+by2+cz2=3√a+3√b+3√c , if ax3=by3=cz3 and 1x+1y+1z=1 .
View Solution