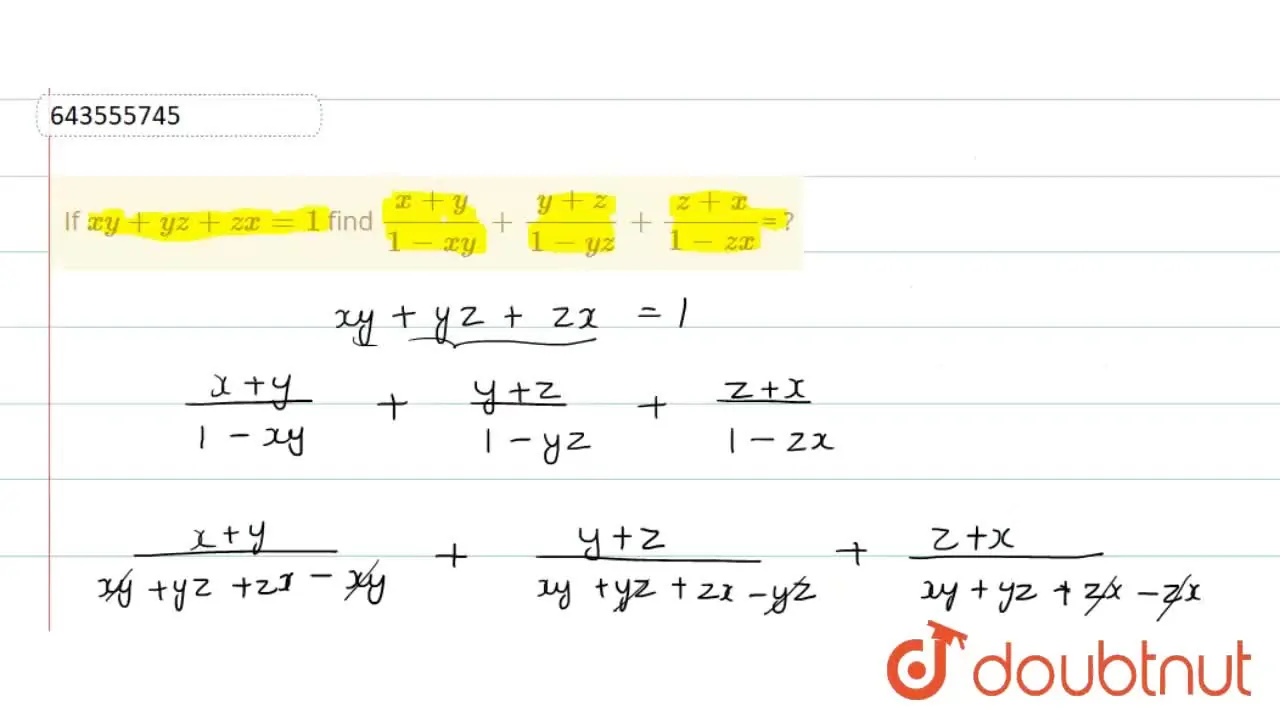
If xy+yz+zx=1 find x+y1−xy+y+z1−yz+z+x1−zx= ?
Video Solution
|
Answer
Step by step video, text & image solution for If xy + yz + zx = 1 find (x+y)/(1-xy) + (y+z)/(1-yz) + (z + x)/(1-zx)= ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
If xy+yz+zx=1, then
x1−x2+y1−y2+z1−z2=4xyz(1−x2)(1−y2)(1−z2)View SolutionIf xy+yz+zx=1 then (∑x+y1−xy=
View SolutionSimplify-
x−yxy+y−zyz+z−xzxView SolutionIf xy+yz+zx=1, then
x1+x2+y1+y2+z1+z2=1[(1+x2)(1+y2)(1+z2)]1/2View SolutionIf xy+yz+zx=1 then ∑x+y1−xy=
View SolutionIf xy+yz+zx=1, show that x1−x2+y1−y2+z1−z2=4xyz(1−x2)(1−y2)(1−z2)
View SolutionIf xy+yz+zx=1, show that x1−x2+y1−y2+z1−z2=4xyz(1−x2)(1−y2)(1−z2)
View Solutionxy:yz:zx=2:3:7
Find (x+y):(y+z):(z+x)=?View SolutionIf x+y+z=0 then x2yz+y2zx+z2xy= ?
View SolutionIf x+y+z=0, then the value of x2yz+y2zx+z2xy is:
View Solution