The taxi charges in a city contain fixed charges and additional charge/km. The charge for a distance of 10 km is Rs 350 and for 25 km is Rs 800. The charge for a distance of 30 km is:
Rs 900
Rs 950
Rs 800
Rs 750
The correct Answer is:b
To solve the problem step by step, we will set up equations based on the information provided about taxi charges.
Step 1: Define Variables
Let:
- x = fixed charge (in Rs)
- y = charge per kilometer (in Rs)
Step 2: Set Up Equations
From the information given:
1. For a distance of 10 km, the total charge is Rs 350:
x+10y=350(Equation 1)
2. For a distance of 25 km, the total charge is Rs 800:
x+25y=800(Equation 2)
Step 3: Subtract the Equations
To eliminate x, we can subtract Equation 1 from Equation 2:
(x+25y)−(x+10y)=800−350
This simplifies to:
15y=450
Step 4: Solve for y
Now, divide both sides by 15 to find y:
y=45015=30
So, the charge per kilometer y is Rs 30.
Step 5: Substitute y Back to Find x
Now, substitute y back into Equation 1 to find x:
x+10(30)=350
This simplifies to:
x+300=350
Now, subtract 300 from both sides:
x=350−300=50
So, the fixed charge x is Rs 50.
Step 6: Calculate Charge for 30 km
Now that we have both x and y, we can calculate the charge for a distance of 30 km:
Total charge=x+30y
Substituting the values of x and y:
Total charge=50+30(30)=50+900=950
Final Answer
The charge for a distance of 30 km is Rs 950.
---
Let:
- x = fixed charge (in Rs)
- y = charge per kilometer (in Rs)
From the information given:
1. For a distance of 10 km, the total charge is Rs 350:
x+10y=350(Equation 1)
x+25y=800(Equation 2)
To eliminate x, we can subtract Equation 1 from Equation 2:
(x+25y)−(x+10y)=800−350
This simplifies to:
15y=450
Now, divide both sides by 15 to find y:
y=45015=30
So, the charge per kilometer y is Rs 30.
Now, substitute y back into Equation 1 to find x:
x+10(30)=350
This simplifies to:
x+300=350
Now, subtract 300 from both sides:
x=350−300=50
So, the fixed charge x is Rs 50.
Now that we have both x and y, we can calculate the charge for a distance of 30 km:
Total charge=x+30y
Substituting the values of x and y:
Total charge=50+30(30)=50+900=950
The charge for a distance of 30 km is Rs 950.
Topper's Solved these Questions
- NUMBER SYSTEM Book:ADVANCED MATHS BY ABHINAY MATHS ENGLISHChapter:NUMBER SYSTEM Exercise:MULTIPLE CHOICE QUESTIONS
Explore 225 Videos
- MOCK TEST IIBook:ADVANCED MATHS BY ABHINAY MATHS ENGLISHChapter:MOCK TEST IIExercise:MCQ
Explore 100 Videos
- PARTNERSHIPBook:ADVANCED MATHS BY ABHINAY MATHS ENGLISHChapter:PARTNERSHIPExercise:Questions
Explore 26 Videos
Similar Questions
The taxi charges in a city contain fixed charges and additional charge per km. The fixed charge is for a distance of upto 5 km and additional charge/km there after. The charge for a distance of 10 km is ₹350 and for 25 km is ₹800. The charge for a distance of 30 km is :-
एक शहर में टैक्सी का एक निश्चित किराया है और प्रति किमी अतिरिक्त शुल्क है। निश्चित किराया 5 किमी की दूरी के लिए है तथा उसके बाद प्रति किमी के लिए अतिरिक्त किराया है। 10 किमी दूरी का किराया ₹350 है तथा 25 किमी दूरी का किराया ₹800 है तो 30 किमी की दूरी का किराया ज्ञात करें।
View SolutionThe taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 20 km, the charge paid is 205 and for a distance of 25 km, the charge paid is 255. Find the fixed charges and the charge per km. How much does a person have to pay for covering a distance of 50 km? Sol. Let the fixed charges be x and charge per km be y.
View Solution
Knowledge Check
The taxi charges in a city contain fixed charges and additional charge/km. The charge for a distance of 10 km is Rs 350 and for 25 km is Rs 800. The charge for a distance of 30 km is:
ARs 900BRs 950CRs 800DRs 750The taxi charges in a city contain fixed charges and additional charge per kilometre. The fixed charge is for a distance of upto 5 km and additional charge per kilometre there after. The charge for a distance of 10 km is Rs 350 and for 25 km is Rs 800. The charge for a distance of 30 km is
ARs 800BRs 750CRs 900DRs 950
The taxi charges in a city comprise of a fixed charge together with the charge for the distance covered. For a journey of 10 km the charge paid is Rs 75 and for a journey of 15 km the charge paid is Rs 110. What will a person have to pay for travelling a distance of 25 km?
View Solution[" (IV) The taxi charges in a city consist of a fixed charge together with the charge for the "],[" distance covered.For a distance of "10km" ,the charge paid is "₹105" and for a "],[" journey of "15km" ,the charge paid is "₹155" .What are the fixed charges and the "],[" charge per km? How much does a person have to pay for travelling a distance of "],[25km" ? "]
View SolutionThe car hire charges in a city comprise of a fixed charges together with the charge for the distance covered. For a journey of 12 km, the charge paid is Rs 89 and for a journey of 20 km, the charge paid is Rs 145. What will a person have to pay for travelling a distance of 30 km?
View SolutionThe taxi charges in a city comprise of a fixed charge, together with the charge of the distance covered. For a journey of 16 km, the charges paid are Rs 156 and for a journey of 24 km, the charges paid are Rs 204. What will a person have to pay for travelling a distance of 30 km? (a) Rs 236 (b) Rs 240 (c) Rs 248 (d) Rs 252
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
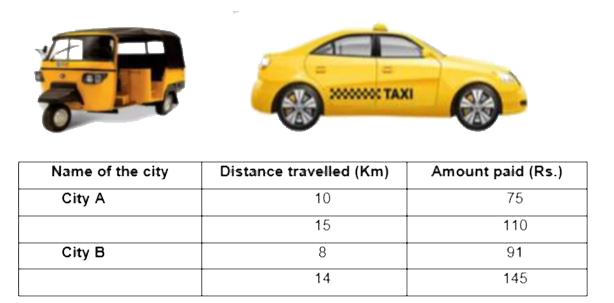
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
The graph of lines representing the conditions are: (situation 2)
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
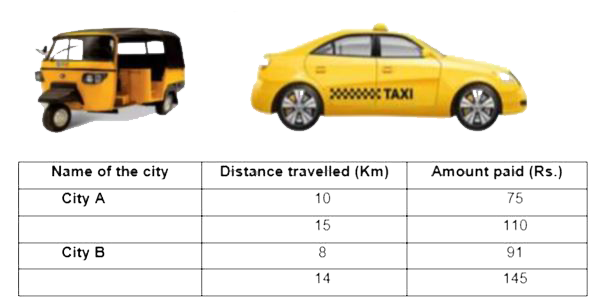
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
If the fixed charges of auto rickshaw be Rs x and the running charges be Rs y km/hr, the pair of linear equations representing the situation is
View Solution