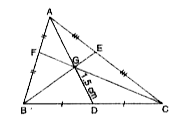
G is the centroid of ΔABC. The medians AD and BE intersect at right angles . If the lengths of AD and BE are 9 cm and 12 cm respectively , then the length of AB (in cm ) is ?
The correct Answer is:10
To solve the problem, we need to find the length of side AB in triangle ABC, given that G is the centroid, and the medians AD and BE intersect at right angles. The lengths of the medians are AD = 9 cm and BE = 12 cm.
1. Understanding the Centroid and Medians:
- The centroid (G) of a triangle divides each median into a ratio of 2:1. This means that if we denote AG as 2x and GD as x, then AD = AG + GD = 3x.
- Similarly, for median BE, we can denote BG as 2y and GE as y, giving us BE = BG + GE = 3y.
2. Setting Up the Equations:
- From the problem, we know:
- AD = 9 cm → 3x = 9 → x = 3 cm
- BE = 12 cm → 3y = 12 → y = 4 cm
3. Finding AG and BG:
- Now we can find the lengths of AG and BG:
- AG = 2x = 2(3) = 6 cm
- BG = 2y = 2(4) = 8 cm
4. Applying the Pythagorean Theorem:
- Since the medians intersect at right angles, we can apply the Pythagorean theorem in triangle AGB:
- AB² = AG² + BG²
- AB² = (6 cm)² + (8 cm)²
- AB² = 36 + 64
- AB² = 100
5. Calculating AB:
- Taking the square root to find AB:
- AB = √100 = 10 cm
Conclusion:
The length of AB is 10 cm.
- The centroid (G) of a triangle divides each median into a ratio of 2:1. This means that if we denote AG as 2x and GD as x, then AD = AG + GD = 3x.
- Similarly, for median BE, we can denote BG as 2y and GE as y, giving us BE = BG + GE = 3y.
- From the problem, we know:
- AD = 9 cm → 3x = 9 → x = 3 cm
- BE = 12 cm → 3y = 12 → y = 4 cm
- Now we can find the lengths of AG and BG:
- AG = 2x = 2(3) = 6 cm
- BG = 2y = 2(4) = 8 cm
- Since the medians intersect at right angles, we can apply the Pythagorean theorem in triangle AGB:
- AB² = AG² + BG²
- AB² = (6 cm)² + (8 cm)²
- AB² = 36 + 64
- AB² = 100
- Taking the square root to find AB:
- AB = √100 = 10 cm
The length of AB is 10 cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
G is the centroid of ΔABC. The medians AD and BE Intersect at right angles. If the lengths of AD and BE are 9 cm and 12 cm re spectively, then the length of AB (in cm) is
A9.5B10C11D10.5O is the centroid of △ABC. The medians AD and BE intersect at right angles. If the lenghts of AD and BE are 9 cm and 12 cm respectively : then the length of AB (in cm) is
A9.5B10C11D10.5ΔABC is a right angle triangle and ∠ABC=90∘. If the length of AB and BC is 12 cm and 16 cm respectively, then find the length of BD. (Where, BD⊥AC)
A6.4 cmB7.2 cmC8.4 cmD9.6 cm
The medians of a triangle ABC are 9 cm, 12 cm and 15 cm respectively. Then the area of the triangle is.
View SolutionTwo medians AD and BE of ΔABC intersect at G at right angles If AD=9cm and BE=6cm then the length of BD in cm is
View SolutionTwo medians AD and BE of ΔABC intersect at G at right angles . If AD=9cmandBE=6cm, them the length of BD (in cm) is
View SolutionG is the centroid of the triangle ABC, where AB, BC and CA are 7 cm, 24 cm and 25 cm respectively, then BG is:
G त्रिभुज ABC का केन्द्रक है जिसमें AB, BC और CA क्रमशः 7 सेमी, 24 सेमी और 25 सेमी हैं | BG का मान ज्ञात करें |
View SolutionIn ΔABC medians BE and CF intersect at G. If the straight line AGD meets BC in D in such a way that GD = 1.5 cm, then the length of AD is :
View Solution
