Find the two equation of regression line for the given data:
n=102,∑x=510,∑y=7140,∑x2=4150,∑y2=740200,∑xy=54900
Also estimate the value of y when x = 7.
The correct Answer is:Regression equation of y on x: y = 12x + 10,
Regression equation of x on y:x=0.08y−0.6;y=94
To find the two equations of regression lines for the given data, we will follow these steps:
Step 1: Calculate the Means ¯x and ¯y
Given:
- n=102
- ∑x=510
- ∑y=7140
The mean values are calculated as follows:
¯x=∑xn=510102=5
¯y=∑yn=7140102=70
Step 2: Calculate the Regression Coefficients bxy and byx
The formulas for the regression coefficients are:
bxy=∑xy−n⋅¯x⋅¯y∑y2−n⋅¯y2
byx=∑xy−n⋅¯x⋅¯y∑x2−n⋅¯x2
Given:
- ∑xy=54900
- ∑y2=740200
- ∑x2=4150
Now we can calculate bxy:
bxy=54900−102⋅5⋅70740200−102⋅702
Calculating the numerator:
54900−102⋅5⋅70=54900−35700=19200
Calculating the denominator:
740200−102⋅702=740200−102⋅4900=740200−499800=240400
Thus,
bxy=19200240400≈0.08
Now we calculate byx:
byx=54900−102⋅5⋅704150−102⋅52
Calculating the numerator (same as before):
54900−35700=19200
Calculating the denominator:
4150−102⋅52=4150−2550=1600
Thus,
byx=192001600=12
Step 3: Write the Regression Equations
The regression equations are given by:
1. y−¯y=bxy(x−¯x)
2. x−¯x=byx(y−¯y)
Substituting the values:
1. For y:
y−70=0.08(x−5)⟹y=0.08x+70−0.4⟹y=0.08x+69.6
2. For x:
x−5=12(y−70)⟹x=12y−840+5⟹x=12y−835
Step 4: Estimate the Value of y when x=7
Using the first regression equation:
y=0.08(7)+69.6=0.56+69.6=70.16
Using the second regression equation:
x=12y−835⟹7=12y−835⟹12y=842⟹y=84212≈70.17
Final Results
The two regression equations are:
1. y=0.08x+69.6
2. x=12y−835
The estimated value of y when x=7 is approximately 70.16.
- n=102
- ∑x=510
- ∑y=7140
- ∑xy=54900
- ∑y2=740200
- ∑x2=4150
2. x−¯x=byx(y−¯y)
y−70=0.08(x−5)⟹y=0.08x+70−0.4⟹y=0.08x+69.6
x−5=12(y−70)⟹x=12y−840+5⟹x=12y−835
1. y=0.08x+69.6
2. x=12y−835
Topper's Solved these Questions
Similar Questions
Find the regression coefficient of x on y from the following data:
∑x=15,∑y=15,∑y2=49,∑xy=44,n=5, Also find the value of x when y = 7.
View SolutionFind the correlation coefficient r(x,y) if :
n=10,∑x=60,∑y=60,∑x2=400,∑y2=580,∑xy=305.
View SolutionA computer while calculating the correlation coefficient between the variables x and y obtained the following results :
n=25,∑xi=125,∑yi=100,∑x2i=650,∑y2i=460,∑xiyi=508 It was however later discovered at the time of checking that it has copied down two pairs of obervations as (6,14) and (8,6) where as values were (8,12) and (6,8) .Calculate the correct correlation coefficient of x and y.
View SolutionFind the equation of tangent line to y=2x2+7 which is parallel to the line 4x−y+3=0 .
View SolutionIn a blvariate data ∑x=30,∑y=400,∑x2=196,∑xy=850andn=10. Find the regression coefficient of y on x.
View SolutionFor a bivariate date, you are given following information:
∑x=125,∑x2=1650,∑y=100,∑y2=1500,∑xy=50,n=25
Find line of regression.
View SolutionFind the line of regression of y on x from the following table :
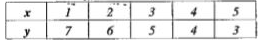
Hence estimate the value of y when x=6.
View SolutionFrom the following data find regression equation of y on x, Σx=55,Σy=88,Σx2=385,Σy2=1114,Σxy=586,n=10
View SolutionFind the equation of the tangent line to the curve y=x2−2x+7 which is parallel to the line 2x−y+9=0
View SolutionFind the equation of the tangent line to the curve y=x2−2x+7 which is perpendicular to the line 5y−15x=13 .
View Solution
