Out of the following two regression lines, find the line of regression of Y on X:3x+12y=7,3y+9x=46.
The correct Answer is:3x+2y=7
To find the line of regression of Y on X from the given regression lines, we will follow these steps:
Step 1: Write down the equations of the regression lines
The two regression lines given are:
1. 3x+12y=7 (Equation 1)
2. 3y+9x=46 (Equation 2)
Step 2: Rearrange Equation 1 to find the regression of Y on X
We will rearrange Equation 1 to express Y in terms of X.
Starting with:
3x+12y=7
Subtract 3x from both sides:
12y=7−3x
Now, divide by 12:
y=−312x+712
This simplifies to:
y=−14x+712
Step 3: Identify the regression line of Y on X
The regression line of Y on X can be expressed in the form:
y=byxx+a
where byx is the slope of the regression line.
From our rearranged equation, we can see that:
byx=−14
Step 4: Write the final answer
Thus, the line of regression of Y on X is:
y=−14x+712
The two regression lines given are:
1. 3x+12y=7 (Equation 1)
2. 3y+9x=46 (Equation 2)
We will rearrange Equation 1 to express Y in terms of X.
3x+12y=7
12y=7−3x
y=−312x+712
y=−14x+712
The regression line of Y on X can be expressed in the form:
y=byxx+a
where byx is the slope of the regression line.
byx=−14
Thus, the line of regression of Y on X is:
y=−14x+712
Topper's Solved these Questions
Similar Questions
Two regression lines are represented by 4y+10x=9and6x+3y=4. Find the line of regression of y on x.
View SolutionGiven two regression lines 4x+3y+7=0and3x+4y+8=0, determine.
The regression line of x on y
View SolutionGiven two regression lines 4x+3y+7=0and3x+4y+8=0, determine.
The regression line of y on x
View SolutionFind r(X,Y) from the two regression equation 3x=y and 4y=3x.
View SolutionTwo regression lines are represented by 2x+3y−10=0 and 4x+y−5=0
Find the line of regression of y on x.
View SolutionFind the equations of two lines of regression for the data:
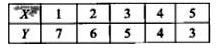
And hence find an estimate of variable Y for X = 3.5 from the appropriate line of regression.
View SolutionFor the lines of regression 4x−2y=4and2x−3y+6=0, find the mean of 'x' and the mean of 'y'.
View SolutionFind the equations of the two lines of regression for the following observations : (3, 6), (4, 5), (5, 4), (6,3), (7,2). Find the estimation of y for x = 2.5.
View SolutionFind the regression coefficient byxandbxy when the lines of regression are
6x−5y+10=0,10x−3y−28=0.
View SolutionIn a bivariate distribution, it was found σx=3, the regression line of Y on X is 8x−10y+66=0 while regression line of X on Y is 40x−18y−214=0. Calculate r(x,y)
View Solution