Given below are the heights (in cm) of 11 boys of a class :
149, 142, 154, 146, 143, 152, 148, 132, 140, 139, 128
Arrange the above data in an ascending order and find
(i) the height of the tallest boy
(ii) the height of the shortest boy
(iii) the range of the given data
(iv) the mean height
To solve the problem step by step, we will follow these instructions:
Step 1: Arrange the Data in Ascending Order
We are given the heights of 11 boys:
149, 142, 154, 146, 143, 152, 148, 132, 140, 139, 128.
First, we need to arrange these heights in ascending order (from smallest to largest).
Ascending Order:
1. 128
2. 132
3. 139
4. 140
5. 142
6. 143
7. 146
8. 148
9. 149
10. 152
11. 154
Step 2: Find the Height of the Tallest Boy
The tallest boy's height is the maximum value in the arranged data.
Tallest Boy's Height:
- The tallest boy is 154 cm.
Step 3: Find the Height of the Shortest Boy
The shortest boy's height is the minimum value in the arranged data.
Shortest Boy's Height:
- The shortest boy is 128 cm.
Step 4: Calculate the Range of the Given Data
The range is calculated by subtracting the shortest height from the tallest height.
Range Calculation:
- Range = Tallest Height - Shortest Height
- Range = 154 cm - 128 cm = 26 cm.
Step 5: Calculate the Mean Height
To find the mean height, we need to sum all the heights and then divide by the number of boys.
Sum of Heights:
- Sum = 128 + 132 + 139 + 140 + 142 + 143 + 146 + 148 + 149 + 152 + 154 = 1573 cm.
Mean Calculation:
- Mean Height = Total Sum of Heights / Number of Boys
- Mean Height = 1573 cm / 11 = 143 cm.
Final Answers:
(i) The height of the tallest boy: 154 cm
(ii) The height of the shortest boy: 128 cm
(iii) The range of the given data: 26 cm
(iv) The mean height: 143 cm
---
We are given the heights of 11 boys:
149, 142, 154, 146, 143, 152, 148, 132, 140, 139, 128.
1. 128
2. 132
3. 139
4. 140
5. 142
6. 143
7. 146
8. 148
9. 149
10. 152
11. 154
The tallest boy's height is the maximum value in the arranged data.
- The tallest boy is 154 cm.
The shortest boy's height is the minimum value in the arranged data.
- The shortest boy is 128 cm.
The range is calculated by subtracting the shortest height from the tallest height.
- Range = Tallest Height - Shortest Height
- Range = 154 cm - 128 cm = 26 cm.
To find the mean height, we need to sum all the heights and then divide by the number of boys.
- Sum = 128 + 132 + 139 + 140 + 142 + 143 + 146 + 148 + 149 + 152 + 154 = 1573 cm.
- Mean Height = Total Sum of Heights / Number of Boys
- Mean Height = 1573 cm / 11 = 143 cm.
(i) The height of the tallest boy: 154 cm
(ii) The height of the shortest boy: 128 cm
(iii) The range of the given data: 26 cm
(iv) The mean height: 143 cm
Topper's Solved these Questions
- DATA HANDLING BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24A
18 Videos
- DATA HANDLING BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24B
10 Videos
- DATA HANDLING AND MEASURES OF CENTRAL TENDENCYBOOK - ICSECHAPTER - DATA HANDLING AND MEASURES OF CENTRAL TENDENCYEXERCISE - TRY THIS
19 Videos
- DECIMALS BOOK - ICSECHAPTER - DECIMALS EXERCISE - TRY THIS
41 Videos
Similar Questions
The heights of 10 girls were measured in cm and the results are as follows: 135, 150, 139, 128, 151, 132, 146, 149, 143, 141.
(i) What is the height of the tallest girl?
(ii) What is the height of the shortest girl?
(iii) What is the range of the data?
View SolutionThe heights of 10 girls were measured in cm and the results are as follows:135, 150, 139, 128, 151, 132, 146, 149, 143, 141.(i) What is the height of the tallest girl? (ii) What is the height of the shortest girl?(iii) What is the range of the data? (iv) What is the mean height of the girls?(v) How many girls have heights more than the mean height
View SolutionThe heights of 6 boys are 146 cm, 154 cm, 153 cm, 160 cm, 157 cm and 160 cm. Find their mean height.
View SolutionThe heights (in cm) of 35 students of a class are given below :
Height (in cm)157152153154155156Number of students743597
Find the median height.
View SolutionGiven below is the frequency distribution of the heights of 50 students of a class: Class Interval:, 140-145, 145-150, 150-155, 155-160, 160-165 Frequency:, 8, 12, 18, 10, 5 Draw a histogram representing the above data.
View SolutionA survery regrding the heights (in cm ) of 50 girls of a class conducted and the following data was obatained.
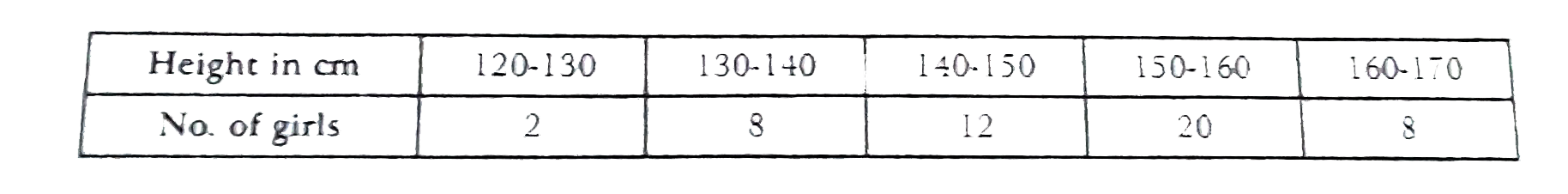
Find the mean of above data.
View SolutionGiven below is a bar graph showing the heights of five mountain peaks.
Read the bar graph carefully and answer the following questions:
(i) Which is the highest peak and what is its height?
(ii) What is the ratio of the heights of the highest peak and the next highest peak?
(iii) Arrange the heights of the given peaks in descending order.
View SolutionUse Graph paper for this question.
A survey regarding height ( in cm) of 60 boys belonging to Class 10 of a school was conducted. The following data was recorded.
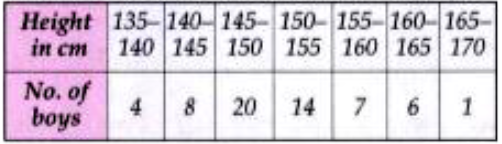
Taking 2 cm = height of 10 cm along one axis and 2 cm = 10 boys along the other axis draw an ogive of the above distribution. Use the graph to estimate the following :
(i) the median
View SolutionUse Graph paper for this question.
A survey regarding height ( in cm) of 60 boys belonging to Class 10 of a school was conducted. The following data was recorded.
Taking 2 cm = height of 10 cm along one axis and 2 cm = 10 boys along the other axis draw an ogive of the above distribution. Use the graph to estimate the following :
(iii) if above 158 cm is considered as the tall boys of the class. Find the number of boys in the class who are tall.
View SolutionThe following table shows the heights of 50 students of a class :
Height (in cm)152148150153149151147Number of students834712106
Find the mean and the median.
Using empirical formula, calculate its mode.
View Solution
