A factory manufactures two types of screws, A and B, each type requiring the use of two machines, an automatic and a hand-operated. It takes 4 minutes on the automatic and 6 minutes on hand-operated machines to manufacture a packet of screws 'A', while it takes 6 minutes on the automatic and 3 minutes on the hand-operated machines to manufacture a packet of screws 'B'. Each machine is available for at the most 4 hours on any day. The manufacturer can sell a packet of screws 'A' at a profit of 70 paise and screws 'B' at a profit of Rs. 1. Assuming that he can sell all the screws he manufactures, how many packets of each type should the factory owner produce in a day in order to maximise his profit ? Formulate the above L.P.P. and solve it graphically and find the maximum profit.
The correct Answer is:30 packets of type-A and 20 packets of type B, Maximum profit = Rs. 41
To solve the problem of maximizing the profit from manufacturing screws A and B, we can follow these steps:
Step 1: Define Variables
Let:
- x = number of packets of screw A produced
- y = number of packets of screw B produced
Step 2: Formulate the Objective Function
The profit from each type of screw is given as:
- Profit from screw A = 0.7 rupees per packet
- Profit from screw B = 1 rupee per packet
Thus, the total profit Z can be expressed as:
Z=0.7x+1y
Step 3: Set Up the Constraints
Each machine is available for 4 hours a day, which is equivalent to 240 minutes. The time taken for each type of screw is as follows:
- For screw A:
- Automatic machine: 4 minutes
- Hand-operated machine: 6 minutes
- For screw B:
- Automatic machine: 6 minutes
- Hand-operated machine: 3 minutes
From this, we can derive the following constraints:
1. Time on the automatic machine:
4x+6y≤240(1)
2. Time on the hand-operated machine:
6x+3y≤240(2)
3. Non-negativity constraints:
x≥0,y≥0(3)
Step 4: Convert Inequalities to Equations
To graphically solve the inequalities, we convert them to equations:
1. 4x+6y=240
2. 6x+3y=240
Step 5: Find Intercepts for Graphing
For the first equation 4x+6y=240:
- If x=0:
6y=240⇒y=40(0,40)
- If y=0:
4x=240⇒x=60(60,0)
For the second equation 6x+3y=240:
- If x=0:
3y=240⇒y=80(0,80)
- If y=0:
6x=240⇒x=40(40,0)
Step 6: Plot the Lines and Identify Feasible Region
Plot the lines on a graph:
- Line from (0, 40) to (60, 0) for 4x+6y=240
- Line from (0, 80) to (40, 0) for 6x+3y=240
The feasible region is where the two lines intersect and is bounded by the axes.
Step 7: Find Intersection Point
To find the intersection of the two lines:
1. From 4x+6y=240 and 6x+3y=240:
- Multiply the second equation by 2:
12x+6y=480
- Subtract the first equation from this:
12x+6y−(4x+6y)=480−240⇒8x=240⇒x=30
2. Substitute x=30 back into the first equation:
4(30)+6y=240⇒120+6y=240⇒6y=120⇒y=20
Thus, the intersection point is (30,20).
Step 8: Evaluate Profit at Corner Points
The corner points of the feasible region are:
1. (0,0): Z=0.7(0)+1(0)=0
2. (40,0): Z=0.7(40)+1(0)=28
3. (30,20): Z=0.7(30)+1(20)=21+20=41
4. (0,40): Z=0.7(0)+1(40)=40
Step 9: Determine Maximum Profit
The maximum profit occurs at the point (30,20):
- Number of packets of screw A = 30
- Number of packets of screw B = 20
- Maximum Profit = 41 rupees
Final Answer
The factory owner should produce 30 packets of screw A and 20 packets of screw B to maximize profit, resulting in a maximum profit of 41 rupees.
Let:
- x = number of packets of screw A produced
- y = number of packets of screw B produced
The profit from each type of screw is given as:
- Profit from screw A = 0.7 rupees per packet
- Profit from screw B = 1 rupee per packet
Z=0.7x+1y
Each machine is available for 4 hours a day, which is equivalent to 240 minutes. The time taken for each type of screw is as follows:
- For screw A:
- Automatic machine: 4 minutes
- Hand-operated machine: 6 minutes
- For screw B:
- Automatic machine: 6 minutes
- Hand-operated machine: 3 minutes
4x+6y≤240(1)
6x+3y≤240(2)
x≥0,y≥0(3)
To graphically solve the inequalities, we convert them to equations:
2. 6x+3y=240
For the first equation 4x+6y=240:
- If x=0:
6y=240⇒y=40(0,40)
- If y=0:
4x=240⇒x=60(60,0)
- If x=0:
3y=240⇒y=80(0,80)
- If y=0:
6x=240⇒x=40(40,0)
Plot the lines on a graph:
- Line from (0, 40) to (60, 0) for 4x+6y=240
- Line from (0, 80) to (40, 0) for 6x+3y=240
To find the intersection of the two lines:
1. From 4x+6y=240 and 6x+3y=240:
- Multiply the second equation by 2:
12x+6y=480
- Subtract the first equation from this:
12x+6y−(4x+6y)=480−240⇒8x=240⇒x=30
2. Substitute x=30 back into the first equation:
4(30)+6y=240⇒120+6y=240⇒6y=120⇒y=20
Thus, the intersection point is (30,20).
The corner points of the feasible region are:
1. (0,0): Z=0.7(0)+1(0)=0
2. (40,0): Z=0.7(40)+1(0)=28
3. (30,20): Z=0.7(30)+1(20)=21+20=41
4. (0,40): Z=0.7(0)+1(40)=40
The maximum profit occurs at the point (30,20):
- Number of packets of screw A = 30
- Number of packets of screw B = 20
- Maximum Profit = 41 rupees
The factory owner should produce 30 packets of screw A and 20 packets of screw B to maximize profit, resulting in a maximum profit of 41 rupees.
Topper's Solved these Questions
Similar Questions
A factory manufactures two types of screws, A and B. Each type of screw requires the use of two machines, an automatic and a hand operated. It takes 4 minutes on the automatic and 6 minutes on hand operated machines to manufacture a package of screws A, while it takes 6 minutes on automatic and 3 minutes on the hand operated machines to manufacture a package of screws B. Each machine is available for at the most 4 hours on any day. The manufacturer can sell a package of screws A at a profit of 70 paise and screws B at a profit of Rs 1. Assuming that he can sell all the screws he manufactures, how many packages of each type should the factory owner produce in a day in order to maximise his profit? Formulate the above LPP and solve it graphically and determine the maximum profit.
View SolutionA factory manufactures two types of screws, A and B. Each type of screw requires the use of two machines, an automatic and a hand operated. It takes 4 minutes on the automatic and 6 minutes on hand operated machines to manufacture a package of screws A, while it takes 6 minutes on automatic and 3 minutes on the hand operated machines to manufacture a package of screws B. Each machine is available for at the most 4 hours on any day. The manufacturer can sell a package of screws A at a profit of 70 paise and screws B at a profit of Rs 1. Assuming that he can sell all the screws he manufactures, how many packages of each type should the factory owner produce in a day in order to maximise his profit? Formulate the above LPP and solve it graphically and determine the maximum profit.
View SolutionA cottage industry manufactures pedestal lamps and wooden shades, each requiring the use of a grinding/cutting machine and a sprayer. It takes 2 hours on the grinding/cutting machine and 3 hours on the sprayer to manufacture a pedestal lamp. It takes 1 hour on the grinding/cutting machine and 2 hours on the sprayer to manufacture a shade. On any day, the sprayer is available for at the most 20 hours and the grinding/cutting machine for at the most 12 hours. The profit from the sale of a lamp is Rs. 25 and that from a shade is Rs. 15. Assuming that the manufacturer can sell all the lamps and shades that he produces, how should he schedule his daily production in order to maximise his profit. Formulate an LPP and solve it graphically.
View SolutionA cottage industry manufactures pedestal lamps and wooden shades, each requiring the use of grinding/cutting machine and a sprayer. It takes 2 hours on the grinding/cutting machine and 3 hours on the sprayer to manufacture a pedestal lamp. It takes one hour on the grinding/cutting machine and 2 hours on the sprayer to manufacture a shade. On any day, the sprayer is available for at the most 20 hours and the grinding/cutting machine for at the most 12 hours. The profit from the sale of a lamp is Rs. 5 and that from a shade is Rs. 3. Assuming that the manufacturer can sell all the lamps and shades that he produces, how should he schedule his daily production in order to maximise his profit? Make an L.P.P. and solve it graphically.
View SolutionA cottage industry manufactures pedestal lamps and wooden shades, each requiring the use of grinding/cutting machine and a sprayer. It takes 2 hours on the grinding/cutting machine and 3 hours on the sprayer to manufacture a pedestal lamp. It takes one hour on the grinding/cutting machine and 2 hours on the sprayer to manufacture a shade. On any day, the sprayer is available for at the most 20 hours and the grinding/cutting machine for at the most 12 hours. The profit from the sale of a lamp is Rs. 5 and that from a shade is Rs. 3. Assuming that the manufacturer can sell all the lamps and shades that he produces, how should he schedule his daily production in order to maximise his profit? Make an L.P.P. and solve it graphically.
View SolutionThe type of simple machine a door knob is
View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the tune (in minutes) required for each toy on the machines is given below:
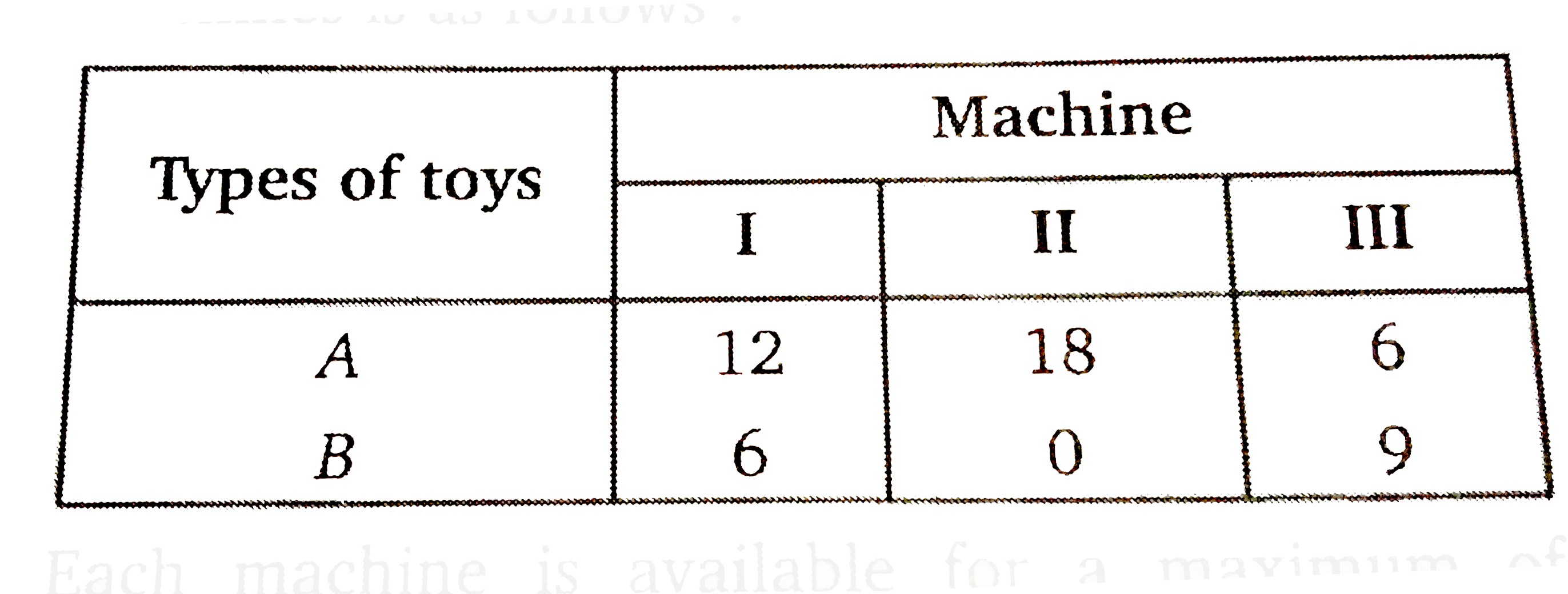 Each machine is available for a
maximum of 6 hours per day. If the profit
Each machine is available for a
maximum of 6 hours per day. If the profit
View SolutionA company manufactures two types of screws A and B. all the screws have to pass through a threading machine and a slotting machine. A box of type A screw requires 2min on the threading machine and 3 min on the slotting machine. A box of type B screw requires 8 min on the threading machine and 2 min on the slotting machine. In a week each machine is availbale for 60h. On selling these screws, the company gets a profit of 100 box on type A screw and 170 per box on type B screws. Formulate this problem as a LPP given that the objective is to maximise profit.
View SolutionA manufacturer produces nuts and bolts. It takes 2 hours work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 2 hours on machine B to produce a package of bolts. He earns a profit of Rs. 24 per package on nuts and Rs. 18 per package on bolts. How many packages of each should be produced each day so as to maximize his profit, if he operates his machines for at the most 10 hours a day. Make an L.P.P. from above and solve it graphically ?
View SolutionA cottage industry manufactures pedestal lamps and wooden shades.Both the products require machine time as well as craftsman time in the making.The number of hours required for producing 1 unit of each and the corresponding profit is given in the following table:
In a day,the factory has availability of not more than 42 hours of machine time and 24 hours of craftsman time.
Assuming that all items manufactured are sold,how should the manufacturer schedule his daily production in order to maximise the profit?Formulate it as an LPP and solve it graphically
View Solution