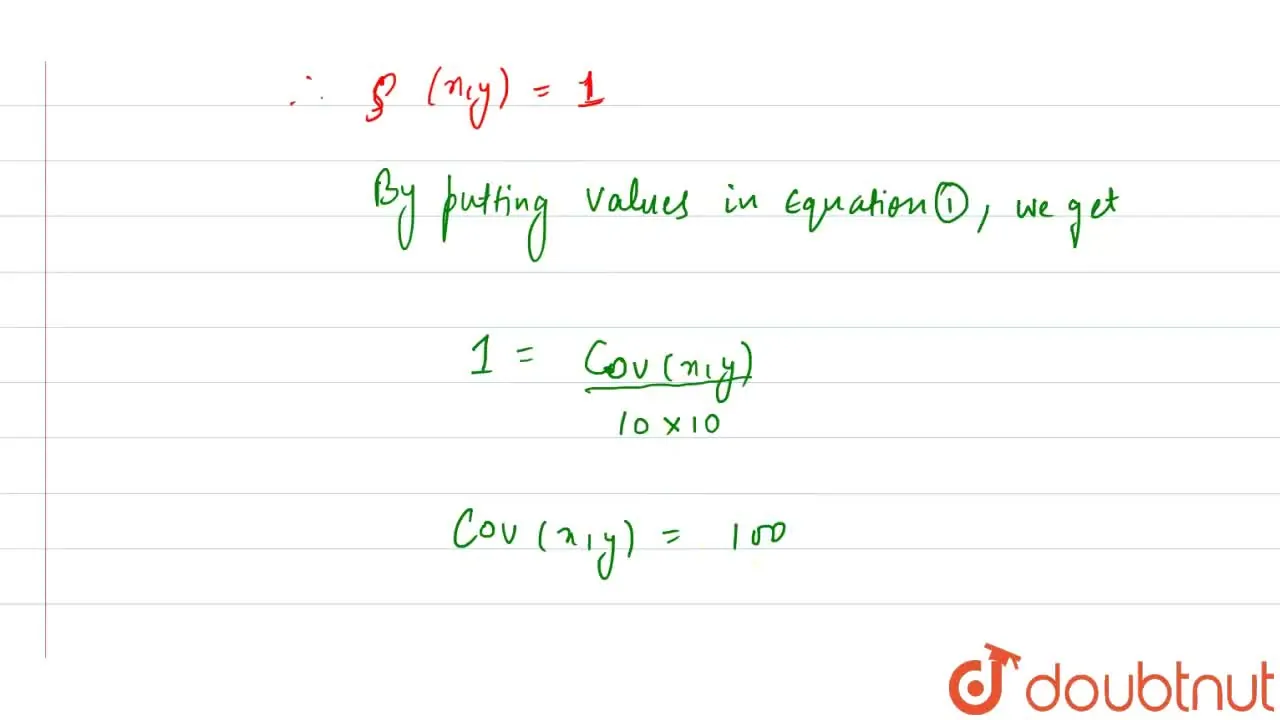
If σx=10, σy=10, then the value of Cov(x,y) is
A
100
B
√10
C
10
D
0
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video, text & image solution for If sigma _x =10 , sigma _y =10 , then the value of Cov(x,y) is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
If y=log10x, then the value of dydx is
View SolutionIf σx=3,σy=4, and bxy=13, then the value of r is
View SolutionIf Cov(x,y) = -2, Σy=30,Σx=25andΣxy=140 , find the number of observations .
View SolutionIf σx=5,r=−12andbyx=−27 , then the value of σy is:
View SolutionIn naphthalene number of σbonds=x and number of πbonds=ythen the value of (x−y)
View SolutionIf 8x−2y=10and3y−9x=12, then what is the value of y−x ?
View SolutionIf Σ2ni=1sin−1xi=nπ , then find the value of Σ2ni=1xi.
View SolutionIf 3x+5y=15andx−2y=10, what is the value of 2x+7y?
View SolutionIf x=y and x+y=10, then 2x+y=
View SolutionIf Σ2ni=1cos−1xi=0,then find the value of Σ2ni=1xi
View Solution