Calculate the variance and standard deviation of the observations : 11, 12, 13, . . . .. 20.
The correct Answer is:8.25;2.87
To calculate the variance and standard deviation of the observations 11, 12, 13, ..., 20, we will follow these steps:
Step 1: List the observations
The observations given are:
11,12,13,14,15,16,17,18,19,20
Step 2: Calculate the mean (¯x)
The mean is calculated using the formula:
¯x=∑xin
where n is the number of observations.
Calculating the sum:
∑xi=11+12+13+14+15+16+17+18+19+20=155
The number of observations n=10.
Now, calculating the mean:
¯x=15510=15.5
Step 3: Calculate xi−¯x for each observation
Now we will calculate xi−¯x for each observation:
- For 11: 11−15.5=−4.5
- For 12: 12−15.5=−3.5
- For 13: 13−15.5=−2.5
- For 14: 14−15.5=−1.5
- For 15: 15−15.5=−0.5
- For 16: 16−15.5=0.5
- For 17: 17−15.5=1.5
- For 18: 18−15.5=2.5
- For 19: 19−15.5=3.5
- For 20: 20−15.5=4.5
Step 4: Calculate (xi−¯x)2
Now we will square each of the results from Step 3:
- For 11: (−4.5)2=20.25
- For 12: (−3.5)2=12.25
- For 13: (−2.5)2=6.25
- For 14: (−1.5)2=2.25
- For 15: (−0.5)2=0.25
- For 16: (0.5)2=0.25
- For 17: (1.5)2=2.25
- For 18: (2.5)2=6.25
- For 19: (3.5)2=12.25
- For 20: (4.5)2=20.25
Step 5: Calculate the sum of squares
Now we will sum these squared differences:
∑(xi−¯x)2=20.25+12.25+6.25+2.25+0.25+0.25+2.25+6.25+12.25+20.25=112.5
Step 6: Calculate the variance (σ2)
The variance is given by:
σ2=∑(xi−¯x)2n
Substituting the values:
σ2=112.510=11.25
Step 7: Calculate the standard deviation (σ)
The standard deviation is the square root of the variance:
σ=√σ2=√11.25≈3.3541
Final Results
- Variance: 11.25
- Standard Deviation: 3.3541
The observations given are:
11,12,13,14,15,16,17,18,19,20
The mean is calculated using the formula:
¯x=∑xin
where n is the number of observations.
∑xi=11+12+13+14+15+16+17+18+19+20=155
The number of observations n=10.
¯x=15510=15.5
Now we will calculate xi−¯x for each observation:
- For 11: 11−15.5=−4.5
- For 12: 12−15.5=−3.5
- For 13: 13−15.5=−2.5
- For 14: 14−15.5=−1.5
- For 15: 15−15.5=−0.5
- For 16: 16−15.5=0.5
- For 17: 17−15.5=1.5
- For 18: 18−15.5=2.5
- For 19: 19−15.5=3.5
- For 20: 20−15.5=4.5
Now we will square each of the results from Step 3:
- For 11: (−4.5)2=20.25
- For 12: (−3.5)2=12.25
- For 13: (−2.5)2=6.25
- For 14: (−1.5)2=2.25
- For 15: (−0.5)2=0.25
- For 16: (0.5)2=0.25
- For 17: (1.5)2=2.25
- For 18: (2.5)2=6.25
- For 19: (3.5)2=12.25
- For 20: (4.5)2=20.25
Now we will sum these squared differences:
∑(xi−¯x)2=20.25+12.25+6.25+2.25+0.25+0.25+2.25+6.25+12.25+20.25=112.5
The variance is given by:
σ2=∑(xi−¯x)2n
Substituting the values:
σ2=112.510=11.25
The standard deviation is the square root of the variance:
σ=√σ2=√11.25≈3.3541
- Variance: 11.25
- Standard Deviation: 3.3541
Topper's Solved these Questions
- MODEL TEST PAPER - 9 BOOK - ICSECHAPTER - MODEL TEST PAPER - 9 EXERCISE - SECTION - B
10 Videos
- MODEL TEST PAPER - 9 BOOK - ICSECHAPTER - MODEL TEST PAPER - 9 EXERCISE - SECTION - C
9 Videos
- MODEL TEST PAPER - 20 BOOK - ICSECHAPTER - MODEL TEST PAPER - 20 EXERCISE - SECTION - C
10 Videos
- MODEL TEST PAPER -11BOOK - ICSECHAPTER - MODEL TEST PAPER -11EXERCISE - Sections - C
10 Videos
Similar Questions
Calculate variance and standard deviation of the following data : 10, 12, 8, 14, 16
View SolutionFind the variance and standard deviation of the following data:

View SolutionThe standard deviation of the observations 22, 26, 28, 20, 24, 30 is
View SolutionCalculate variance and standard deviation for the following distribution.
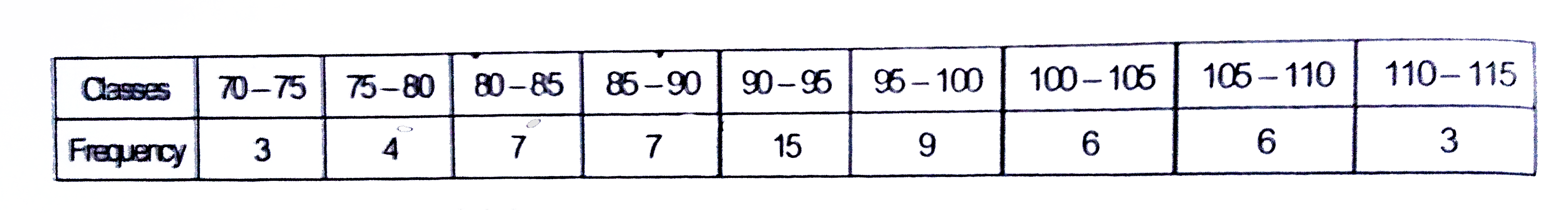
View SolutionCalculate the standard deviation of the following data:
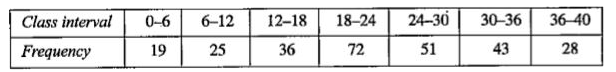
View SolutionCalculate the standard deviation of the following data:

View SolutionCalculate the mean, variance and standard devation for the following data :
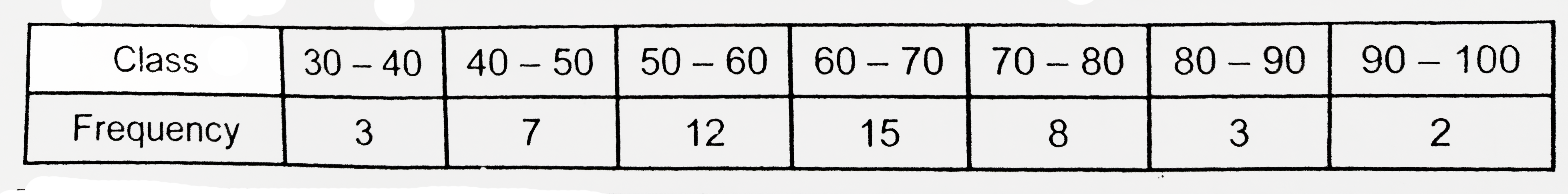
View SolutionFind the variance and standard deviation from the following data:

View SolutionFind the variance and standard deviation of the following distribution

View SolutionCalculate the standard deviation of the following distribution:

View Solution
