Find the median and upper quartile for the following data : 9,5,7,11,13,17,15.
The correct Answer is:11;15
To find the median and upper quartile for the given data set: 9, 5, 7, 11, 13, 17, 15, we will follow these steps:
Step 1: Arrange the data in ascending order.
First, we need to sort the data from smallest to largest.
Data: 9, 5, 7, 11, 13, 17, 15
Arranged Data: 5, 7, 9, 11, 13, 15, 17
Step 2: Find the median.
The median is the middle value of a data set. Since we have 7 observations (which is an odd number), the median will be the value at the position given by (n + 1) / 2, where n is the number of observations.
1. Count the number of observations (n):
n = 7
2. Calculate the position of the median:
Position = (n + 1) / 2 = (7 + 1) / 2 = 8 / 2 = 4
3. Locate the 4th value in the arranged data:
The 4th value in the arranged data (5, 7, 9, 11, 13, 15, 17) is 11.
Median = 11
Step 3: Find the upper quartile (Q3).
The upper quartile is the median of the upper half of the data. To find it, we first identify the upper half of the data.
1. Identify the upper half of the data:
The upper half consists of the values above the median. In our arranged data, the upper half is: 13, 15, 17
2. Calculate the upper quartile:
Since there are 3 values in the upper half (which is an odd number), the upper quartile will be the middle value of this subset.
- The middle value (Q3) is the 2nd value in the upper half (13, 15, 17), which is 15.
Upper Quartile (Q3) = 15
Summary of Results:
- Median = 11
- Upper Quartile (Q3) = 15
---
First, we need to sort the data from smallest to largest.
Arranged Data: 5, 7, 9, 11, 13, 15, 17
The median is the middle value of a data set. Since we have 7 observations (which is an odd number), the median will be the value at the position given by (n + 1) / 2, where n is the number of observations.
n = 7
Position = (n + 1) / 2 = (7 + 1) / 2 = 8 / 2 = 4
The 4th value in the arranged data (5, 7, 9, 11, 13, 15, 17) is 11.
The upper quartile is the median of the upper half of the data. To find it, we first identify the upper half of the data.
The upper half consists of the values above the median. In our arranged data, the upper half is: 13, 15, 17
Since there are 3 values in the upper half (which is an odd number), the upper quartile will be the middle value of this subset.
- Median = 11
- Upper Quartile (Q3) = 15
Topper's Solved these Questions
Similar Questions
Find the median for the following data :

View SolutionFind the median for the following data :
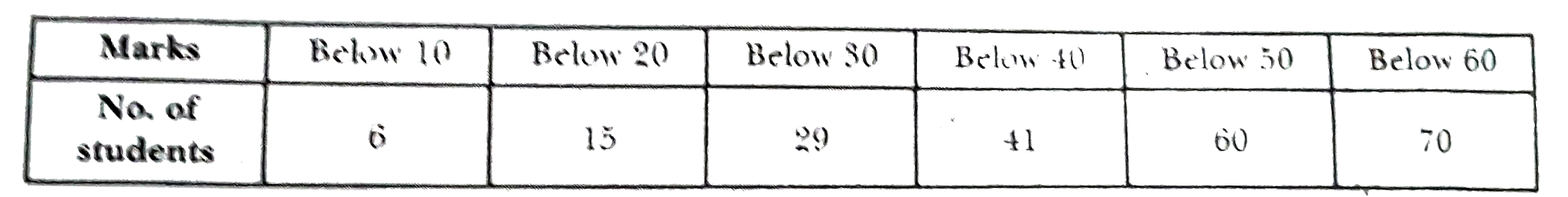
View SolutionFind the median for the following data

View SolutionFind the mean deviation about the median for the following data
13,17,16,11,13,10,16,11,18,12,17
View SolutionFind the mean deviation about the median for the following data:
11, 3, 8, 7, 5, 14, 10, 2, 9
View SolutionFind the mean deviation about the mean for the following data :12, 3, 18, 17, 4, 9, 17, 19, 20, 15, 8, 17, 2, 3, 16, 11, 3, 1, 0, 5
View SolutionFind the mean deviation about the median for the following data:4,6,9,3,10,13,2
View SolutionFind the mean deviation about the median for the following data:3,9,5,3,12,10,18,4,7,19,21.
View SolutionCalculate the mean deviation from the mean for the following data:13,17,16,14,11,13,10,16,11,18,12,17
View SolutionFind the standard deviation for the following data: (i) x: , 3, 8, 13, 18, 23 f: , 7, 10, 15, 10, 6 (ii) x: , 2, 3, 4, 5, 6, 7, 7 f: , 4, 9, 16, 14, 11, 6, 6
View Solution
