The specimen is taken in the form of 'disc' in Lee's disc method to find the thermal conductivity is a bad conductor. Why?
To understand why the specimen taken in the form of a disc in Lee's disc method to find thermal conductivity is a bad conductor, let's break down the explanation step by step.
1. Understanding Lee's Disc Method:
- Lee's disc method is an experimental technique used to measure the thermal conductivity of materials. It involves a disc-shaped specimen placed between two brass plates.
2. Setup of the Experiment:
- In this method, one brass plate is heated (let's call it Plate 1), and the other brass plate (Plate 2) is kept at a lower temperature. The specimen (the disc) is placed between these two plates.
3. Heat Transfer Mechanism:
- Heat flows from the hot brass plate (Plate 1) through the specimen to the cooler brass plate (Plate 2). The rate of heat transfer depends on the thermal conductivity of the specimen.
4. Thermal Conductivity Measurement:
- The thermal conductivity (k) of the specimen is determined by measuring the temperature difference (ΔT) between the two brass plates and knowing the heat flow rate.
5. Impact of Good Conductors:
- If the specimen is a good conductor of heat, it will allow heat to flow quickly from Plate 1 to Plate 2. This would result in a small temperature difference (ΔT) between the two plates.
6. Difficulty in Measurement:
- A small temperature difference makes it difficult to accurately measure the thermal conductivity, as the formula for calculating thermal conductivity involves ΔT. If ΔT is too small, it becomes challenging to obtain reliable results.
7. Conclusion:
- Therefore, to ensure that there is a measurable temperature difference between the two brass plates, the specimen must be a bad conductor of heat. A bad conductor will create a larger temperature gradient, allowing for a more accurate measurement of thermal conductivity.
Final Answer
The specimen taken in the form of a disc in Lee's disc method is a bad conductor because a good conductor would result in a negligible temperature difference between the two brass plates, making it difficult to measure the thermal conductivity accurately.
---
- Lee's disc method is an experimental technique used to measure the thermal conductivity of materials. It involves a disc-shaped specimen placed between two brass plates.
- In this method, one brass plate is heated (let's call it Plate 1), and the other brass plate (Plate 2) is kept at a lower temperature. The specimen (the disc) is placed between these two plates.
- Heat flows from the hot brass plate (Plate 1) through the specimen to the cooler brass plate (Plate 2). The rate of heat transfer depends on the thermal conductivity of the specimen.
- The thermal conductivity (k) of the specimen is determined by measuring the temperature difference (ΔT) between the two brass plates and knowing the heat flow rate.
- If the specimen is a good conductor of heat, it will allow heat to flow quickly from Plate 1 to Plate 2. This would result in a small temperature difference (ΔT) between the two plates.
- A small temperature difference makes it difficult to accurately measure the thermal conductivity, as the formula for calculating thermal conductivity involves ΔT. If ΔT is too small, it becomes challenging to obtain reliable results.
- Therefore, to ensure that there is a measurable temperature difference between the two brass plates, the specimen must be a bad conductor of heat. A bad conductor will create a larger temperature gradient, allowing for a more accurate measurement of thermal conductivity.
The specimen taken in the form of a disc in Lee's disc method is a bad conductor because a good conductor would result in a negligible temperature difference between the two brass plates, making it difficult to measure the thermal conductivity accurately.
Topper's Solved these Questions
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - VERY SHORT ANSWER QUESTIONS
5 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SELECTED PROBLEMS (Taken from the Previous Years ISC, AISSCE, HSSCE various States. Boards Roorke Qns & NCERT text) FROM RATE OF HEAT FLOW
6 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - LONG ANSWER QUESTIONS
5 Videos
- SELF ASSESSMENT PAPER 5BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-D
6 Videos
- THERMAL RADIATION BOOK - ICSECHAPTER - THERMAL RADIATION EXERCISE - SELECTED PROBLEMS (from WIEN.S DISPLACEMENT LAW)
14 Videos
Similar Questions
Explain Lee's disc method of finding the thermal conductivity of a bad conductor.
View SolutionDescribe an experiment to determine the coefficient of thermal conductivity of a good conductor by Searle's method.
View SolutionWhy is diamond a bad conductor of electricity but a good conductor of heat?
View SolutionA cylindrical block of length 0.4 m and area of cross-section 0.04m2 is placed coaxially on a thin metal disc of mass 0.4 kg and of the same cross - section. The upper face of the cylinder is maintained at a constant temperature of 400 K and the initial temperature of the disc is 300K. if the thermal conductivity of the material of the cylinder is 10watt/m−K and the specific heat of the material of the disc is 600J/kg−K, how long will it take for the temperature of the disc to increase to 350 K? Assume for purpose of calculation the thermal conductivity of the disc to be very high and the system to be thermally insulated except for the upper face of the cylinder.
View SolutionExplain why the covalent solids are bad conductors of electricity.
View SolutionA conductor is at T kelvin. Find the thermal speed of free electrons of the conductor .
View SolutionA thermal conductor is heated at one end. What is the theoretical value of its thermal conductivity in order that the other end also attains the same temperature?
View SolutionFind the electric field on the axis of a charged disc.
View SolutionA charge Q is uniformly distributed over the surface of non - conducting disc of radius R. The disc rotates about an axis perpendicular to its plane and passing through its centre with an angular to its plane and passing through its centre of the disc. If we keep both the amount of charge placed on the disc and its angular velocity to be constant and vary the radius of the disc then the variation of the magnetic induction at the centre of the disc will br represented by the figure:
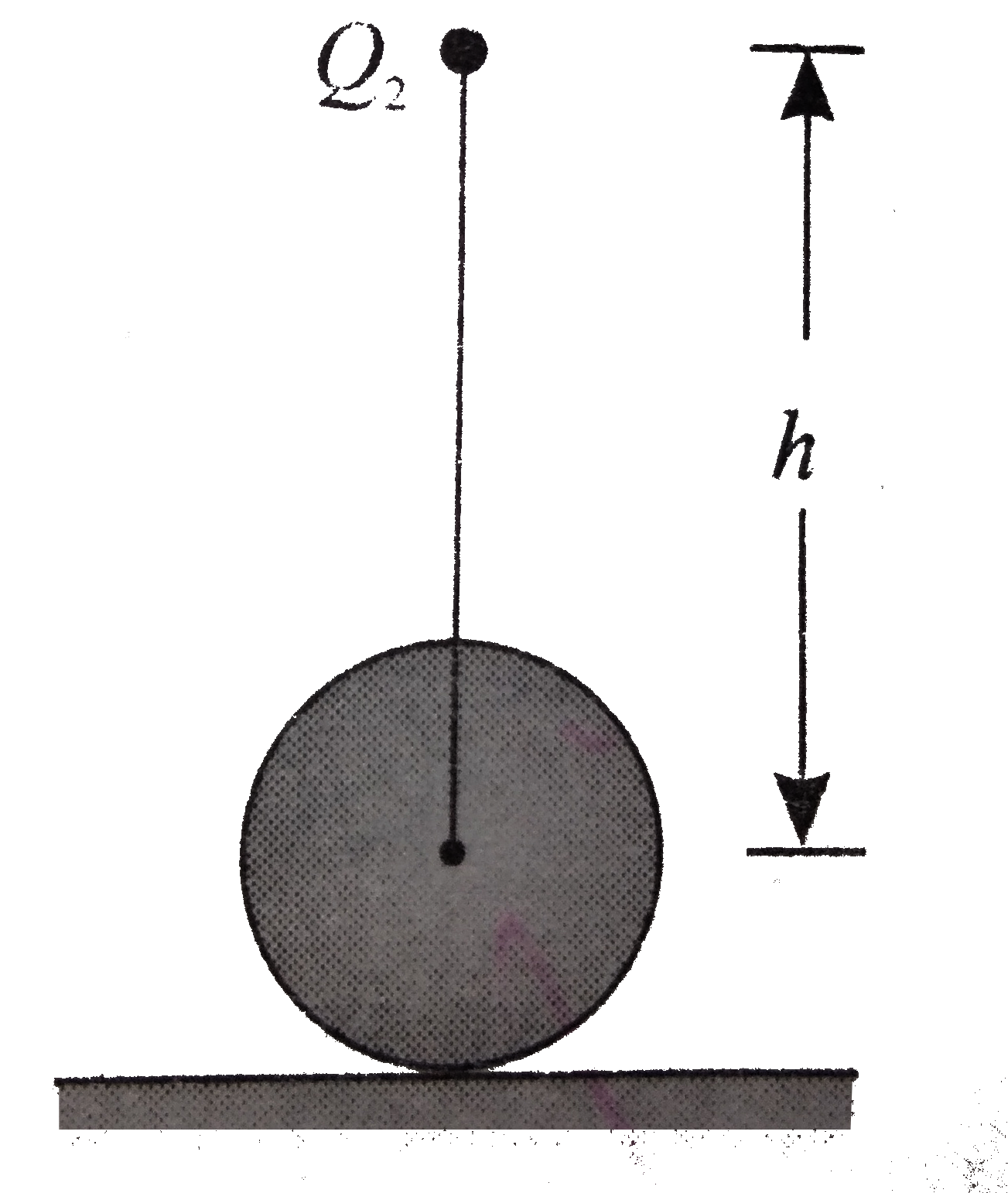
View SolutionA point charge Q1=−125μC is fixed at the center of an insulated disc of mass 1 kg. The disc rests on a rough horizontal plane. Another charge Q2=125μC is fixed verically above the center of the disc at a height h=1m. After the disc is displaced slightly in the horizontall direction (friction is sufficient to prevent slipping), find the period of oscillation of disc.
View Solution
