A cylinder of height 2x is circumscribed by a sphere of radius 2x such that the circular ends of the cylinder are two small circles on the sphere. What is the ratio of the curved surface area of the cylinder to the surface area of the sphere?
√3:4
√3:3
√3:2
√3:1
The correct Answer is:A
To solve the problem, we need to find the ratio of the curved surface area of a cylinder to the surface area of a sphere that circumscribes it. Let's break it down step by step.
Step 1: Identify the dimensions of the cylinder and sphere
- The height of the cylinder (h) is given as 2x.
- The radius of the sphere (R) is given as 2x.
Step 2: Determine the radius of the cylinder
Since the cylinder is circumscribed by the sphere, we can use the Pythagorean theorem to find the radius of the cylinder (r). The center of the cylinder is at the center of the sphere, and the height of the cylinder is divided equally above and below the center.
- The distance from the center of the sphere to the top or bottom of the cylinder is x (half of the height).
- The radius of the sphere is 2x.
Using the Pythagorean theorem:
R2=h2+r2
Substituting the known values:
(2x)2=(x)2+r2
4x2=x2+r2
r2=4x2−x2=3x2
r=√3x
Step 3: Calculate the curved surface area of the cylinder
The formula for the curved surface area (CSA) of a cylinder is:
CSA=2πrh
Substituting the values we have:
CSA=2π(√3x)(2x)=4π√3x2
Step 4: Calculate the surface area of the sphere
The formula for the surface area (SA) of a sphere is:
SA=4πR2
Substituting the radius of the sphere:
SA=4π(2x)2=4π(4x2)=16πx2
Step 5: Find the ratio of the curved surface area of the cylinder to the surface area of the sphere
Now we can find the ratio:
Ratio=CSA of cylinderSA of sphere=4π√3x216πx2
Cancelling out common terms:
Ratio=4√316=√34
Final Answer
The ratio of the curved surface area of the cylinder to the surface area of the sphere is:
√34
- The height of the cylinder (h) is given as 2x.
- The radius of the sphere (R) is given as 2x.
Since the cylinder is circumscribed by the sphere, we can use the Pythagorean theorem to find the radius of the cylinder (r). The center of the cylinder is at the center of the sphere, and the height of the cylinder is divided equally above and below the center.
- The radius of the sphere is 2x.
R2=h2+r2
Substituting the known values:
(2x)2=(x)2+r2
4x2=x2+r2
r2=4x2−x2=3x2
r=√3x
The formula for the curved surface area (CSA) of a cylinder is:
CSA=2πrh
Substituting the values we have:
CSA=2π(√3x)(2x)=4π√3x2
The formula for the surface area (SA) of a sphere is:
SA=4πR2
Substituting the radius of the sphere:
SA=4π(2x)2=4π(4x2)=16πx2
Now we can find the ratio:
Ratio=CSA of cylinderSA of sphere=4π√3x216πx2
Cancelling out common terms:
Ratio=4√316=√34
The ratio of the curved surface area of the cylinder to the surface area of the sphere is:
√34
Topper's Solved these Questions
Similar Questions
As shown in the adjacent figure, a sphere is placed in a cylinder. It touches the top, bottom and the curved surface of the cylinder. If radius of the base of the cylinder is 'r',
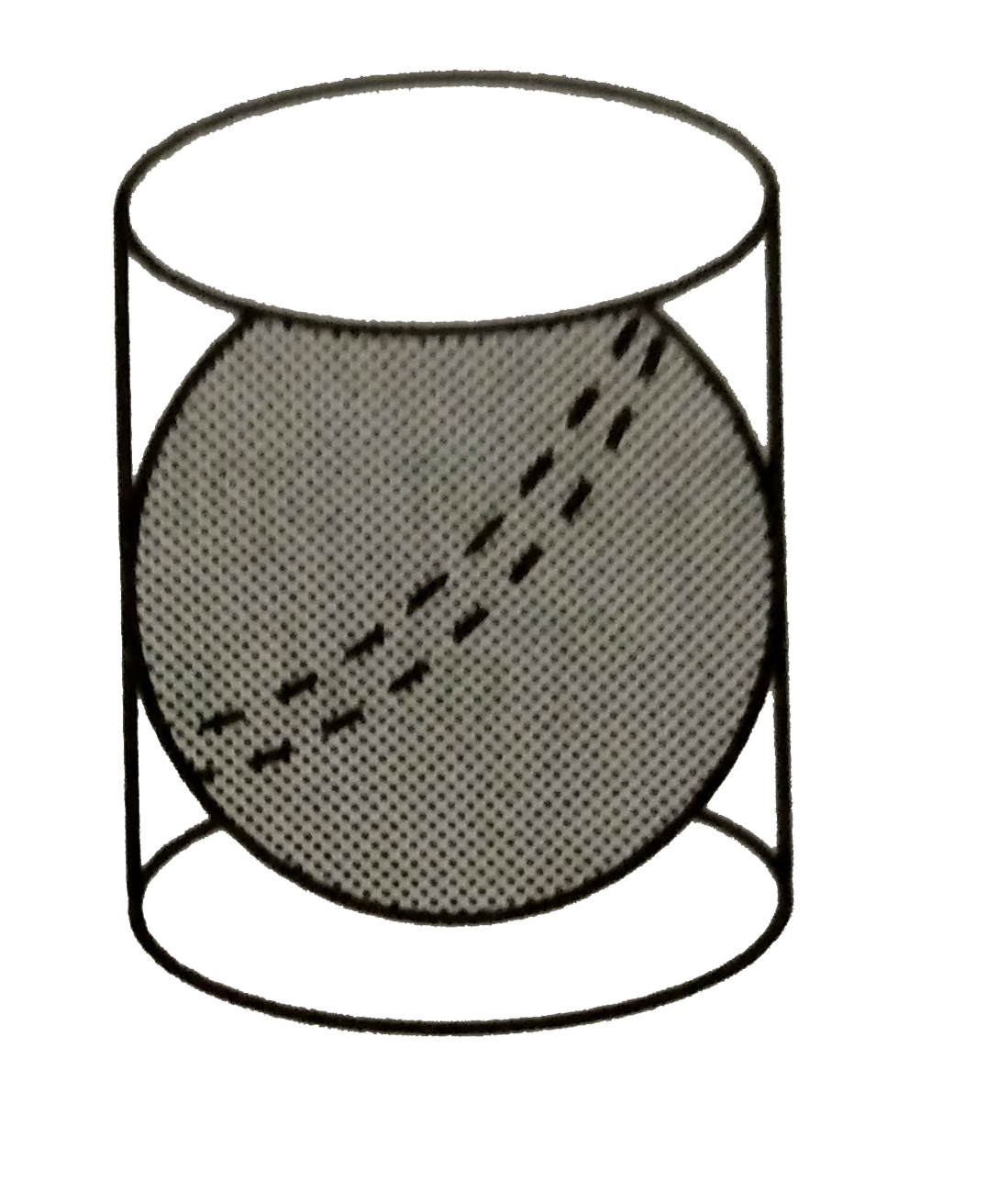
(i) what is ratio of the radii of the sphere and the cylinder?
(ii)what is the ratio of the curved surface area of the cylinder and the surface area of the sphere?
(iii) what is the ratio of the volumes of the cylinder and the sphere?
View SolutionA right circular cylinder circumscribes a sphere of radius r. Find the ratio of the surface area of the sphere to the curved surface area of the cylinder.
View Solution
Knowledge Check
A sphere and a cylinder have equal volume and equal radius. The ratio of the curved surface area of the cylinder to that of the sphere is
A4:3B2:3C3:2D3:4The ratio of the surface area of a sphere and the curved surface area of the cylinder circumscribing the sphere is
A1:2B1:1C2:1D2:3The ratio of heights of two cylinders is 3:2 and the ratio of their radii is 6:7 . What is the ratio of their curved surface areas ?
A9:7B1:1C7:9D7:4
Prove that the surface area of a sphere is equal to the curved surface area of the circumscribed cylinder.
View SolutionA sphere and the base of a cylinder have equal radii. The diameter of the sphere is equal to the height of the cylinder. The ratio of the curved surface are of the cylinder and surface area of the sphere is __________.
View SolutionA sphere just fits in a cylindrical vessel and the height of the cylindrical vessel is the same as the height of the spere . Show that the curved surface area of the cylinder is the same as the curved surface area of the sphere .
View SolutionIf radius is r and height is h, then find curved surface area of cylinder.
View SolutionThe ratio of heights of two cylinders is 3:2 and the ratio of their radii is 6:7 . What is the ratio of their curved surface areas ?
View Solution
