For a random variable X, E(X) = 3 and E(X2)=11 .Then variance of X is
8
5
2
1
The correct Answer is:C
To find the variance of the random variable X, we can use the formula for variance:
Variance(X)=E(X2)−(E(X))2
Step 1: Identify the given values
We are given:
- E(X)=3
- E(X2)=11
Step 2: Calculate (E(X))2
Now, we need to calculate (E(X))2:
(E(X))2=(3)2=9
Step 3: Substitute values into the variance formula
Now we can substitute the values into the variance formula:
Variance(X)=E(X2)−(E(X))2=11−9
Step 4: Perform the subtraction
Now, perform the subtraction:
Variance(X)=11−9=2
Conclusion
Thus, the variance of X is:
Variance(X)=2
Final Answer
The variance of X is 2.
---
We are given:
- E(X)=3
- E(X2)=11
Now, we need to calculate (E(X))2:
(E(X))2=(3)2=9
Now we can substitute the values into the variance formula:
Variance(X)=E(X2)−(E(X))2=11−9
Now, perform the subtraction:
Variance(X)=11−9=2
Thus, the variance of X is:
Variance(X)=2
The variance of X is 2.
Topper's Solved these Questions
Similar Questions
The probability distribution of a random variable X is given below.

Find k, mean and variance of X
View SolutionConsider the probability distribution of a random variable X

Calculate
(i) V(X2) (ii) Variance of X.
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View SolutionA random variable X has the distribution.
Then , variance of the distribution, is
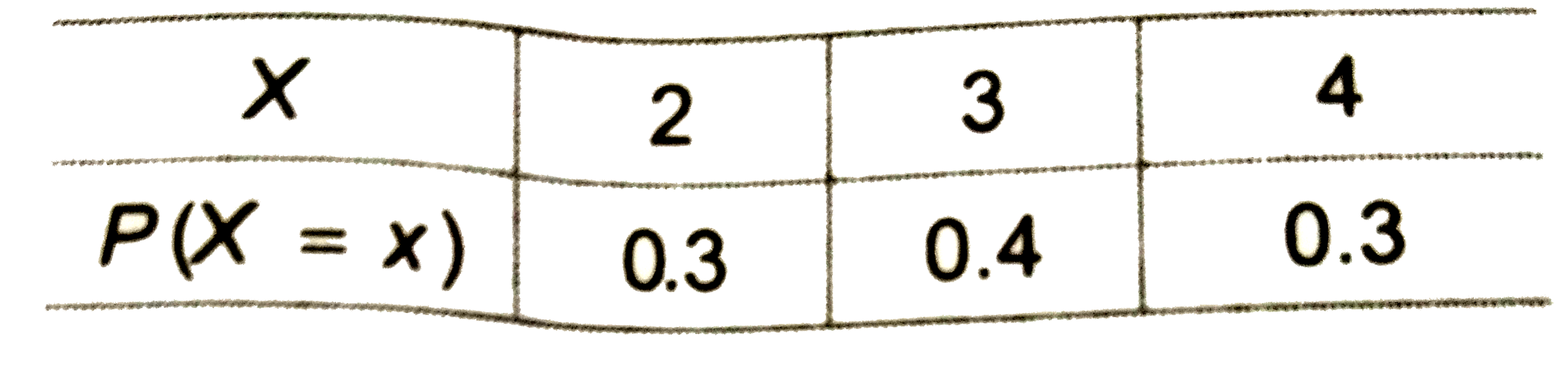
View SolutionThe probability distribution of a discrete random variable x is given as under
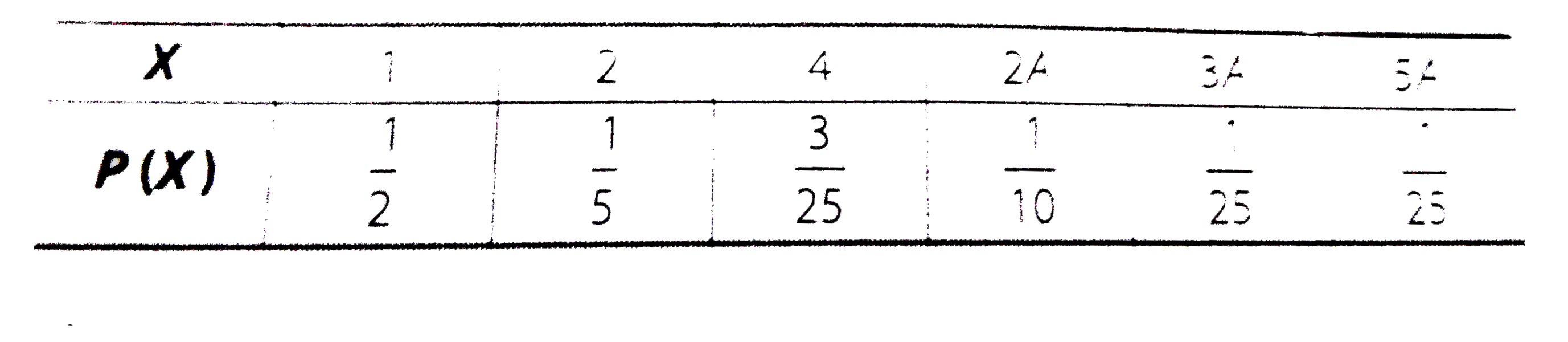
Calculate
(i) the value of A, if E(X)=2.94.
(ii) variance of X.
View SolutionProbability distribhution of a random variable X is given by:

Find k, hence find the mean and variance of the distributions.
View SolutionLet X be the discrete random variable. Then the variance of X is (a). E(X2) (b). E(X2)+(E(X))2 (c). E(X2)−(E(X))2 (d). √E(X2)−(E(X))2
View SolutionA random variable X has the following distribution .

The mean and variance of x are
View SolutionLet a random variable X have a binomial distribution with mean 8 and variance r. If P(X≤2)=k216, then k is equal to
View Solution
