कथन - I : ∫3−31xcosec98(x−1x)dx=0
क्योकि
कथन - II : ∫a−af(x)dx=0, यदि f ( - x) = - f (x)
कथन - I सत्य है, कथन - II सत्य है, कथन - II, कथन - I का सही स्पष्टीकरण है।
कथन - I सत्य है, कथन - II सत्य है, कथन - II, कथन - I का सही स्पष्टीकरण नहीं है।
कथन - I सत्य है,परन्तु कथन - II असत्य है
कथन - I असत्य है,परन्तु कथन - II सत्य है
The correct Answer is:A
Step by step video, text & image solution for कथन - I : int _(-3) ^(3) (1)/(x) cosec^(98) (x - (1)/( x) ) dx = 0 क्योकि कथन - II : int _( - a) ^(a) f (x) dx = 0, यदि f ( - x) = - f (x) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DEFINITE INTEGRATIONBOOK - ALLEN HINDICHAPTER - DEFINITE INTEGRATIONEXERCISE - Exercise - 03 (गद्यांश आधारित प्रश्न) गद्यांश 1
3 Videos
- DEFINITE INTEGRATIONBOOK - ALLEN HINDICHAPTER - DEFINITE INTEGRATIONEXERCISE - Exercise - 03 (गद्यांश आधारित प्रश्न) गद्यांश 2
3 Videos
- DEFINITE INTEGRATIONBOOK - ALLEN HINDICHAPTER - DEFINITE INTEGRATIONEXERCISE - Exercise - 03 (स्तम्भ सुमेलन)
3 Videos
- CONTINUITY BOOK - ALLEN HINDICHAPTER - CONTINUITY EXERCISE - EXERCISE -05 [B] (JEE-ADVANCED: PREVIOUS YEAR QUESTIONS)
2 Videos
- DETERMINANTBOOK - ALLEN HINDICHAPTER - DETERMINANTEXERCISE - DO YOURSELF -9
1 Video
Similar Questions
माना g(x)=∫x0f(t)dt, जहाँ f एक फलन है जिसका आरेख दर्शाया गया है।
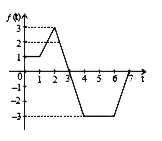
x∈[0,7] में g (x) का अधिकतम मान होगा -
View Solutionफलन f (x) का अन्तराल [ a, b] में, औसत मान संख्या μ=1b−a∫baf(x)dx है |
{1b−a∫ba[f(x)]2dx}1/2 को [a, b] में f का वर्ग माध्य मूल मान कहते है। μ औसत मान प्राप्त करेगा यदि f, [ a, b] का संतत् हो।
अन्तराल [0,π] पर y = sin x की औसत कोटि है -
View Solutionनिम्न प्रश्न में दो स्तम्भ में वत्क्व्य (statements) दिये गए है जिनका सम्मेलन (match) करना है । स्तम्भ - I(Column-I) के वत्क्व्यो को A, B, C तथा D नामित किया गया है जबकि स्तम्भ -II - (Column-II) के वत्क्व्यो को p,q, r तथा s नामित किया ज्ञात है। स्तम्भ- I (Column-I ) में दिए गए कोई एक वत्क्व्य स्तम्भ -II (Column-II ) के एक वत्क्व्य से सही सुमेल करता है।

View Solutionफलन f(x)=⎧⎨⎩e1/x−1e1/x+1जबx≠01जबx=0 का x=0 पर सततता का परीक्षण कीजिए।
View Solutionसमीकरण निकाय λx+y+z=0,−x+λy+z=0,−x−y+λz=0 का एक अशून्य हल होगा यदि λ का वास्तविक मान है
View SolutionIf int f(x)dx=F(x), then int x^(3)f(x^(2))dx is equal to:
View Solutionint(x^(3)+x+1)/(x^(2)-1)dx is equal to f(x) and f(0)=4 then constant term in f(x) is equal to
View SolutionIf f(x)+int{f(x)}^(-2)dx=0 and f(1)=0 then f(x) is
View Solutionint(x^(6)-1)/(x^(4)+x^(3)-x-1)dx=f(x) and backslash f(0)=0
View SolutionIf (X)=f(X),f(0)=1 then ∫dxf(x)+f(−x)=
View SolutionIf f(x)=∫dxx1/3+2 and f(0)=12loge2, then the value of f(−1) is−.
View SolutionLet f(x)=∫1(1+x2)3/2dx and f(0)=0 then f(1)=
View SolutionIf f'(x)=f(x)+∫10f(x) dx and given f(0)=1, then ∫f(x)dx is equal to :
View SolutionLet the function f be defined by
f(x)=|x−1|−12,0≤x≤2,f(x+2)=f(x) for all x∈R.
Evaluate (i)∫1000f(x)dx
(ii) ∫10|f(2x)|dx
View SolutionIf f(x).f(1x)=f(x)+f(1x), and f(3)=82, then ∫f(x)x2+1dx=
View Solution
