Obtain an expression for the electric field intenstiy at a point on the equatorial line of an electric dipole.Solution in Kannada

Video Solution

Text Solution

Verified by Experts

Consider an electric dipole of dipole moment $P = q \times 2 a$ placed in vaccum.
Where q= magnitude of either charge
2a - distance between the charges
Let p be the point on the equatorial line of the dipole at a distance r from the centre of the dipole O (fig )
Electric field at P due to the charge +q is
${\to E}_{1} = (\frac{1}{4 π ε_{0}}) \frac{q}{(r^{2} + a^{2})} ˆ P$
along Ap .... (1)
Electric field at p due to the charge - q is
${\to E}_{2} (\frac{1}{4 π ε_{0}}) \frac{q}{(r^{2} + a^{2})} ˆ P$
along PB .... (2)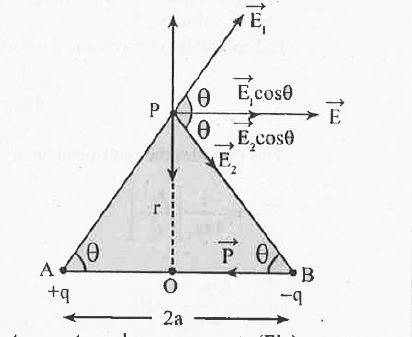
Electric fileds ${\to E}_{1}$ and $V e c E_{2}$ can be resolve into two rectangular components (Fig0 THe components ${\to E}_{1}$ and $sin θ$ and ${\to E}_{2} sin θ$ are equal in magnitude but acting in opposite direction , so they cancel each other . The component ${\to E}_{1} cos θ$ and ${\to E}_{2} cos θ$ are acting parallel to the dipole axis gets at P is
$\to = (E_{1} cos θ + E_{2} cos t^) ˆ p$
$\to E = - 2 E_{1} cos θ ˆ P$
$∴ E_{1} = E_{2}$
From equation (1) we get
$\to E = - 2 [(\frac{1}{4 π ε_{0}}) \frac{q}{(r^{2} + a^{2})}] cos θ ˆ P$
$= - 2 [(\frac{1}{4 π ε_{0}}) \frac{q}{(r^{2} + a^{2})}] \frac{a}{{(r^{2} + a^{2})}^{1 / 2}} ˆ p$
$= - (\frac{1}{4 π ε_{0}}) \frac{2 a q}{{(r^{2} + a^{2})}^{3 / 2}} ˆ p$
$\to E = - (\frac{1}{4 π ε_{0}}) \frac{p}{{(r^{2} + a^{2})}^{3 / 2}}$
If the dipole is short (i.e., a $< <$ r) n $a^{2}$ can be neglected.
$\to E = - (\frac{1}{4 π ε_{0}}) \frac{p}{{(r^{2})}^{3 / 2}})$
$\to E = - (\frac{1}{4 π ε_{0}}) \frac{p}{r^{3}}$

Updated on:21/07/2023

|

Class 12 PHYSICS ANNUAL EXAM QUESTION PAPER MARCH -2015

Topper's Solved these Questions

Similar Questions

SUNSTAR PUBLICATION-ANNUAL EXAM QUESTION PAPER MARCH -2015-PART-A

Home
Ask Doubt
Profile