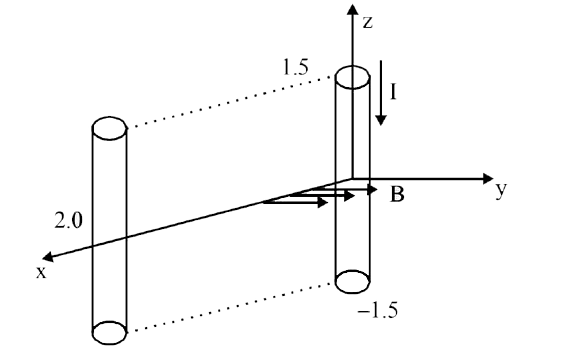
A conductor lies along the z-axis at $- 1.5 \leq z \leq 1.5$ m and carries a fixed current of $1.0 A$ in $- {\hat{a}}_{z}$ direction (see figure). For a field $\vec{B} = 3.0 \times 10^{- 4} e^{- 0.2 x} {\hat{a}}_{y}$ T, find the power required to move the conductor at constant speed to $x = 2.0 m, y = m$ in $5 \times 10^{- 3} s$ . Assume parallel motion along the x-axis.

A

1.57 W

B

2.97 W

C

4.45 W

D

9.87 W

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Force exerted on curretn carrying conductor (F)=BII
Given , $B = 3 \times 10^{4} e^{0.2 x} a y, I = 10.0 A$
$A v e r a \geq p o w e r = \frac{W or k d o \neq}{T i m e t a k e n}$
$P = \frac{1}{5 \times 10^{- 3} \int \overset{2}{\int_{0}} 3 \times 10^{- 4} e^{- 0.2 x} \times 10 \times 3 d x}$
$= 9 [1 - e^{- 0.4}] = 9 [1 - \frac{1}{e^{0.4}}] = 2.967 W = 2.97 W$

Updated on:21/07/2023

|

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

DC PANDEY ENGLISH-SOLVED PAPER 2017-Solved papers 2017(AIIMS)

Home
Ask Doubt
Profile