A long solenoid of diameter 0.1 m has 2×104 turns per meter. At centre of the solenoid is 100 turns coil of radius 0.01 m placed with its axis coinciding with solenoid axis. The current in the solenoid reduce at a constant rate to 0A from 4 a in 0.05 s . If the resistance of the coil is 10π2Ω, the total charge flowing through the coil during this time is
32πμC
16μC
32μC
16πμc
The correct Answer is:C
To solve the problem step by step, we will calculate the total charge flowing through the coil during the time the current in the solenoid decreases.
Step 1: Identify the parameters
- Diameter of the solenoid = 0.1 m, hence radius rs=0.05 m.
- Turns per meter of the solenoid ns=2×104 turns/m.
- Number of turns in the coil N=100.
- Radius of the coil rc=0.01 m.
- Initial current Ii=4 A, final current If=0 A.
- Time duration t=0.05 s.
- Resistance of the coil R=10π2Ω.
Step 2: Calculate the change in current
The change in current ΔI is given by:
ΔI=If−Ii=0−4=−4A
Step 3: Calculate the magnetic field inside the solenoid
The magnetic field B inside a solenoid is given by:
B=μ0nsI
Where μ0=4π×10−7T m/A.
Step 4: Calculate the initial magnetic field
Using the initial current Ii=4 A:
Bi=μ0nsIi=(4π×10−7)×(2×104)×4
Calculating this gives:
Bi=32π×10−3T
Step 5: Calculate the final magnetic field
Using the final current If=0:
Bf=μ0nsIf=(4π×10−7)×(2×104)×0=0T
Step 6: Calculate the change in magnetic field
ΔB=Bf−Bi=0−32π×10−3=−32π×10−3T
Step 7: Calculate the area of the coil
The area A of the coil is given by:
A=πr2c=π(0.01)2=π×10−4m2
Step 8: Calculate the change in magnetic flux
The change in magnetic flux ΔΦ through the coil is given by:
ΔΦ=N⋅A⋅ΔB
Substituting the values:
ΔΦ=100⋅π×10−4⋅(−32π×10−3)
ΔΦ=−3200π2×10−7Wb
Step 9: Calculate the induced EMF
Using Faraday's law, the induced EMF E is:
E=−ΔΦΔt=3200π2×10−70.05
Calculating this gives:
E=64000π2×10−7V
Step 10: Calculate the total charge Q
Using Ohm's law, the total charge Q is given by:
Q=E⋅ΔtR
Substituting the values:
Q=64000π2×10−7⋅0.0510π2
Q=3200π2×10−710π2=320×10−6C=32μC
Final Answer
The total charge flowing through the coil during this time is 32μC.
- Diameter of the solenoid = 0.1 m, hence radius rs=0.05 m.
- Turns per meter of the solenoid ns=2×104 turns/m.
- Number of turns in the coil N=100.
- Radius of the coil rc=0.01 m.
- Initial current Ii=4 A, final current If=0 A.
- Time duration t=0.05 s.
- Resistance of the coil R=10π2Ω.
The change in current ΔI is given by:
ΔI=If−Ii=0−4=−4A
The magnetic field B inside a solenoid is given by:
B=μ0nsI
Where μ0=4π×10−7T m/A.
Using the initial current Ii=4 A:
Bi=μ0nsIi=(4π×10−7)×(2×104)×4
Calculating this gives:
Bi=32π×10−3T
Using the final current If=0:
Bf=μ0nsIf=(4π×10−7)×(2×104)×0=0T
ΔB=Bf−Bi=0−32π×10−3=−32π×10−3T
The area A of the coil is given by:
A=πr2c=π(0.01)2=π×10−4m2
The change in magnetic flux ΔΦ through the coil is given by:
ΔΦ=N⋅A⋅ΔB
Substituting the values:
ΔΦ=100⋅π×10−4⋅(−32π×10−3)
ΔΦ=−3200π2×10−7Wb
Using Faraday's law, the induced EMF E is:
E=−ΔΦΔt=3200π2×10−70.05
Calculating this gives:
E=64000π2×10−7V
Using Ohm's law, the total charge Q is given by:
Q=E⋅ΔtR
Substituting the values:
Q=64000π2×10−7⋅0.0510π2
Q=3200π2×10−710π2=320×10−6C=32μC
The total charge flowing through the coil during this time is 32μC.
Topper's Solved these Questions
- SOLVED PAPER 2017BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(AIIMS)
34 Videos
- SOLVED PAPER 2017BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(JIPMER)
32 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
A long solenoid of diameter 0.1m has 2×104 turns per metre. At the centre of the solenoid, a 100−turns coil of radius 0.01m is placed with its axis coinciding with the constant rate from +2A to 2A in 0.05s. Find the total charge (in μC) flowing through the coil during this time when the resistance of the coil is 40≠2Ω.
View SolutionA current l = 1.5 A is flowing through a long solenoid of diameter 3.2 cm, having 220 turns per cm. At its centre, a 130 turn closely packed coil of diameter 2.1 cm is placed such that the coil is coaxial with the long solenoid. The current in the solenoid is reduced to zero at a steady rate in 25 ms. What is the magnitude of emf induced in the coil while the current in the solenoid is changing?
View Solution
Knowledge Check
A long solenoid with 10 turns per cm has a small loop of area 3cm2 placed inside, normal to the axis of the solenoid. If the currnet carried by the solenoid changes steadily from 2 A to 4 A in 0.2 s, what is the induced voltage in the loop, while the current is changing?
A4.2×10−8VB2.8×10−8VC7.3×10−6VD3.8×10−6V
Through a long solenoid of diameter 4.1 cm, having 100 turns per cm, a current I = 1A is flowing. At its centre, a 60 turns closely packed coil of diameter 3.1 cm si placed such that the coil is coaxial with the long solenoid. The current in the solenoid is reduced to zero at a steady rate in 10 ms. What is the magnitude of emf induced in the coil while the current in the solenoid is changing?
View SolutionAn ideal solenoid of cross-sectional area 10−4m2 has 500 turns per metre. At the centre of this solenoid, another coil of 100 turns is wrapped closely around it. If the current in the coil changes from 0 to 2 A in 3.14 ms, the emf developed in the second coil is
View SolutionA long solenoid with 15 turns per cm has small loop of area 2.0cm2 placed inside, normal to the axis of the soleniod. If current carried by the solenoid changes steadily from 2 A to 4 A in 0.1 s, what is the induced voltage in the loop, while the current is changing ?
View SolutionA solenoid of 2.5 m length and 2.0 cm diameter possesses 10 turns per cm. A current of 0.5 A is flowing through it . The magnetic induction at axis inside the solenoid is
View SolutionA solenoid has 2000 turns would over a length of 0.30 m. The area of its cross-section is 1.2×10−3m2. If an initial current of 2 A in the solenoid is reversed in 0.25 s, then the emf induced in the coil is
View SolutionA long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
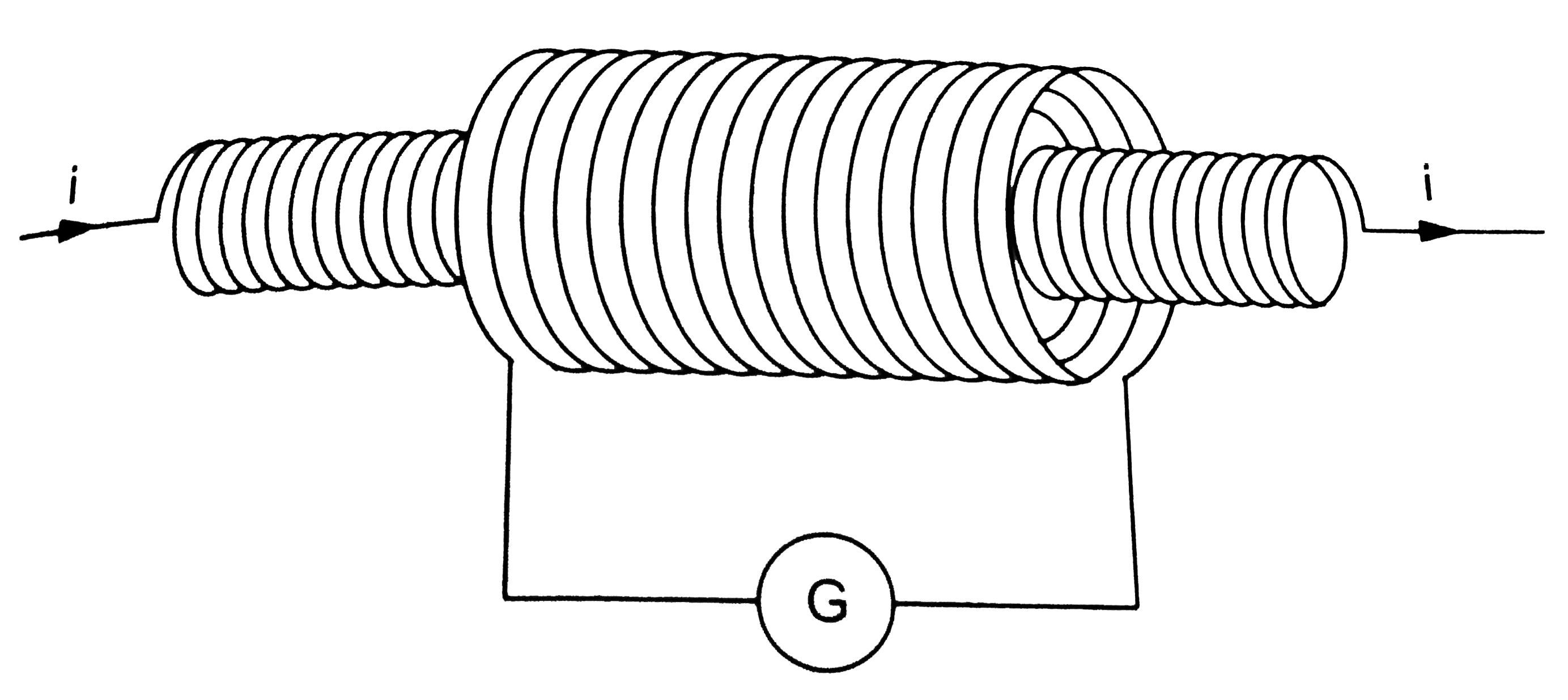
View SolutionA long solenoid of radius 2 cm has 100 turns/cm and is surrounded by a 100- turn coil of radius 4cm having a total resistance of 20Ω. The coil is connected to a galvanometer as shown in fig. If the current in the solenoid is changed form 5 A in one direction to 5 A in the opposite direction, find the charge which flows through the galvanometer.
View Solution
