A plane polarized light is incident normally on a tourmaline plate. Its E vector make an angle of 60∘ with the optic axis of the plate. Find the percentage difference between initial and final intensities
50%
25%
75%
90%
The correct Answer is:C
To solve the problem of finding the percentage difference between the initial and final intensities of plane polarized light incident on a tourmaline plate, we can follow these steps:
1. Understanding the Problem:
We have plane polarized light incident normally on a tourmaline plate. The electric field vector (E vector) makes an angle of 60∘ with the optic axis of the plate. We need to find the percentage difference between the initial intensity I and the final intensity I′ after passing through the plate.
2. Applying Malus's Law:
According to Malus's Law, the intensity of polarized light after passing through a polarizer (in this case, the tourmaline plate) is given by:
I′=Icos2θ
where I is the initial intensity, I′ is the final intensity, and θ is the angle between the light's electric field vector and the optic axis of the polarizer.
3. Substituting the Given Values:
Here, θ=60∘. Therefore, we can calculate I′:
I′=Icos2(60∘)
We know that cos(60∘)=12, so:
I′=I(12)2=I⋅14
4. Calculating the Final Intensity:
Thus, the final intensity I′ is:
I′=I4
5. Finding the Percentage Difference:
The percentage difference between the initial and final intensities can be calculated using the formula:
Percentage Difference=I−I′I×100
Substituting I′ into the equation:
Percentage Difference=I−I4I×100
6. Simplifying the Expression:
This simplifies to:
Percentage Difference=I−I4I×100=4I4−I4I×100=3I4I×100
The I cancels out:
Percentage Difference=34×100=75%
Final Answer:
The percentage difference between the initial and final intensities is 75%.
We have plane polarized light incident normally on a tourmaline plate. The electric field vector (E vector) makes an angle of 60∘ with the optic axis of the plate. We need to find the percentage difference between the initial intensity I and the final intensity I′ after passing through the plate.
According to Malus's Law, the intensity of polarized light after passing through a polarizer (in this case, the tourmaline plate) is given by:
I′=Icos2θ
where I is the initial intensity, I′ is the final intensity, and θ is the angle between the light's electric field vector and the optic axis of the polarizer.
Here, θ=60∘. Therefore, we can calculate I′:
I′=Icos2(60∘)
We know that cos(60∘)=12, so:
I′=I(12)2=I⋅14
Thus, the final intensity I′ is:
I′=I4
The percentage difference between the initial and final intensities can be calculated using the formula:
Percentage Difference=I−I′I×100
Substituting I′ into the equation:
Percentage Difference=I−I4I×100
This simplifies to:
Percentage Difference=I−I4I×100=4I4−I4I×100=3I4I×100
The I cancels out:
Percentage Difference=34×100=75%
The percentage difference between the initial and final intensities is 75%.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE main (Only one option is Correct )22videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced Only one option is correct22videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - match column4videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER22videos
SOLVED PAPERS 2018
Similar Questions
Plane polarised light is incident on an analyser . The intensity then becomes three fourth . The angle of the axis of the analyser with the beam is
View SolutionA plane polarized light with intensity I0 is incident on a polaroid with Electric Field vector making an angle of 60∘ with transmission axis of polaroid. The intensity of the resulting light will be
View Solution
Knowledge Check
- Question 1 - Select One
A ray of light in incident on a glass plate at an angle of 60∘. What is the refractive index of glass if the reflected and refracted rays are perpendicular to each other?
A12B√32C32D√3
A light ray is incident normally on a plane mirror.
What is its angle of incidence?View SolutionA polarized light of intensity I0 is passed through another polarizer whose pass axis makes an angle of 60∘ with the pass axis of the former, What is the intensity of emergent polarized light from second polarizer?
View SolutionA polarised light intensity I0 is passed through another polariser whose pass axis makes an angle of 60∘ with the pass axis of the former, What is the intensity of emergent polarised light from second polarised?
View SolutionAn unpolarised beam of intensity I0 is incident on a pair of nicols making an angle of 60∘ with each other. The intensity of light emerging from the pair is
View SolutionA plane polarized light is incident on a polariser with its pass axis making anlge θ with x axis as shown in the figure At four different values of θ,θ=8∘,38∘,188∘and218∘ the observed intensities are same what is the angle between the direction of polariztion and x axis ?
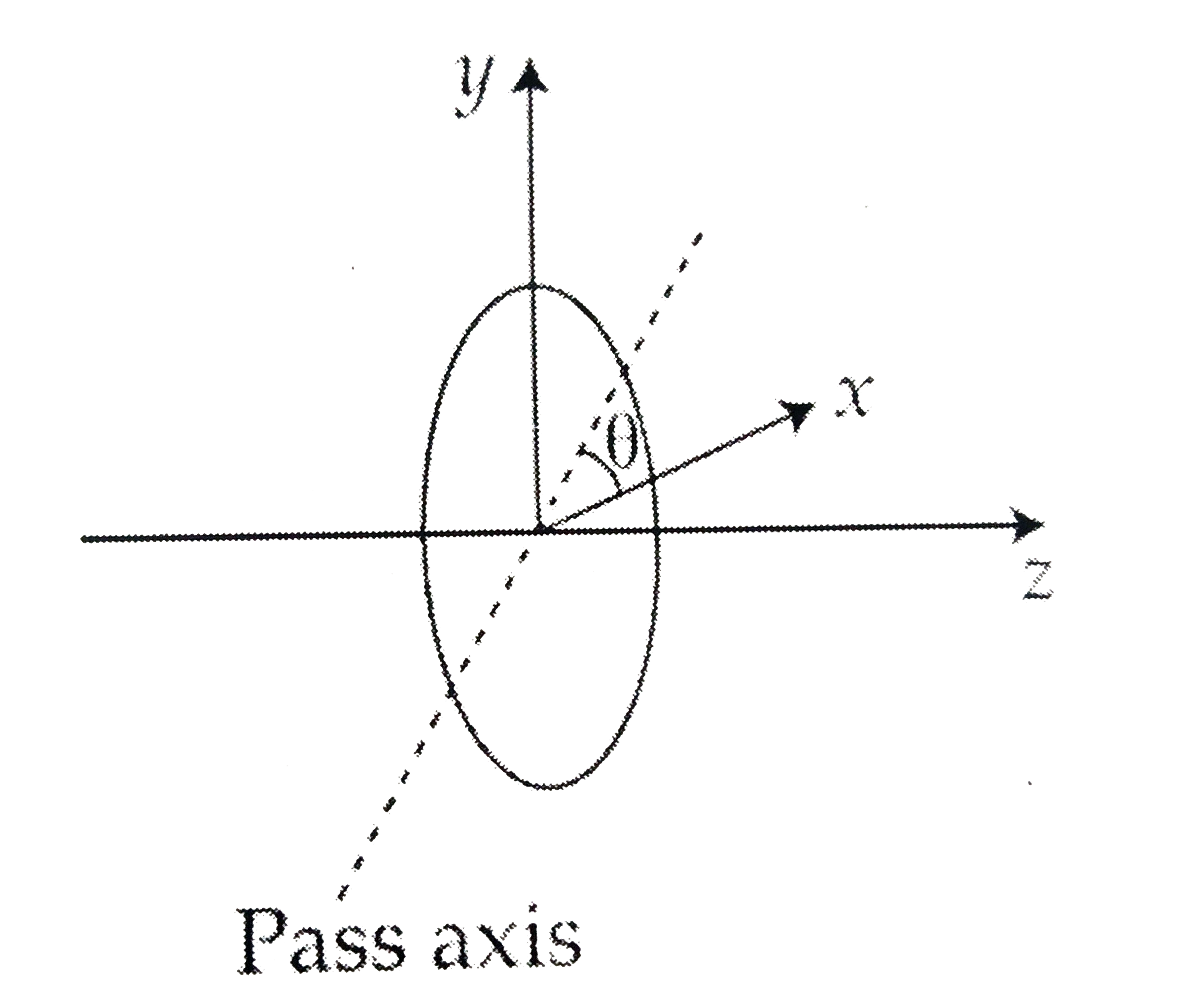 View Solution
View SolutionA unpolarised light is passed through 3 polarisers. If the second polariser is at an angle 30∘ with the first and the third polariser is at an angle 60∘ with the second. Find the final intensity of the light passed through this combination in initial intensity was I.
View SolutionUnpolarized light is incident on a plane surface of glass of refractive index μ at angle i. If the reflected light gets totally polarized, write the relation between the angle i and refractive index μ.
View Solution
