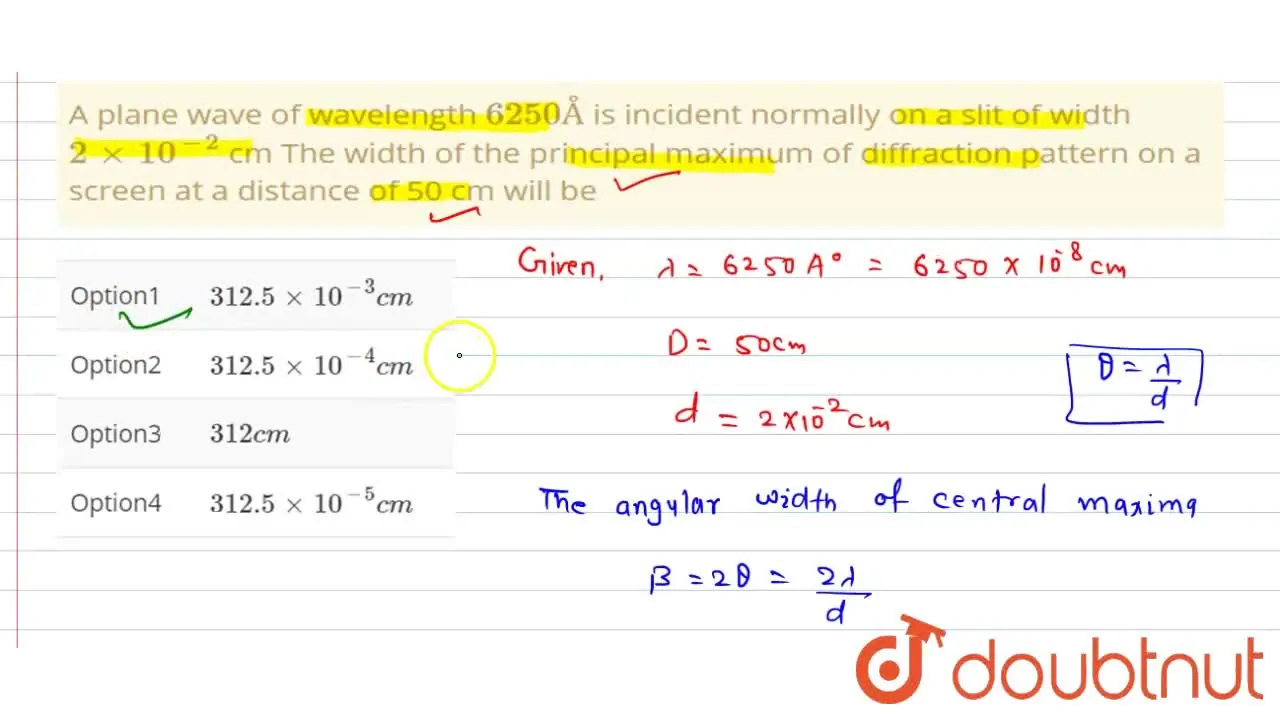
A plane wave of wavelength 6250Å is incident normally on a slit of width 2×10−2 cm The width of the principal maximum of diffraction pattern on a screen at a distance of 50 cm will be
312.5×10−3cm
312.5×10−4cm
312cm
312.5×10−5cm
The correct Answer is:A
According to the question
λ=6250Å=6250×10−10×102cm=6250×10−8cm
Width of the slit a=2×10−2cm
The angular width of central maxima β=2θ=2λa
Thus, linear width is
L=β×D=2λa×D=2×6250×10−82×10−2×50
=6250×10−6×50=3125×10−4
=312.5×10−3cm
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE main (Only one option is Correct )22videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced Only one option is correct22videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - match column4videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER22videos
SOLVED PAPERS 2018
Similar Questions
Light of wavelength 600 nm is incident normally on a slit of width 0.2 mm. The angular width of central maxima in the diffraction pattern is
View SolutionLight of wavelength 6328Å is incident normally on a slit of width 0.2 mm. Angular width of the central maximum on the screen will be :
View SolutionLight of wavelength 5000Å is incident over a slit of width 1μm. The angular width of central maxima will be
View SolutionLight wavelength 6000 Å is incident normally on a slit of width 24×10−5 cm. find out the angular position of seconds minimum from central maximum?
View SolutionHow does the angular width of principal maximum in the diffraction pattern vary with the width of slit ?
View SolutionLight of wavelength 589.3nm is incident normally on the slit of width 0.1mm. What will be the angular width of the central diffraction maximum at a distance of 1m from the slit?
View SolutionLights of wavelength 550 nm falls normally on a slit of width k22.0×10−5cm. The angular position of the central maximum will be (in radian) :
View SolutionA parallel beam of light of wavelength 4000Å passes through a slit of width 5×10−3m. The angular spread of the central maxima in the diffraction pattern is
View SolutionMonochromatic light of a wavelength 650 nm falls normally on a slit of width 13×10−4 cm and the resulting Fraunhofer diffraction is obtained on a screen. Find the angular width of the central maxima.
View SolutionMonochromatic light of a wavelength 650 nm falls normally on a slit of width 1:3×10−4 cm and the resulting Fraunhofer diffraction is obtained on a screen. Find the angular width of the central maxima.
View Solution