Consider a ray of light incident from air onto a slab of glass (refractive index n) of width d, at angle $θ$ . The phase difference between the ray reflected by the top surface of the glass and the bottom surface is

A

$\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{- 1 / 2} + π$

B

$\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2}$

C

$\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2} + \frac{π}{2}$

D

$\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2} + 2 π$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Consider the diagram the ray (P) is incident at an angle $θ$ and gets reflected in the direction P and refracted in the direction P Due to reflection from the glass medium there is a phase change of $π$
Time taken to travel along OP
$Δ t = \frac{O P}{v} = \frac{d / cos r}{c / n} = \frac{n d}{c cos r}$
From Snell's law $n = \frac{sin θ}{sin r} \Rightarrow sin r = \frac{sin θ}{n}$
$cos r = \sqrt{1 - {sin}^{2} r} = \sqrt{1 - \frac{{sin}^{2} θ}{n^{2}}}$
$∴ Δ t = \frac{n d}{c {(1 - \frac{{sin}^{2} θ}{n^{2}})}^{1 / 2}} = \frac{n^{2} d}{c} {(1 - \frac{{sin}^{2} θ}{n^{2}})}^{- 1 / 2}$

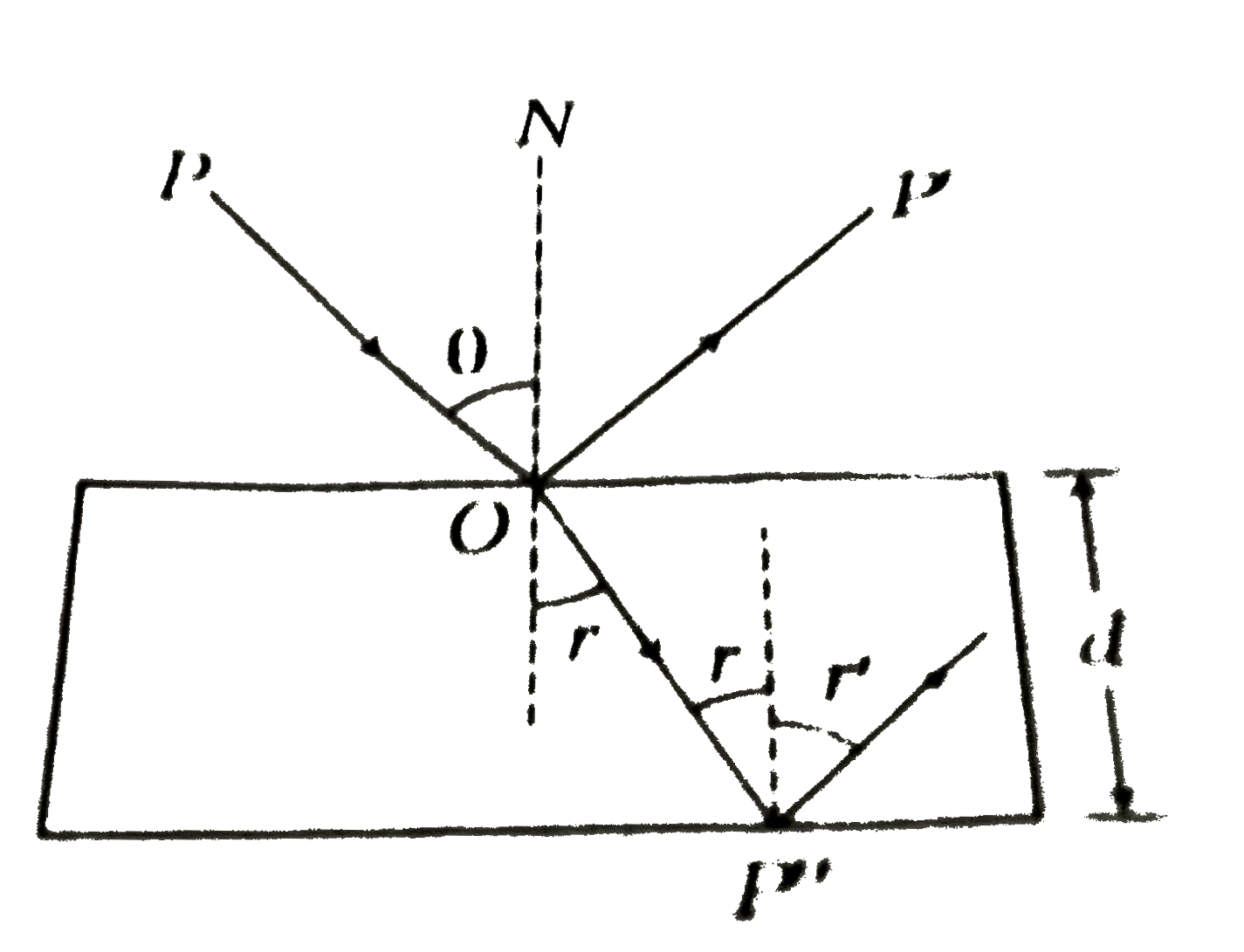
Phase difference $= Δ ϕ = \frac{2 π}{T} \times Δ t = \frac{2 π n d}{λ} {(1 - \frac{{sin}^{2} θ}{n^{2}})}^{- 1 / 2}$
So, net phase difference $= Δ ϕ + π = \frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{- 1 / 2} + π$

|

Updated on:21/07/2023

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Consider a ray of light incident from air onto a slab of glass (refractive index n) of width d, at an angle $θ$ . The phase difference between the ray reflected by the top surface of the glass and the bottom surface is
A $\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2} + π$
B $\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2}$
C $\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2} + \frac{π}{2}$
D $\frac{4 π d}{λ} {(1 - \frac{1}{n^{2}} {sin}^{2} θ)}^{1 / 2} + 2 π$
Question 2 - Select One
A ray of light strikes a transparent rectangular slab of refractive index $\sqrt{2}$ at an angle of incidence of $45^{\circ}$ . The angle betweent the reflected and refracted rays is
A $75^{\circ}$
B $90^{\circ}$
C $105^{\circ}$
D $120^{\circ}$

DC PANDEY ENGLISH-WAVE OPTICS-taking it together

Home
Ask Doubt
Profile