Two parallel rails with negligible resistance are 10.0cm apart. The are connected by a 5.0Ω resistor. The circuit also contains two metal rods having resistances of 10.0Ω and 15.0Ω along the rails. The rods are pulled away from the resistor at constant speeds 4.00ms and 2.00m/s respectively. A uniform magnetic field of magnitude 0.01T is applied perpendicular to the, plane of the rails. Determine the current in the 5.0Ω resistor.
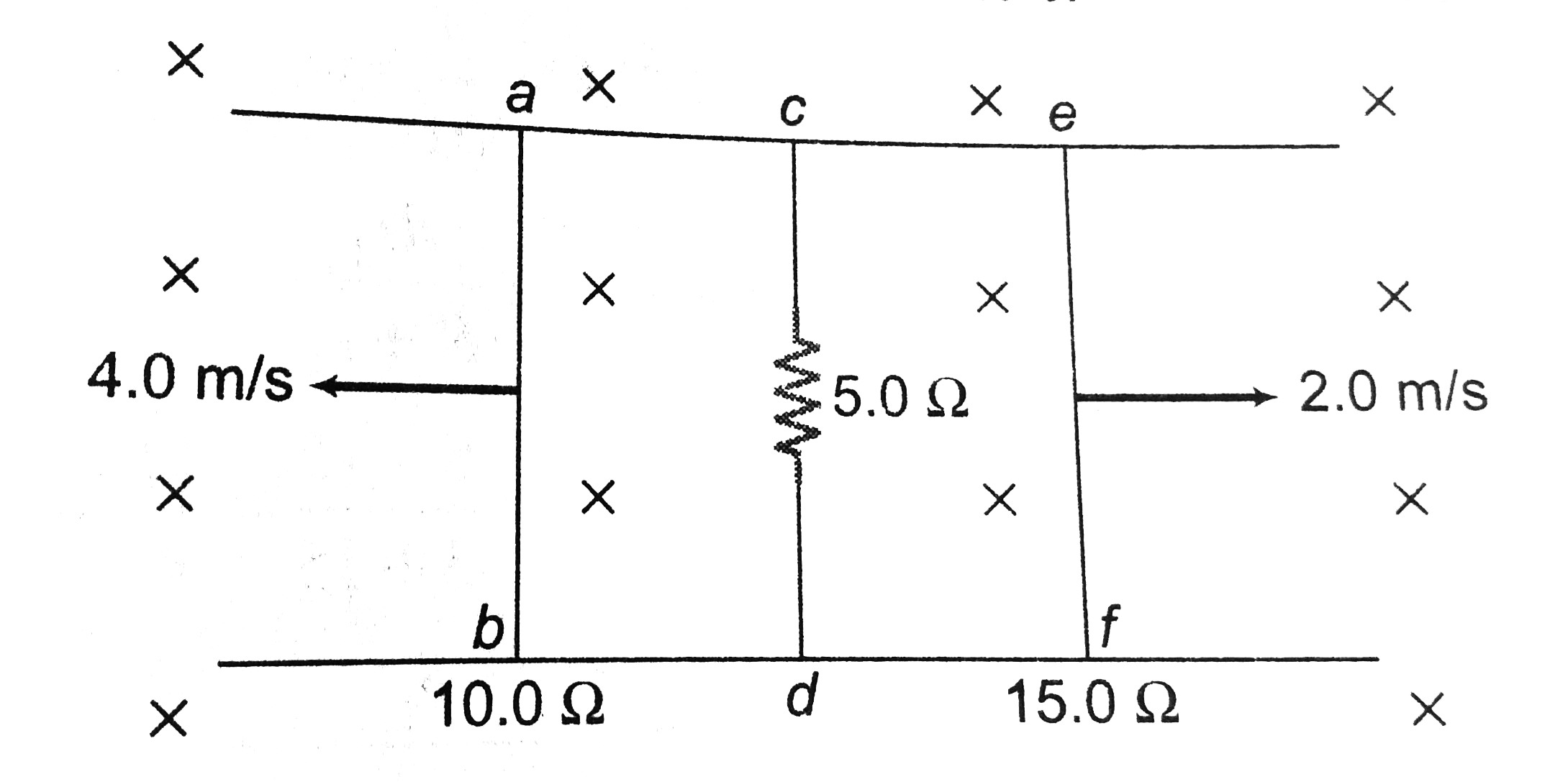
Here, two conductors are moving in uniform magnetic field. So we will use the motional approach. The rod ab will act as a source of emf,
e1=Bvl=(0.01)(4)(0.1)=4×10−3V
and internal resistance, r1=10Ω ltBrgt Similarly, rod ef will also act as a source of emf,
e2=(0.01)(2)(0.1)=2×10−3V
and internal resistance, r2=15Ω
From right hand rule we can see that
Vb>VaandVe>Vf
Now, either by applying Kirchhoff's laws or applying principle of superposition (discussed in the chapter of current electricity) we can find current through 5Ω resistor. We will here use the superposition principle. You solve it by using Kirchhoff's laws.
Now, in the figures, R=5Ω,r1=10Ω,r2=15Ω,
e1=4×10−3Vande2=2×10−3V.

(b) Net resistance of the circuit
=r2+Rr1R+r1=15+10×510+5=553Ω
∴Current, i=e2Net resistance
=2×10−355/3=655×10−3A
∴ Current through R,
i1=(r1R+r1)i=(1010+5)(655×10−3)A
=455×10−3A=455mA
(c) Net resistance of circuit r1+Rr2R+r2=10+5×155+15=554Ω
∴Current,i'=e1Net resistance=4×10−355/4=1635×10−3A
∴ Current through R, i1=(r2R+r2)i'
=(1515+5)(1655)×10−3A=1255mA
From superposition principle net current through 5Ω resistor is
i1'−i1=855mA from d to c
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together
119 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 Subjective
20 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
Two resistors having resistance 4 Ω and 6 Ω are connected in parallel. Find their equivalent resistance.
View SolutionA vertical ring of radius r and resistance on R falls vertically. It is in contact with two vertical rails which are joined at the top. The rails are without friction and resistance. There is a horizontal uniform, magnetic field of magnitude B perpendicular to the plane of the ring and the rails. When the speed of the ring is v , the current in the section PQ is
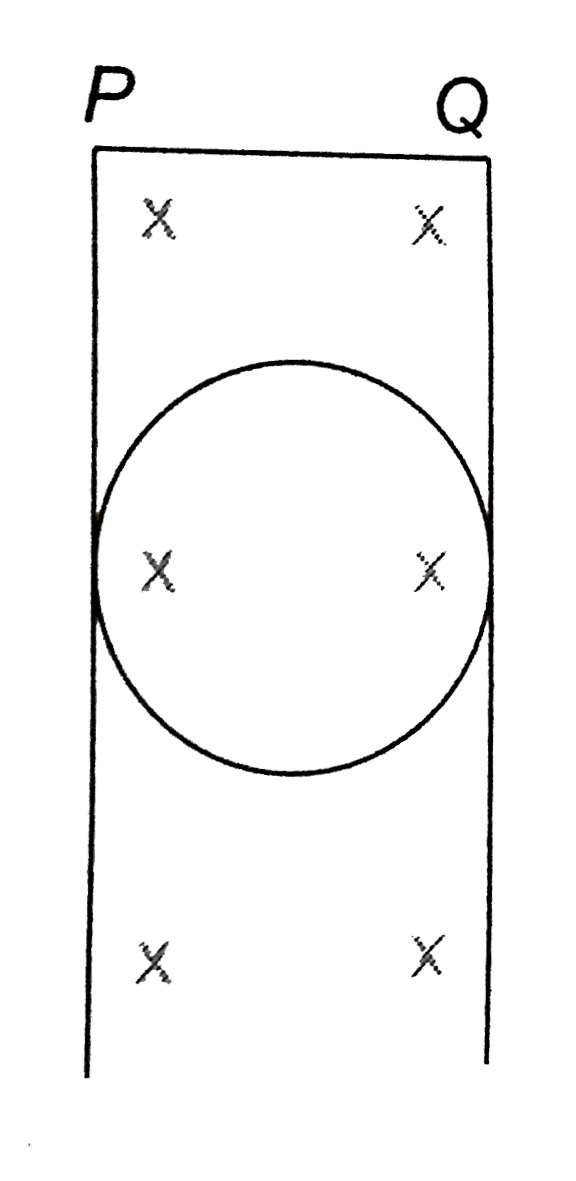
View SolutionA vertical ring of radius r and resistance on R falls vertically. It is in contact with two vertical rails which are joined at the top. The rails are without friction and resistance. There is a horizontal uniform, magnetic field of magnitude B perpendicular to the plane of the ring and the rails. When the speed of the ring is v , the current in the section PQ is

View SolutionThese resistors of 10Ω,15Ωand5Ω are connected in parallel. Find their equivalent resistance.
View SolutionA rod of mass 0.720 kg and radius 6 cm rests on two parallel rails that are d=12cm apart and length of rails is L=49cm. The rod carried a current of I=48A Fig. and rolls along the rails without slipping. A uniform magnetic field of magnetude 0.240 T is directed perpendicular to the rod and the rails. If it starts from rest, what is the speed of the rod as it leaves the rails?

View SolutionA copper rod of mass m slides under gravity on two smooth parallel rails l distance apart set at an angle θ to the horizontal. At the bottom, the rails are joined by a resistance R.
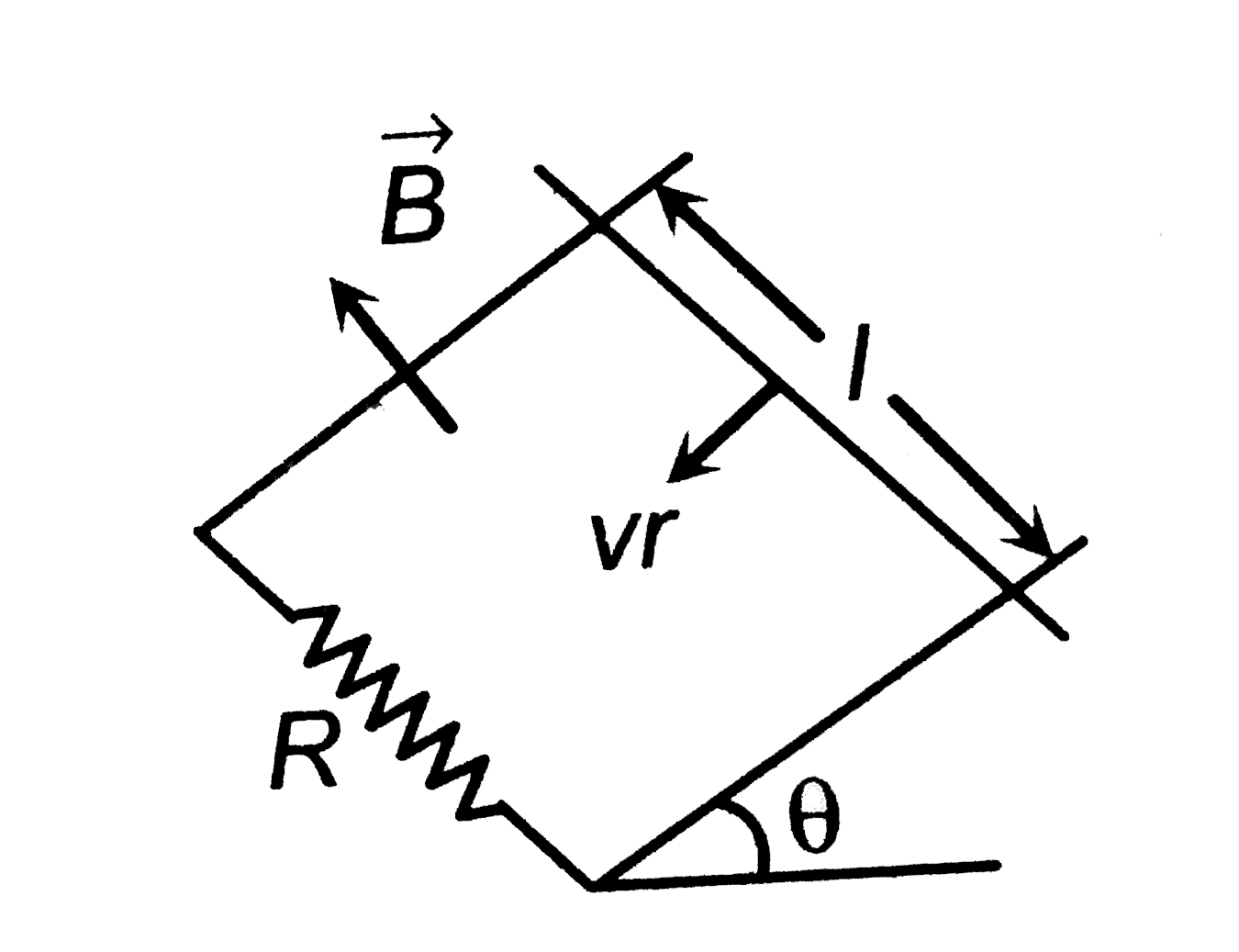
There is a uniform magnetic field perpendicular to the plane of the rails. the terminal valocity of the rod is
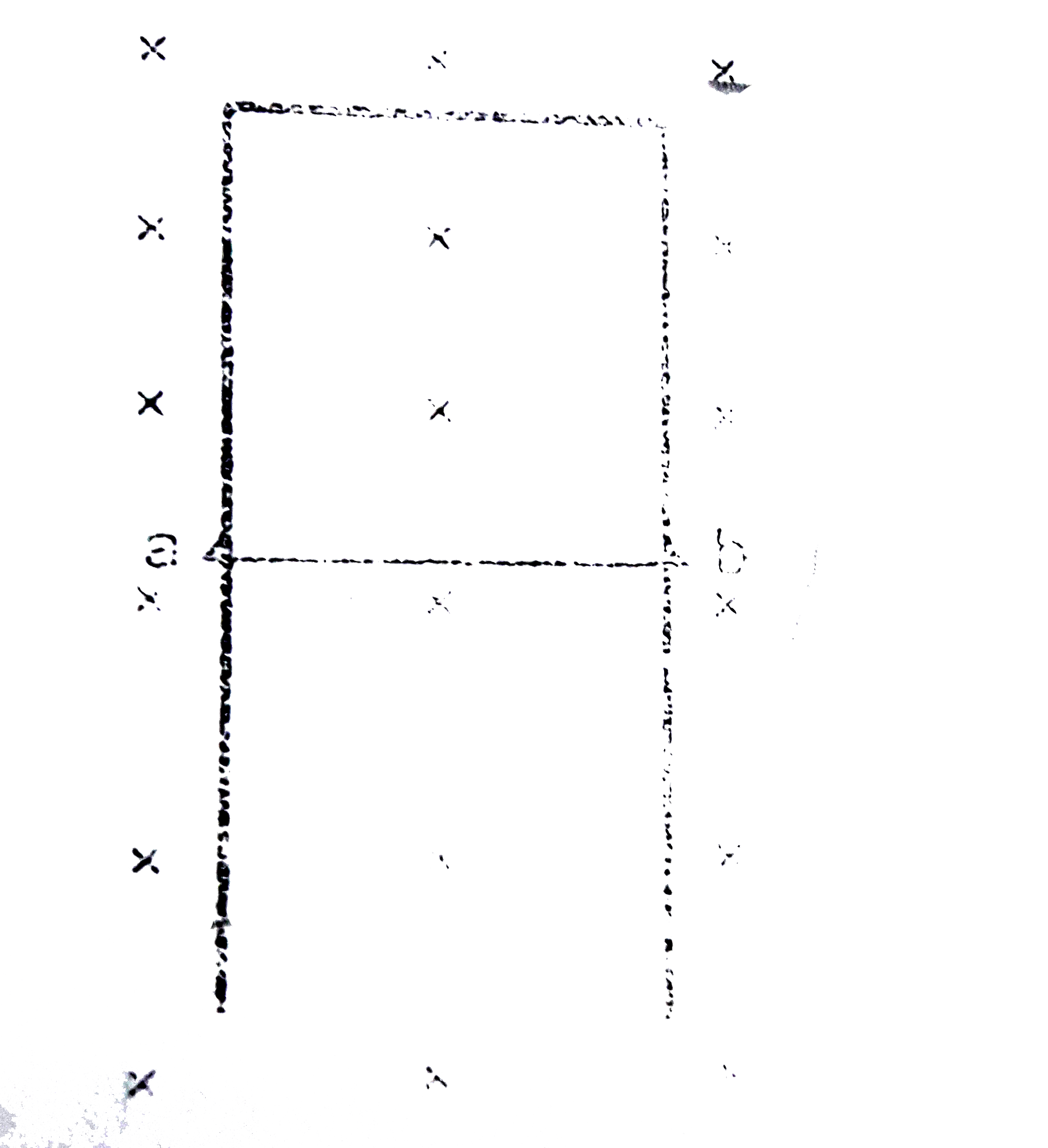
View SolutionA copper wire ab of length l, resistance r and mass m starts sliding at t=0 down a smooth, vertical, thick pair of connected condcuting rails as shown in figure.A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails which options are correct.
View SolutionA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.
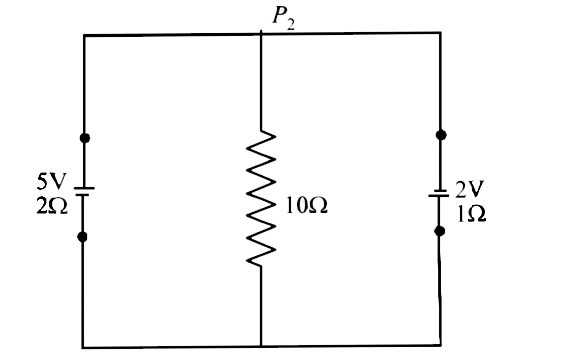
The current in the 10Ω resistor is
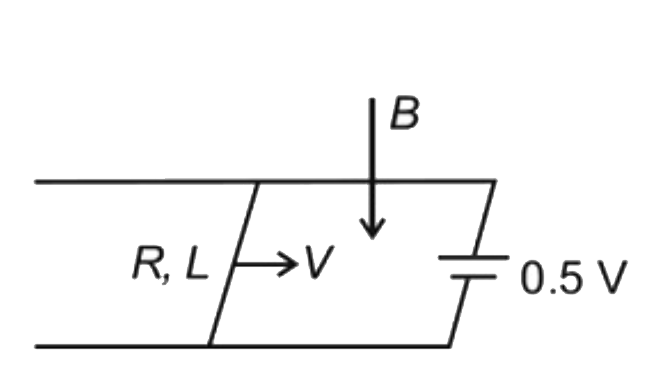
View SolutionThere is a pair of fixed, parallel rails of negligible resistance in a horizontal plane, at a distance of L = 10 cm. The rails are connected, at one of their ends to an ideal battery of EMF 0.5 V, as shown in the figure. The system is placed in a vertically downward and perpendicular magnetic field of magnetic induction B = 1T. A metal rod of resistance R=10Ω is placed perpendicularly on the rails. The rod placed perpendicularly on the rails. The rod and rails are frictionless. The magnitude of force, which is to be exerted on the rod in order to move it with a constant speed of order to move it with a constant speed of 1ms−1 in the direction shown, is
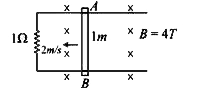
View SolutionTwo fixed long parallel horizontal rails, a distance 1 m apart are joined at one end by a resistance 1Ω. A rod AB of negligible resistance slides towards the resistance. A magnetic field of magnitude 4T perpendicular to the plane of rails exists. Find the current flowing (in Ampere) in the resistance, at an instant when the velocity of the rod is 2 m/s.
View Solution
