Two masses 8 kg 4 kg are suspended together by a massless spring of spring constant 1000Nm−1 . When the masses are in equilibrium 8 kg is removed without disturbing the system . The amplitude of oscillation is
0.5 m
0.08 m
0.4 m
0.04 m
The correct Answer is:B
To solve the problem step by step, we will analyze the situation where two masses (8 kg and 4 kg) are suspended from a spring, and then we will determine the amplitude of oscillation after removing the 8 kg mass.
Step 1: Calculate the total weight of the system before removing the 8 kg mass.
The total weight (W) of the two masses when both are suspended is given by:
W=(m1+m2)⋅g
where m1=8kg, m2=4kg, and g=10m/s2 (approximating gravitational acceleration).
W=(8+4)⋅10=12⋅10=120N
Step 2: Determine the extension of the spring when both masses are attached.
Using Hooke's Law, the extension x1 of the spring can be calculated using:
F=k⋅x
where F is the total weight and k is the spring constant.
Given k=1000N/m:
120=1000⋅x1
x1=1201000=0.12m
Step 3: Calculate the weight of the remaining mass after removing the 8 kg mass.
After removing the 8 kg mass, only the 4 kg mass remains. The weight of the remaining mass W′ is:
W′=m2⋅g=4⋅10=40N
Step 4: Determine the new extension of the spring with only the 4 kg mass.
Using Hooke's Law again for the remaining mass:
40=1000⋅x2
x2=401000=0.04m
Step 5: Calculate the amplitude of oscillation.
The amplitude of oscillation A is the difference in extension of the spring before and after removing the 8 kg mass:
A=x1−x2=0.12−0.04=0.08m
Final Answer:
The amplitude of oscillation is 0.08m.
---
The total weight (W) of the two masses when both are suspended is given by:
W=(m1+m2)⋅g
where m1=8kg, m2=4kg, and g=10m/s2 (approximating gravitational acceleration).
Using Hooke's Law, the extension x1 of the spring can be calculated using:
F=k⋅x
where F is the total weight and k is the spring constant.
120=1000⋅x1
x1=1201000=0.12m
After removing the 8 kg mass, only the 4 kg mass remains. The weight of the remaining mass W′ is:
W′=m2⋅g=4⋅10=40N
Using Hooke's Law again for the remaining mass:
40=1000⋅x2
x2=401000=0.04m
The amplitude of oscillation A is the difference in extension of the spring before and after removing the 8 kg mass:
A=x1−x2=0.12−0.04=0.08m
The amplitude of oscillation is 0.08m.
Similar Questions
Two masses m1=1kgandm2=0.5kg are suspended together by a massless spring of spring constant 12.5Nm−1. When masses are in equilibrium m1 is removed without disturbing the system. New amplitude of oscillation will be
View SolutionTwo masses m 1 and m 2 are suspended together by a massless spring of constant K . When the masses are in equilibrium, m 1 is removed without disturbing the system. The amplitude of oscillations is

View Solution
Knowledge Check
Two masses m1andm2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. Then the angular frequency of oscillation of m2 is
A√(k/m1)B√(k/m2)C√[k(m1+m2)]D√[k/(m1m2)]
Two masses M and m are suspended together by massless spring of force constant -k. When the masses are in equilibrium, M is removed without disturbing the system. The amplitude of oscillations.
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant K. When the masses are in equilibrium, m1 is removed without disturbing the system. Then the angular frequency of oscillation of m2 is -
View SolutionTwo masses m1andm2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. Then the angular frequency of oscillation of m2 is
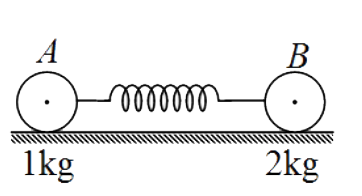
View SolutionTwo uniform discs A and B of equal radii but having different masses 1 kg and 2 kg respectively, are connected (at centres) by a massless spring of spring constant k=1000Nm−1 and then placed on a sufficiently rough horizontal surface. If initially, the system is released from rest with the spring being compressed by 10 cm, then the speed of A when the spring comes back to its natural length, is
View SolutionA mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10cm, then the period of oscillation is

View SolutionTwo blocks of masses 6 kg and 3 kg are attached to the two ends of a massless spring of spring constant 2π2N/m. If spring is compressed and released on a smooth horizontal surface then find the time period (in seconds) of each block.
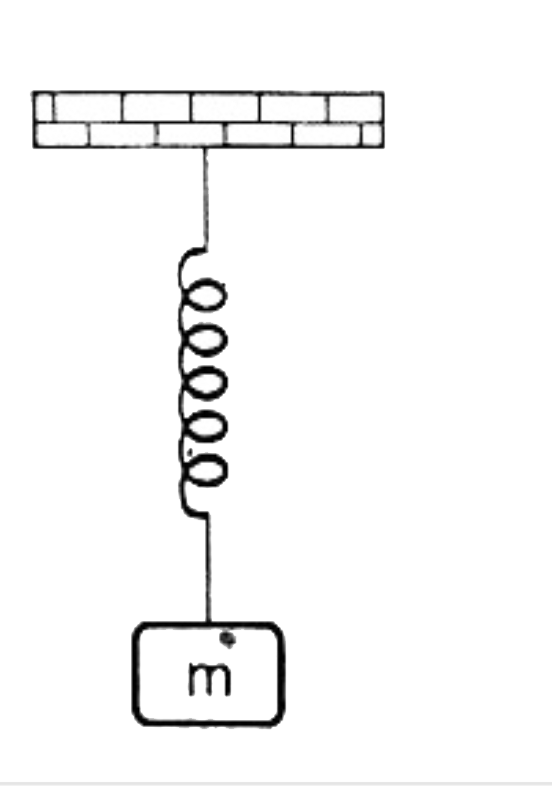
View SolutionA point mass m = 20 kg, is suspended by a massless spring of constant 2000 N/M. The point mass is released when elongation in the spring is 15 cm. The equation of displacement of particle as a function of time is ( Take g=10m/s2 )
View Solution