Question

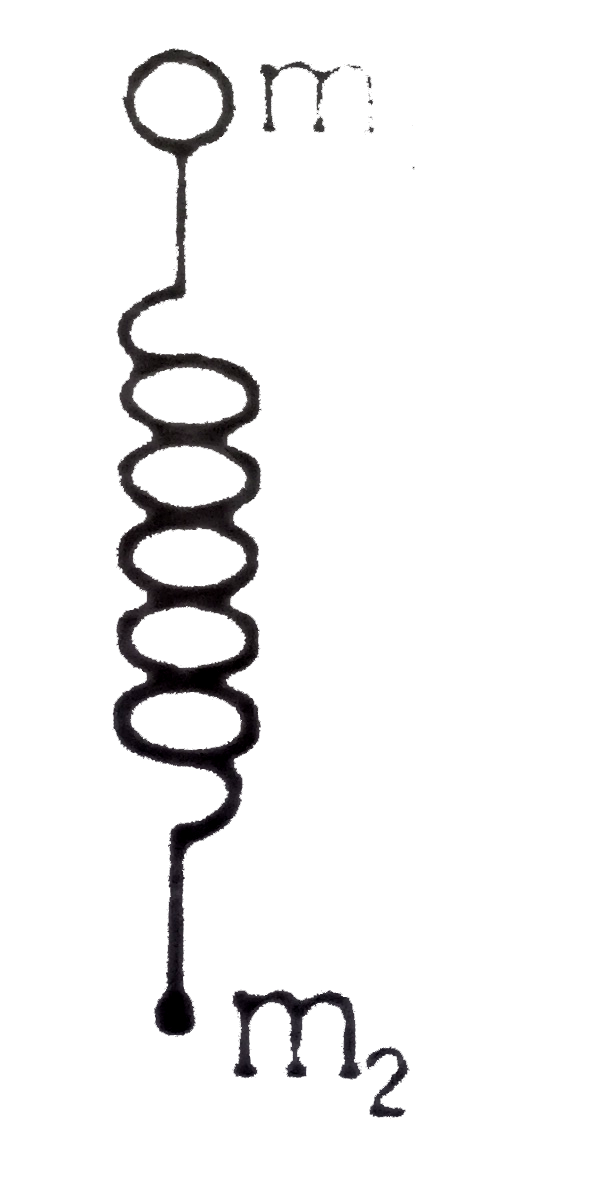
Two masses $m_1\text{and}{m}_2$ are suspended together by a massless spring of constant k. When the masses are in equilibrium, $m_1$ is removed without disturbing the system. Then the angular frequency of oscillation of $m_2$ is

Explanation

Let `l_(1)` and `l_(2)` be the extension produced by masses `m_(1)` and `m_(2)` respectively.
Then, `(m_(1) +m_(2))g=k(l_(1)+l_(2))…(i)`
After `m_(1)` is removed , `m_(2)g=kl_(2)`…(ii)
Eqs. (i) -(ii) gives `m_(1)g=kl_(1)` or `l_(1)=(m_(1)g)/(k)`
Now, angular frequency of `m_(2), omega=(2pi)/(T)=2pisqrt((k)/(m_(2)))`