A simple pendulum has time period T The bob is given negative charge and surface below it is given positive change new time period will be
less than T
greater than T
equal to T
infinite
The correct Answer is:A
To solve the problem of how the time period of a simple pendulum changes when the bob is given a negative charge and the surface below it is given a positive charge, we can follow these steps:
Step 1: Understand the Initial Time Period of the Pendulum
The time period T of a simple pendulum is given by the formula:
T=2π√Lg
where L is the length of the pendulum and g is the acceleration due to gravity.
Step 2: Analyze the Effect of Charges on the Pendulum
When the bob is given a negative charge −q and the surface below it is given a positive charge +Q, an electric field E is created. The force acting on the charged bob due to this electric field is:
Fe=qE
Since the bob has a negative charge, the direction of the force will be opposite to the direction of the electric field.
Step 3: Calculate the Effective Gravitational Acceleration
The effective gravitational acceleration geffective acting on the bob will be modified due to the electric force. The new effective gravitational force can be expressed as:
mgeffective=mg+qE
Thus, we can express the effective gravitational acceleration as:
geffective=g+qEm
where m is the mass of the bob.
Step 4: Substitute into the Time Period Formula
Now, substituting geffective into the time period formula, we get the new time period T′:
T′=2π√Lgeffective=2π
⎷Lg+qEm
Step 5: Compare the New Time Period with the Original Time Period
Since qEm is a positive quantity (as it represents the additional force acting on the bob), we can see that:
geffective>g
This implies that:
√Lgeffective<√Lg
Therefore, the new time period T′ will be:
T′<T
Conclusion
The new time period T′ of the pendulum after charging the bob and the surface will be less than the original time period T.
Final Answer
The new time period will be less than T.
---
The time period T of a simple pendulum is given by the formula:
T=2π√Lg
where L is the length of the pendulum and g is the acceleration due to gravity.
When the bob is given a negative charge −q and the surface below it is given a positive charge +Q, an electric field E is created. The force acting on the charged bob due to this electric field is:
Fe=qE
Since the bob has a negative charge, the direction of the force will be opposite to the direction of the electric field.
The effective gravitational acceleration geffective acting on the bob will be modified due to the electric force. The new effective gravitational force can be expressed as:
mgeffective=mg+qE
Thus, we can express the effective gravitational acceleration as:
geffective=g+qEm
where m is the mass of the bob.
Now, substituting geffective into the time period formula, we get the new time period T′:
T′=2π√Lgeffective=2π
⎷Lg+qEm
Since qEm is a positive quantity (as it represents the additional force acting on the bob), we can see that:
geffective>g
This implies that:
√Lgeffective<√Lg
Therefore, the new time period T′ will be:
T′<T
The new time period T′ of the pendulum after charging the bob and the surface will be less than the original time period T.
The new time period will be less than T.
Similar Questions
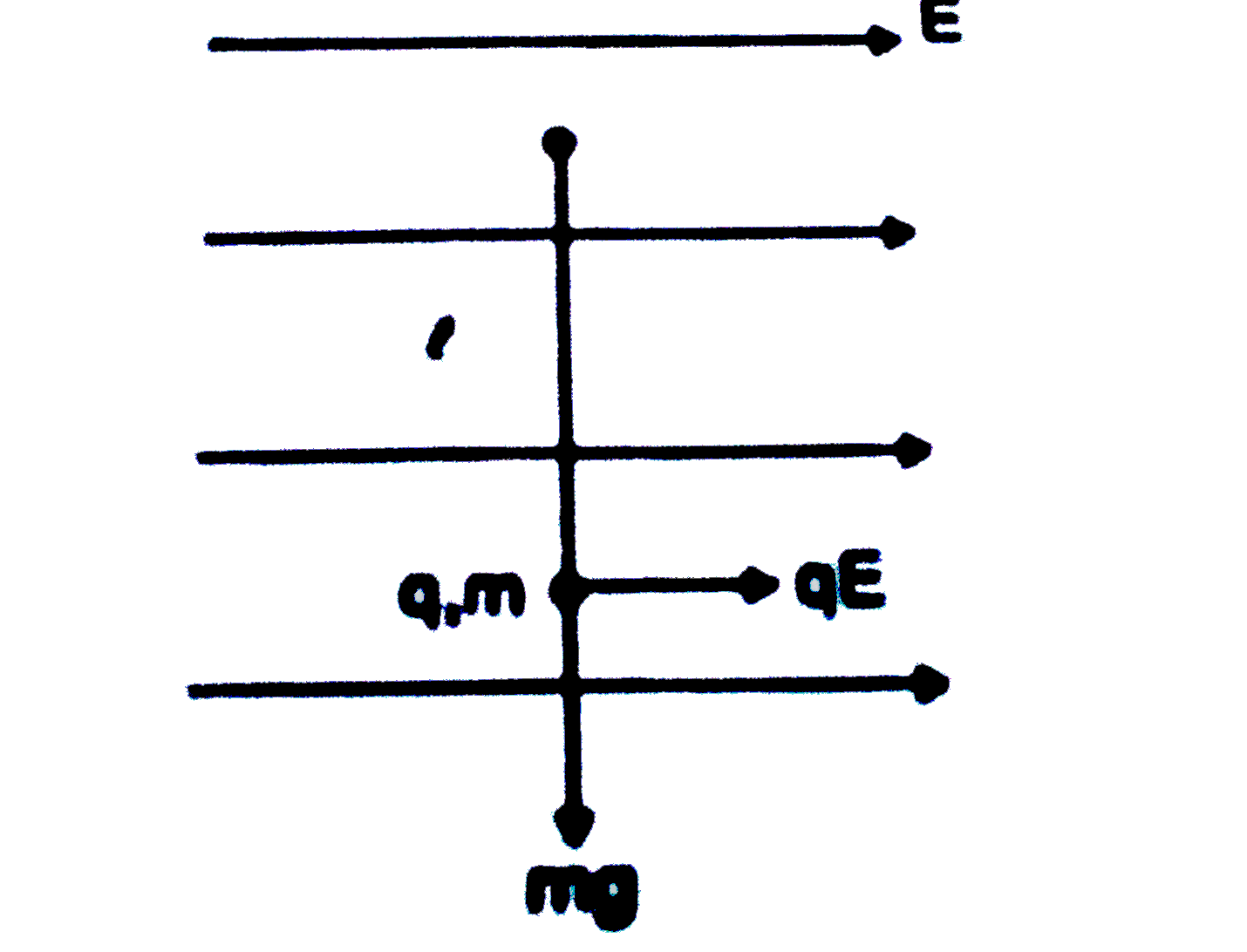
A simple pendulum has a length l & mass of bob m. The bob is given a charge q coulomb. The pendulum is suspended in a uniform horizontal electric field of strength E as shown in figure, then calculate the time period of oscillation when bob is slightly displaced from its mean position.
View SolutionIf length of simple pendulum is increased by 6% then percentage change in the time-period will be
View SolutionA simple pendulum with iron bob has a time period T. The bob is now immersed in a non-viscous liquid and oscillated, if the density of liquid is 112th that of iron, then new time period will be
View SolutionA simple pendulum has some time period T. What will be the percentage change in its time period if its amplitude is decreased by 5%
View SolutionA simple pendulum has time period T = 2s in air. If the whole arrangement is placed in a non viscous liquid whose density is 1/2 times the density of bob. The time period in the liquid will be
View SolutionIn case of a simple pendulum, time period versus length is depicted by
View SolutionA simple pendulum has a time period 2 s on the earth surface. If pendulum is taken to height R2 above the earth 's surface, (R is radius of the earth), the time period becomes
View SolutionThe simple pendulum shown here has time period T, when the cart is at rest. If cart moves with constant velocity 8ms−1 towards left, then the new time period will be
_PHY_E06_344_Q01.png) .
.
View SolutionA simple pendulum has a time period T1 when on the earth's surface and T2 when taken to a height R above the earth's surface, where R is the radius of the earth. The value of T2T1 is
View SolutionA spring-block system undergoes vertical oscillations above a large horizontal metal sheet with uniform positive charge. The time period of the oscillations is T. If the block is given a charge Q, its time period of oscillation will be
View Solution
