Given that the displacement of the body in meter is a function of time as follows
x=2t4+5
The mass of the body is 2 kg. What is the increase in its kinetic energy one second after the start of motion?
8 J
16 J
32 J
64 J
The correct Answer is:D
To solve the problem, we need to find the increase in kinetic energy of a body after one second of motion given its displacement function. Here’s a step-by-step solution:
Step 1: Identify the displacement function
The displacement x of the body is given by:
x=2t4+5
Step 2: Differentiate the displacement to find velocity
To find the velocity v, we differentiate the displacement x with respect to time t:
v=dxdt=ddt(2t4+5)
Using the power rule of differentiation:
v=8t3
Step 3: Calculate the velocity at t=1 second
Now, we substitute t=1 second into the velocity equation:
v(1)=8(1)3=8m/s
Step 4: Determine the initial velocity
Since the body starts from rest, the initial velocity u at t=0 seconds is:
u=0m/s
Step 5: Use the kinetic energy formula to find the increase in kinetic energy
The change in kinetic energy ΔK can be calculated using the formula:
ΔK=12mv2−12mu2
Substituting the known values:
- Mass m=2kg
- Final velocity v=8m/s
- Initial velocity u=0m/s
We have:
ΔK=12×2×(8)2−12×2×(0)2
Step 6: Simplify the equation
Calculating the terms:
ΔK=12×2×64−0
ΔK=1×64=64J
Final Answer
The increase in kinetic energy one second after the start of motion is:
ΔK=64J
---
The displacement x of the body is given by:
x=2t4+5
To find the velocity v, we differentiate the displacement x with respect to time t:
v=dxdt=ddt(2t4+5)
Using the power rule of differentiation:
v=8t3
Now, we substitute t=1 second into the velocity equation:
v(1)=8(1)3=8m/s
Since the body starts from rest, the initial velocity u at t=0 seconds is:
u=0m/s
The change in kinetic energy ΔK can be calculated using the formula:
ΔK=12mv2−12mu2
Substituting the known values:
- Mass m=2kg
- Final velocity v=8m/s
- Initial velocity u=0m/s
ΔK=12×2×(8)2−12×2×(0)2
Calculating the terms:
ΔK=12×2×64−0
ΔK=1×64=64J
The increase in kinetic energy one second after the start of motion is:
ΔK=64J
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRANCE SPECIAL QUESTIONS
16 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS
7 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.3
10 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
Knowledge Check
A body has kinetic energy 250 J. If the mass of the body is 5 kg, then choose its velocity and momentum from the following options.
A50 m/sB50 kg.m/sC20kg. m/sD15 m/sA body possesses kinetic energy of 'X'J. If the mass of body increases 49 times determine its final velocity if Kinetic energy remains constant.
A1/5th the original velocityB1/2 the original velocityC1/7th the original velocityD1/X the original velocity
The displacement x of a body of mass 1 kg on a horizontal smooth surface as a function of time t is given by x=t44. The work done in the first second is
View SolutionThe displacement of a body is given by s=12gt2 where g is acceleration due to gravity. The velocity of the body at any time t is
View SolutionIn the given figure F=10N,R=1m, mass of the body is 2kg and moment of inertia of the body about an axis passing through O and perpendicular to the plane of the body is 4kgm2. O is the centre of mass of the body.
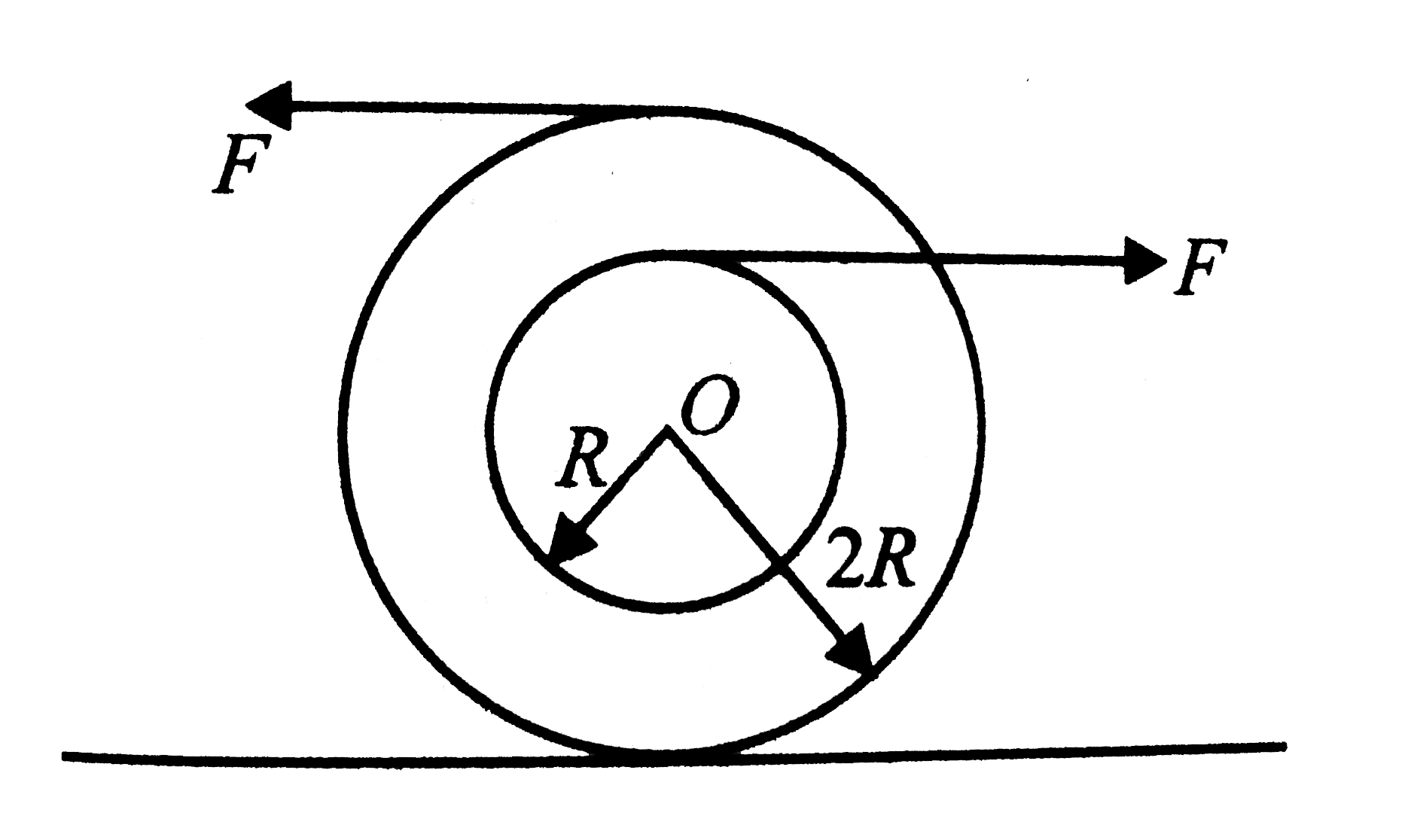
If the ground is smooth, what is the total kinetic energy of the body after 2s?
View SolutionA body of mass 10 kg is moving with a velocity of 20 m s−1 . If the mass of the body is doubled and its velocity is halved, find : (i) the initial kinetic energy, and (ii) the final kinetic energy
View SolutionDisplacement x ( in meters ) , of a body of mass 1 kg as a function of time t, on a horizontal smooth surface , is given as x=2t2 Then work done in the first one second by the external force is
View SolutionUnder the action of a force a 2 kg body moves such that its position x in meters as a function of time t is given by x=t44+3. Then work done by the force in first two seconds is
View Solution
