A spherical ball of mass 20kg is stationary at the top of a hill of height 100m , it rolls down a smooth surface to the ground , then climbs up another bill of height of 30m and final rolls down to a horizontal base at a height of 20m about the ground . The velocity attained by the ball is
To solve the problem of the spherical ball rolling down the hill and finding the velocity attained by the ball, we can use the principle of conservation of energy. Here’s the step-by-step solution:
Step 1: Identify the initial and final heights
- The ball starts at a height of 100 m and ends at a height of 20 m after rolling down the hill and then rolling to the horizontal base.
- The change in height (h) is calculated as:
h=Initial Height−Final Height=100m−20m=80m
Step 2: Apply the conservation of energy principle
- According to the conservation of energy, the potential energy (PE) at the top will be converted into kinetic energy (KE) at the bottom.
- The potential energy at the height of 100 m is given by:
PE=mgh
where:
- m=20kg (mass of the ball)
- g=9.81m/s2 (acceleration due to gravity)
- h=80m (change in height)
Step 3: Calculate the potential energy at the top
- Substituting the values:
PE=20kg×9.81m/s2×80m
PE=20×9.81×80=15696J
Step 4: Set potential energy equal to kinetic energy
- At the bottom, all potential energy is converted into kinetic energy:
KE=12mv2
Setting PE=KE:
15696=12×20×v2
Step 5: Solve for velocity (v)
- Rearranging the equation to solve for v2:
15696=10v2
v2=1569610=1569.6
v=√1569.6≈39.6m/s
Step 6: Final result
- The velocity attained by the ball is approximately:
v≈40m/s
- The ball starts at a height of 100 m and ends at a height of 20 m after rolling down the hill and then rolling to the horizontal base.
- The change in height (h) is calculated as:
h=Initial Height−Final Height=100m−20m=80m
- According to the conservation of energy, the potential energy (PE) at the top will be converted into kinetic energy (KE) at the bottom.
- The potential energy at the height of 100 m is given by:
PE=mgh
where:
- m=20kg (mass of the ball)
- g=9.81m/s2 (acceleration due to gravity)
- h=80m (change in height)
- Substituting the values:
PE=20kg×9.81m/s2×80m
PE=20×9.81×80=15696J
- At the bottom, all potential energy is converted into kinetic energy:
KE=12mv2
Setting PE=KE:
15696=12×20×v2
- Rearranging the equation to solve for v2:
15696=10v2
v2=1569610=1569.6
v=√1569.6≈39.6m/s
- The velocity attained by the ball is approximately:
v≈40m/s
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.1
20 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.2
20 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
A ball is thrown vertically upward with a velocity of 20 m/s. Calculate the maximum height attain by the ball.
View SolutionA ring starts to roll down the inclined plane of height h without slipping . The velocity when it reaches the ground is
View SolutionA ball of mass 2 kg is projected horizontally with a velocity 20m/s from a building of height 15m. The speed with which body hits the ground is :
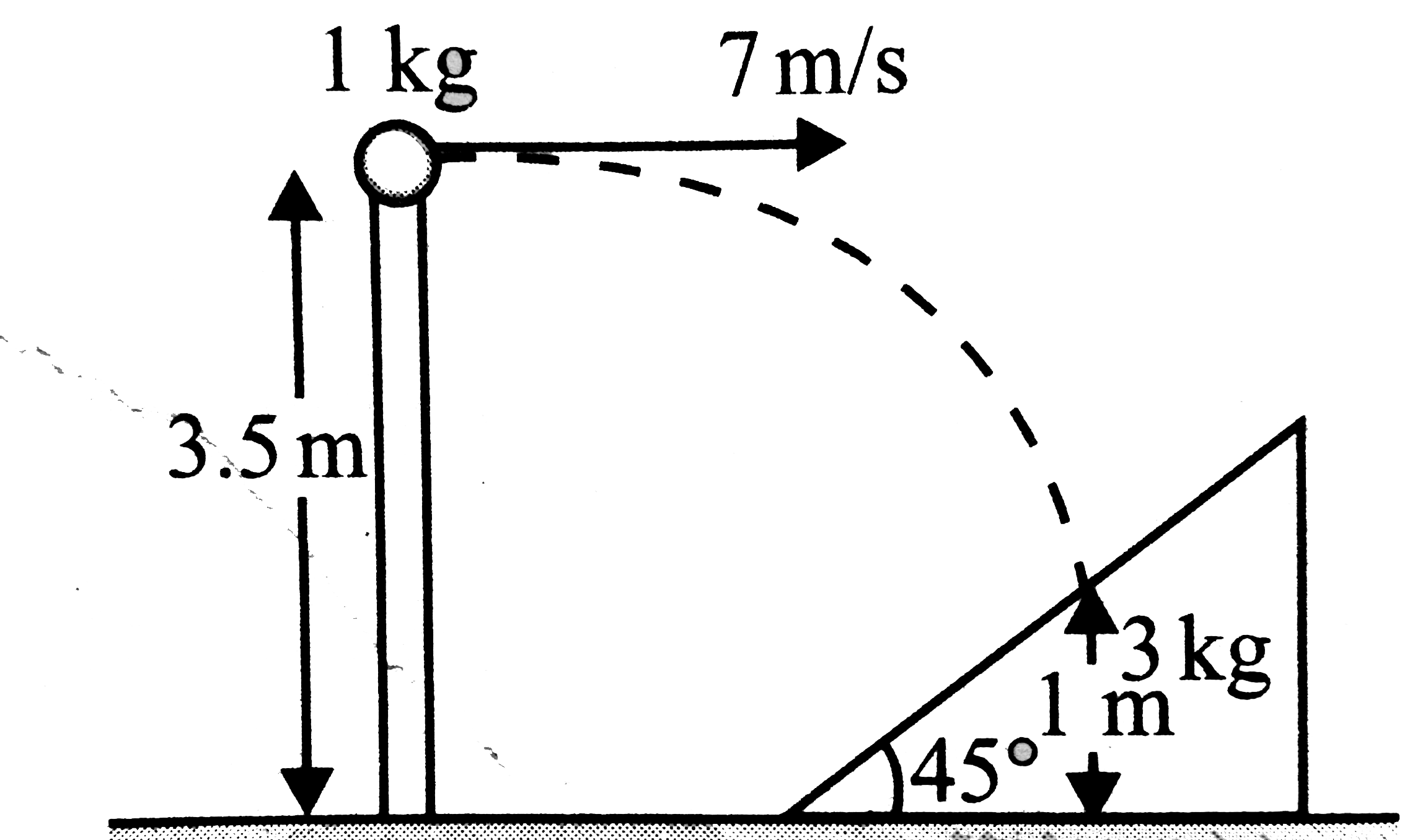
View SolutionA smooth ball of mass 1kg is projected with velocity 7m/s horizontal from a tower of height 3.5m. It collides elastically with a wedge of mass 3 kg and inclination of 45∘ kept on ground. The ball collides with the wedge at a height of 1m above the ground. Find the velocity of the wedge and the ball after collision. (Neglect friction at any contact.)
View SolutionA ball dropped from a height of 2 m rebounds to a height of 1.5 m after hitting the ground. Then fraction of energy lost is
View SolutionA solid sphere rolls on a smooth horizontal surface at 10m/s and then rolls up a smooth inclined plane of inclination 30∘ with horizontal. The mass of the sphere is 2kg. Find the height attained by the sphere before it stops (in m).
View SolutionA ball of mass m is thrown straight up. It goes to a maximum height and then returns. Finally it strikes ground. In the whole process.
View SolutionFrom the top of a tower of height 200m, a ball A is projected up with 10ms−1. And 2s later another ball B is projected verticall down with the same speed. Then .
View SolutionA steel ball is dropped on a hard surface from a height of 1m and rebounds to a height of 64cm. The maximum height attained by the ball after nth bounce is (in m)
View SolutionA ball is dropped from the top of a tower of height 78.4 m Another ball is thrown down with a certain velocity 2 sec later. If both the balls reach the ground simultaneously, the velocity of the second ball is
View Solution
